filmov
tv
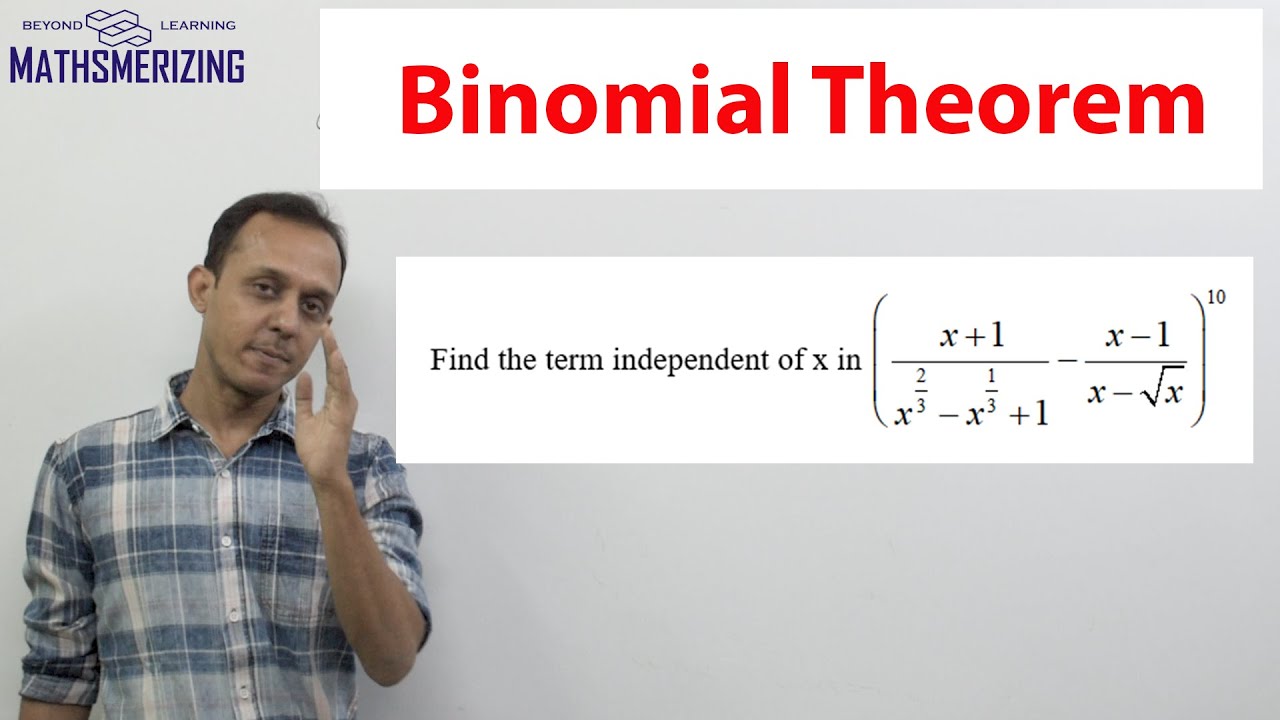
Binomial theorem: General term SE6: Term independent of x {(x+1)(x^2/3-x^1/3+1)-(x-1)(x-x^1/2)}^10
Показать описание
Binomial theorem: General term SE6: Term independent of x {(x+1)(x^2/3-x^1/3+1)-(x-1)(x-x^1/2)}^10 Support the channel:
0:03:34
Binomial theorem: General term SE6: Term independent of x {(x+1)(x^2/3-x^1/3+1)-(x-1)(x-x^1/2)}^10
0:05:04
Binomial theorem: General term & Basic observations
0:13:59
Binomial Theorem (I+F) Concept | Integral Part | JEE Mains + Advanced by Harpreet Singh (HSM Sir)
0:02:36
Binomial theorem SE6: Coefficient of x^5 in (1+x+x^3)^9 : Binomial method
0:05:13
Binomial Expansion | Term Independent of x | AS level Mathematics | CIE
0:02:58
Binomial Theorem - Finding Coefficient of Binomial Expansion
0:17:52
Binomial expansion | Find the Coefficient of x^n
1:17:09
Binomial Theorem Class 11 Maths - Binomial Expansion | Keshav Sir | JEE Mains & Advanced 2023
0:02:55
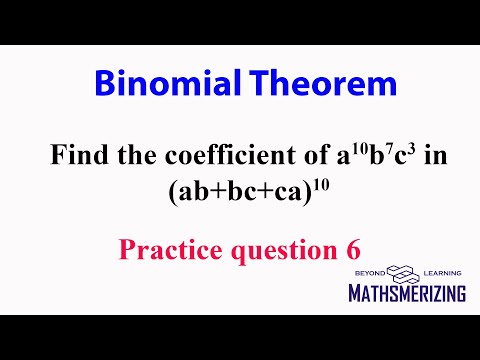
Binomial theorem: PQ6: Coefficient of a^10b^7C^3 in (ab+bc+ca)*10
0:03:36
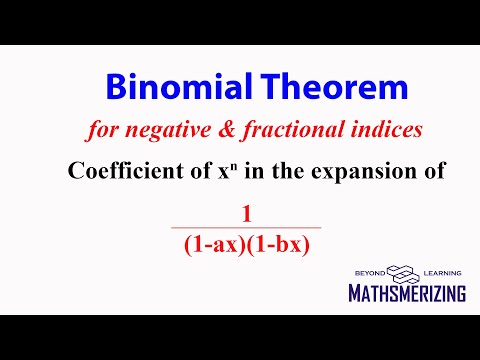
BT: Negative & fractional indices: SE6 Coefficient of x^n in 1/(1-ax)(1-bx)
0:03:58
Binomial theorem SE11: Find the coefficient of x^7 in (1-x-x^2+x^3)^6 Binomial method
0:03:19
Binomial theorem: PQ2: Coefficient of x^r in (x+a)^n-1+(x+a)^n-2(x+b)+...+(x+b)^n-1
0:05:25
Binomial series: Lecture 1: Prerequisites, basic series & summation forms with examples
0:17:32
Lecture 9: Divisibility results based on the binomial theorem
0:05:47
Binomial series: Lecture 5: Sum of all the coefficients in the expansion
0:04:24
find the coefficient of the middle term in the expansion of (1+4x+4x²)⁵ | binomial theorem
0:01:56
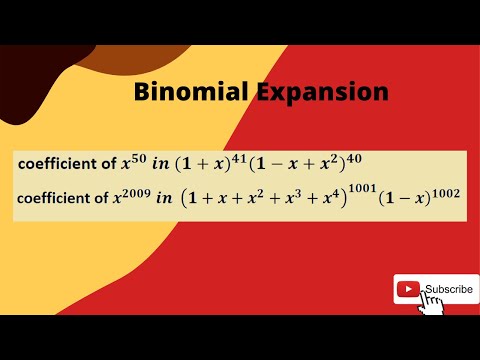
The coefficient of x⁴ in the expansion of (1+x+x²+x³)⁶ in the lowest of x is
0:05:08
Binomial Expansion_20
0:04:10
The term independent of x in expansion of ((x+1)/(x^(2/3)-x^(1/3)+1)-(x-1)/(x-x^(1/2) ))^10 is
0:09:30
The term independent of x in expansion of |IIT JEE Mains-2013| Mathematics
0:05:02
Find the coefficient of x^15 in the expansion (x+x^2)^15.Binomial Theorem Question NCERT.
0:13:18
#Shortcuts of Binomial Theorem
0:02:51
Binomial theorem SE8: Coefficient of x^n in (1+x)(1-x)^n
0:05:09
If the ratio of coefficients of the three consecutives terms in binomial
Комментарии