filmov
tv
Binomial theorem: Comparison problems SE2: Show that (101)^50 greater than (100)^50+(99)^50
Показать описание
Binomial theorem: Comparison problems SE2: Show that (101)^50 greater than (100)^50+(99)^50 Support the channel:
0:02:15
Binomial theorem: Comparison problems SE2: Show that (101)^50 greater than (100)^50+(99)^50
0:01:27
Binomial theorem: Comparison problems: Which is greater ? (1.01)^1000000 or 10000
0:04:31
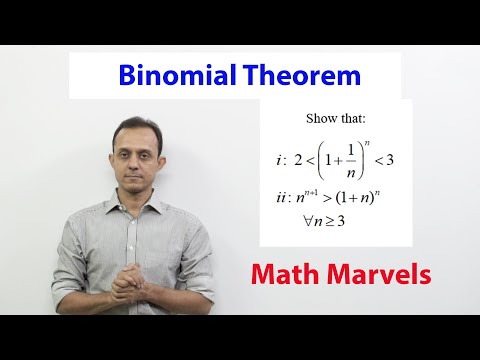
Binomial theorem: Comparison problems SE3: Show that (1+1/n)^n lies between 2 and 3
0:00:55
Binomial Theorem Problem
0:04:47
Binomial Theorem | Which Number is Larger
0:06:58
Compare numbers with large exponents using Binomial expansion: 4^29 and 5^25
0:00:59
Trick for End Term in Expansion | Binomial Expansion from End #shorts #viralshorts #youtubeshorts
0:11:02
Binomial Expansion Example: How to compute binomial coefficient
0:03:41
Greatest Integer Function: Binomial Theorem Solved example 2 If R=(5(3)^1/2+(8)^2n+1 find R.(R-[R])
0:06:36
Show that NF=20^(2n+1), if N = [6*sqrt(6) + 14]^(2n+1) and F is its fractional part.
0:16:10
Binomial series: Lecture 8: Algebraic technique simplifying summation
0:10:19
Which of the following is larger 99^50 + 100^50 or 101^50
0:02:03
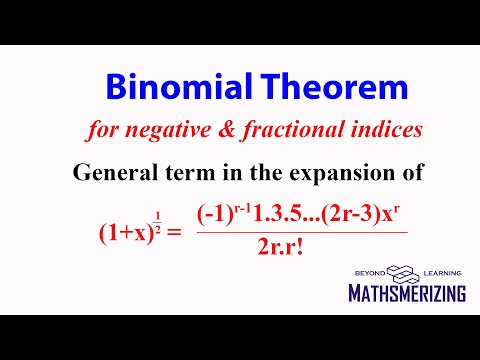
BT: Negative & fractional indices: SE2 General term in the expansion of (1+x)^1/2
0:07:45
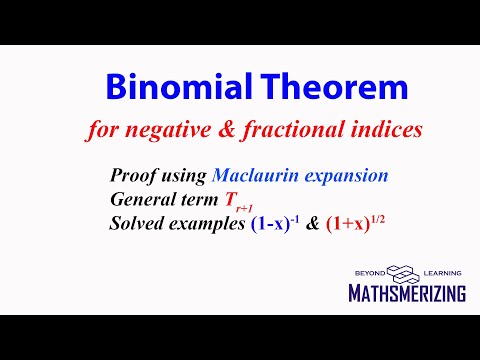
BT: Negative & fractional indices: Proof, general term with solved examples
0:00:16
binomial series #formulas,# shorts
0:00:16
When 99 is greater than 100. #genius #inteligent comment your answer just in 30sec.
0:05:04
Binomial theorem: General term & Basic observations
0:03:29
Binomial theorem SE16: Find sum of the roots of the equation x^2001+(1/2-x)^2001=0
0:04:29
Multiple Summation: Dependent variables SE 2: Find double summation nCi.nCj
0:08:54
Binomial Theorem 05 | Number of Rational Terms | Class 11th | Aman Sir Maths | Bhannat Maths
0:05:47
Binomial series: Lecture 5: Sum of all the coefficients in the expansion
0:16:16
Integral and Fractional Part of Binomial Expansion | Comparing Two Numbers using Binomial Expansion
0:00:16
@mrsir-physics first time in janakpuri Delhi 🖤
0:03:14
Using binomial theorem determine which number is smaller \( (1.2)^{4000} \) or 800 ?
Комментарии