filmov
tv
Limits and Continuity - AP Calculus Unit 1 Review

Показать описание
Thank you for watching my video! Please consider subscribing and sharing my content!
Timestamps
0:00 Intro
0:45 Intro to Continuity
6:19 Naming Discontinuities
8:47 Definition of Continuity
11:44 Intuition for Limits
16:38 Limits from the Left & Right
19:20 Limits and Continuity
AP Calculus AB Unit 1 Review | Limits and Continuity
Limits and Continuity
AP Calculus AB and BC Unit 1 Review [Limits and Continuity]
Strategy in finding limits | Limits and continuity | AP Calculus AB | Khan Academy
Continuity at a point | Limits and continuity | AP Calculus AB | Khan Academy
Calculus 1 - Introduction to Limits
AP Calculus BC Unit 1 Review: Limits and Continuity!
Limits from graphs | Limits and continuity | AP Calculus AB | Khan Academy
Continuity Basic Introduction, Point, Infinite, & Jump Discontinuity, Removable & Nonremovab...
Types of discontinuities | Limits and continuity | AP Calculus AB | Khan Academy
Introduction to limits | Limits | Differential Calculus | Khan Academy
Two Trig Limits YOU NEED for AP Calc Exam
Unbounded limits | Limits and continuity | AP Calculus AB | Khan Academy
Limit properties | Limits and continuity | AP Calculus AB | Khan Academy
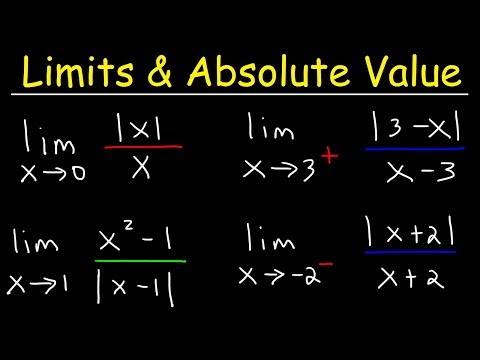
Limits and Absolute Value
3 Step Continuity Test, Discontinuity, Piecewise Functions & Limits | Calculus
Limits of piecewise functions | Limits and continuity | AP Calculus AB | Khan Academy
Limits of trigonometric functions | Limits and continuity | AP Calculus AB | Khan Academy
How To Evaluate Limits From a Graph
Limits (for dummies)
Functions continuous at specific x-values | Limits and continuity | AP Calculus AB | Khan Academy
Limits to define continuity
Infinite Limit Shortcut!! (Calculus)
Continuity over an interval | Limits and continuity | AP Calculus AB | Khan Academy
Комментарии
 0:07:08
0:07:08
 0:19:19
0:19:19
 1:08:28
1:08:28
 0:12:30
0:12:30
 0:08:16
0:08:16
 0:20:20
0:20:20
 0:29:19
0:29:19
 0:06:01
0:06:01
 0:13:31
0:13:31
 0:07:16
0:07:16
 0:11:32
0:11:32
 0:00:58
0:00:58
 0:02:32
0:02:32
 0:05:08
0:05:08
 0:17:44
0:17:44
 0:10:10
0:10:10
 0:03:49
0:03:49
 0:06:06
0:06:06
 0:11:32
0:11:32
 0:08:14
0:08:14
 0:04:21
0:04:21
 0:11:14
0:11:14
 0:00:51
0:00:51
 0:09:00
0:09:00