Differentials and linearization in Multivariable Calculus

In this lesson, we explore differentials (e.g. "dz") in multivariable calculus and their applications in error estimation. Differentials in multivariable calculus are tools for estimating errors and linearizing functions. They provide simpler ways to approximate changes in function values and are particularly useful when dealing with complex functions where direct computation might be challenging.

Multivariable Calculus Unit 3 Lecture 11

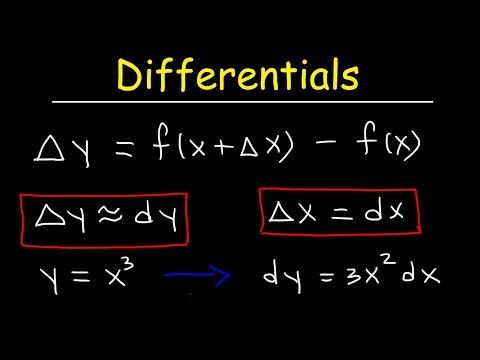
We begin by examining the concept of differentials for scalar-valued functions of multiple variables. In single-variable calculus, for a function 𝑦=𝑓(𝑥), the differential is defined as 𝑑𝑦=𝑓′(𝑥)𝑑𝑥. This differential 𝑑𝑦 approximates the true change Δ𝑦 in response to a small change in 𝑥.

In multivariable calculus, for a function 𝑧=𝑓(𝑥,𝑦), the differential 𝑑𝑧 is given by:

𝑑𝑧=∂𝑓/∂𝑥 𝑑𝑥 + ∂𝑓/∂𝑦 𝑑𝑦,

which can also be represented as a dot product of ∇𝑓 with the differential vector ⟨𝑑𝑥,𝑑𝑦⟩.

For example, given a cylinder with a height of 8 cm and radius of 3 cm, with a possible measurement error of up to 0.2 cm, we use differentials to estimate the maximum error in the volume. The volume function 𝑉(𝑟,ℎ)=𝜋𝑟^2ℎ has the gradient:

∇𝑉=(2𝜋𝑟ℎ,𝜋𝑟2).

Evaluating the gradient at (3,8) and dotting it with the error vector (0.2,0.2), we get:

𝑑𝑉=∇𝑉(3,8)⋅(0.2,0.2)=(2𝜋⋅3⋅8,𝜋⋅32)⋅(0.2,0.2)=(48𝜋,9𝜋)⋅(0.2,0.2)≈35.81 cm^3.

This value approximates the change in volume Δ𝑉: Δ𝑉≈𝑑𝑉.

Linearization is just the tangent plane approximation of a function near a known point in its domain (to estimate the value a function takes near a point in its domain). We do an example in the video.

#mathematics #multivariablecalculus #tangent #differentialcalculus #iitjammathematics #calculus3