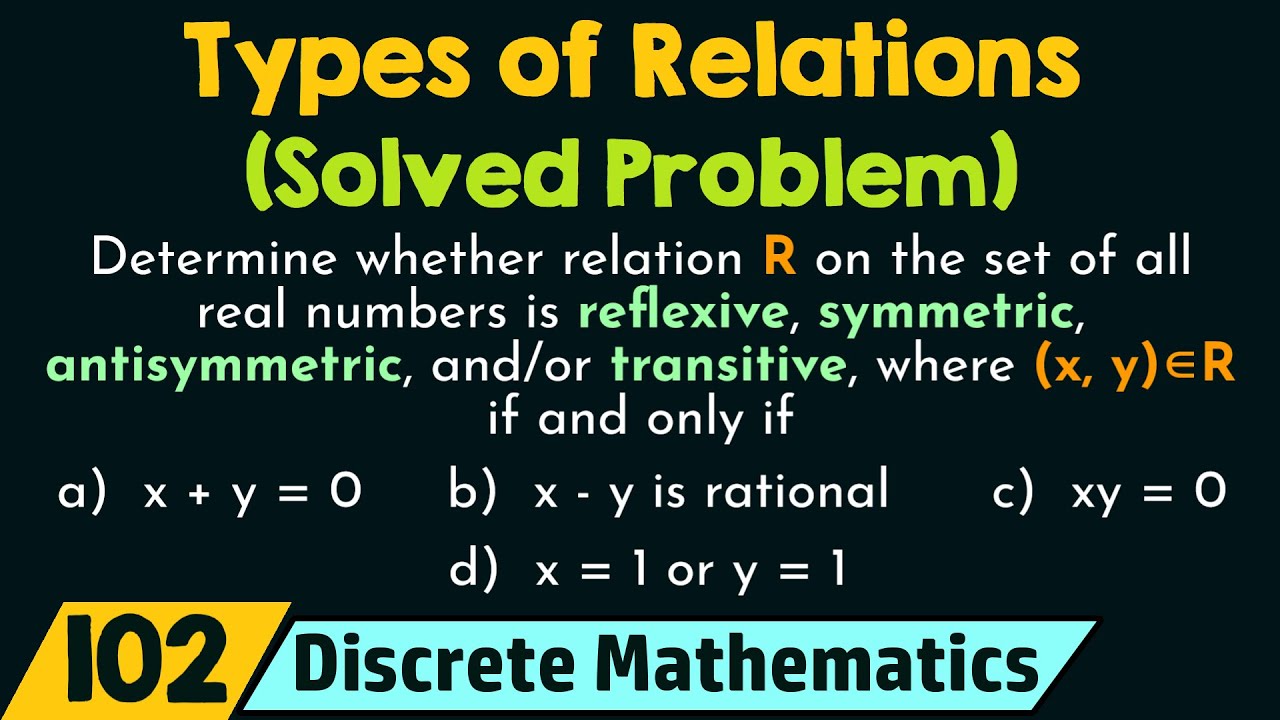
Types of Relations (Solved Problem)

Discrete Mathematics: Types of Relations (Solved Problem)
Topics discussed:
1) Solved problem on relation types.

Music:
Axol x Alex Skrindo - You [NCS Release]

#DiscreteMathematicsByNeso #DiscreteMaths #Relations #TypesOfRelations

[aditianand]

I promise to contribute the day I will get a job because right now my condition is not so good. This channel is the best I have ever found. Thank you so much neso academy

[ir]

Homework Answer:
NOT Transitive
For example,
(2, 1) and (1, 3) belongs to R, but (2, 3) does not belong to R

[deeppandit]

Hello Neso Academy,
First of all thank you so much you guys for these awesome videos. Your content really helps me. But at some point, it feels like videos are not coming much frequently. No offense, I know it's not easy to make these videos and you guys make videos on many different topic too, and it's so much time consuming. But you guys started Discrete Mathematics series 3 years ago and it's still not half a way. I know it's a very huge area, it's going to take time, but it's just a genuine request from your student (your fan), that could you guys please upload videos much frequently. You guys said anyone in college who have this subject in syllabus or anyone preparing for GATE could watch it, but since the series is not fully completed, we can't understand the whole subject. Kitno ki to exam nikal jayegi jab tak yeh series khatam hogi. Just a genuine appeal, btw I love you guys and your content. ❤️

[Enthub]

The last relation {x, y| x=1 or y=1 } is NOT TRANSITIVE because
When we have a set A={1, 2, 3}
(1, 2), (2, 3), (3, 1) in this not all the all the pairs include one hence there’ll be a case where y is not = 1 or x is not =1 ((2, 3)).

[usamaahmed]

the answer is :not transitive see why
if a=1 the answer will be transitive
(a, b)=(1, 2) and (b, c)=(2, 3) then (a, c)=(1, 3)
but if b=2 the answer will be not transitive : (a, b)=(2, 1) and (b, c)=(1, 3) then (a, c)=(2, 3) and it isn’t satisfied the condition of a=1 or b=1 . i hope it’s clear

[corinaalexeynov]

H/W Answer ;
Relation is not transitive since (a, 1) belongs to R and (1, b) belongs to R but (a, b) does not belong to R

[rajeshprajapati]

Answer to H.W : R is Not Transitive. For Ex - a=2, b=1, c=2.

[chibuikemchigbo]

it is transitive. considering a=1, b=1 and c=2, then result will still be 1, 2. x=1 or y=1 doesn't mean that both can not be equal to 1

[Joonie]

Hi, great video. Please cover equivalence relation and equivalence classes in the next episode.

[jackshih]

In my class, we just end number theory...
Maybe I will review discrete using your videos lol.

ps. most i love is your c programming and data structure

[MrGustavier]

Isn't relation b) an equivalence relation on the real numbers ?

[ramanrajagopalan]

Hi @4:01 why did you take x and y as 0 for reflexive and symmetric and why did you take 1 and -1 for antisymmetric and transitive? If we take same 0 for antisymmetric and transitive they will be accepted right?

[acriziosouza]

Homework Answer: Not Transitive.

For being transitive, it's not sufficient that X or Y be 1, the union of ordered pairs (a, b) and (b, c) must implies in the ordered pair (a, c). However, it's possible to not reach this answer. For example:

a = 3
b = 1
c = 2

(a, b) ^ (b, c) ^ (a, c)
(3, 1) ^ (1, 2) ^ (3, 2)

Although "b" fits the criteria (Y = 1), the ordered pair (a, c) isn't a implication of the union of the ordered pairs (a, b) and (b, c).

Of course if a=1, b=2 and c=1, the implication of the union of the same ordered pair would fit the criteria. However, it isn't the only possibility.

Therefore, "X=1 or Y=1" is not a Transitive relation.

[mohammadtarig]

My answer to homework is not transitive. Why? ⬇️


For it to be transitive: (a, b) belongs to R AND (b, c) belongs to R implies (a, c) belongs to R and that is not true.
For example: (0, 1) belongs to R AND (1, 3) belongs to R but (0, 3) doesn't belong to R.