Find Exact Value of Cos(2x) & Sin(2x) Given Sin(x)=-Sqrt(3)/5, Cos(x) is + (Double Angle Identities)

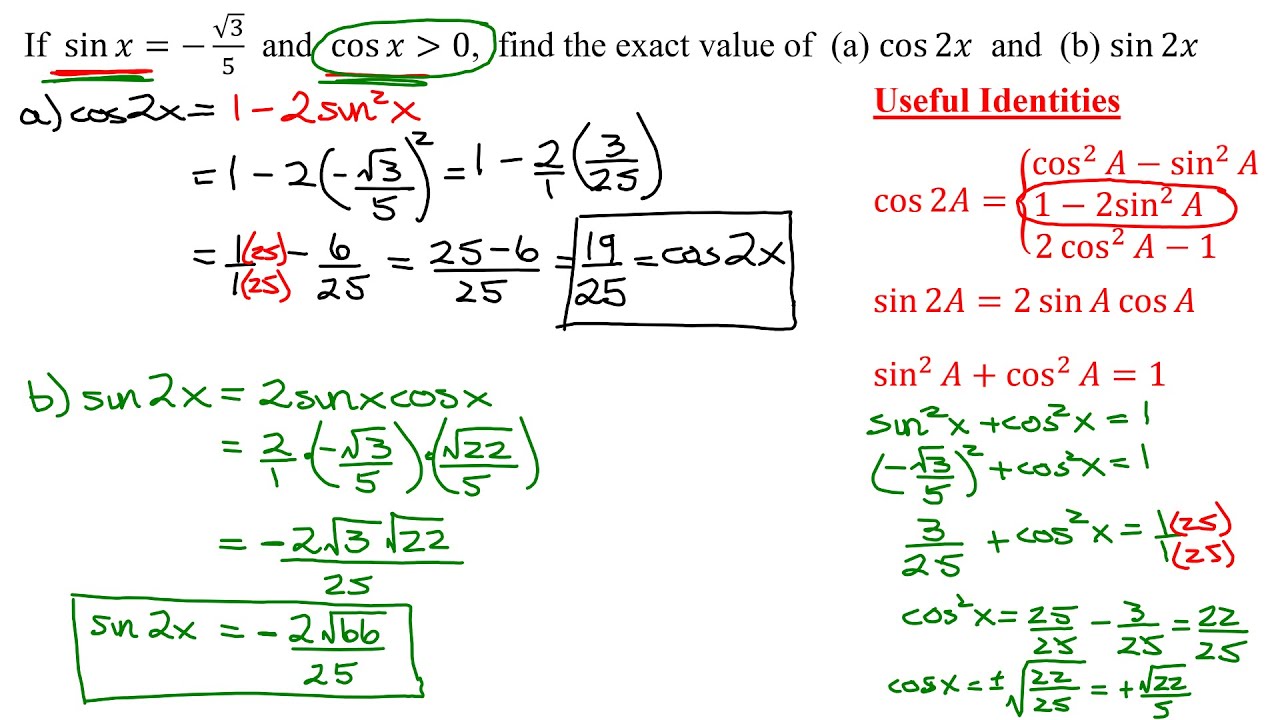
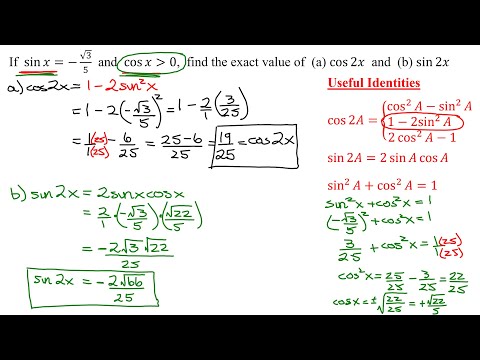
Find cos(2x) and sin(2x), given sin(x)=-sqrt(3)/5 and cos(x) is greater than zero. (a) As there are three version for the identity for cos(2x), the version chosen is the one that only contains the given information of sin(x). The value for sin(x) is substituted into cos(2x)=1-2sin^2(x) and the answer is simplified. (b) As sin(2x)=2sin(x)cos(x), the value of cos(x) needed to be found and the method chosen used the Pythagorean Identity sin^2(x)+cos^2(x)=1. It was given that the cos(x) was greater than zero so the positive square root was used when finding cos(x). Finally the values for sin(x) and cos(x) were substituted into the identity for the sin(2x).

Timestamps
0:00 Introduction
0:52 Double Angle Identity for cos(2A)
1:38 Find cos(2x)
3:46 Double Angle Identity for sin(2A)
4:07 Find cos(x)
6:36 Find sin(2x)

[bryant]

What accent is that? Very interesting to here you talk.