filmov
tv
Exponential integration by parts, integral x^3e^-x^2, choose u and dv and apply integration by parts

Показать описание
Questions or requests? Post your comments below, and I will respond within 24 hours.
In this exponential integration by parts example, we do the integral x^3e^-x^2 by splitting off a factor of x to set up the chain rule backwards for dv in the integration by parts formula when we choose u and dv. In integration by parts, choose u=x^2 so it will get simpler when we differentiate, and the antiderivative of dv is easy to guess. We choose dv=xe^-x^2, because this allows us to use the chain rule backwards to find v. After we apply integration by parts, we're left with another integral whose antiderivative is guessable, and we find our final result of integration by parts.
 0:02:57
0:02:57
 0:05:43
0:05:43
 0:11:16
0:11:16
 0:00:33
0:00:33
 0:32:52
0:32:52
 0:08:11
0:08:11
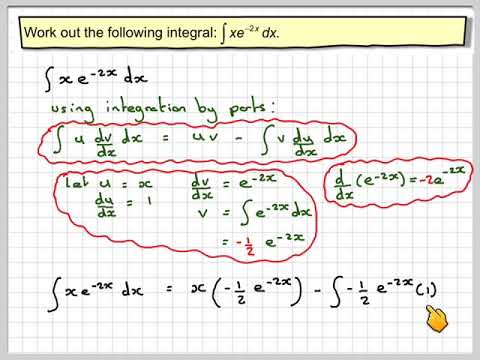 0:03:27
0:03:27
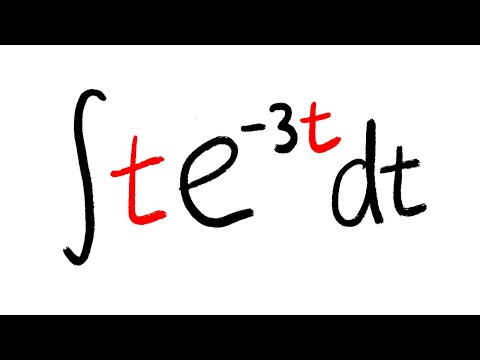 0:04:58
0:04:58
 0:03:57
0:03:57
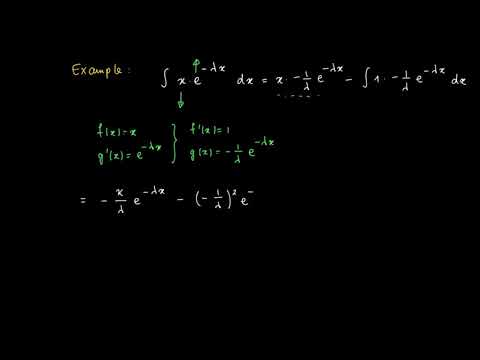 0:02:58
0:02:58
 0:00:13
0:00:13
 0:16:32
0:16:32
 0:01:55
0:01:55
 0:09:00
0:09:00
 0:00:11
0:00:11
 0:04:27
0:04:27
 0:01:22
0:01:22
 0:10:40
0:10:40
 0:06:34
0:06:34
 0:01:04
0:01:04
 0:00:15
0:00:15
 0:00:16
0:00:16
 0:00:45
0:00:45
 0:09:27
0:09:27