Algebraic structure on the Euclidean projective line | Rational Geometry Math Foundations 137

In this video we look at some pleasant consequences of imposing a Euclidean structure on the projective line. We give a proof of the fundamental projective Triple quad formula, talk about the equal p-quadrances theorem, and see how the logistic map of chaos theory makes its appearance as the second Spread polynomial.

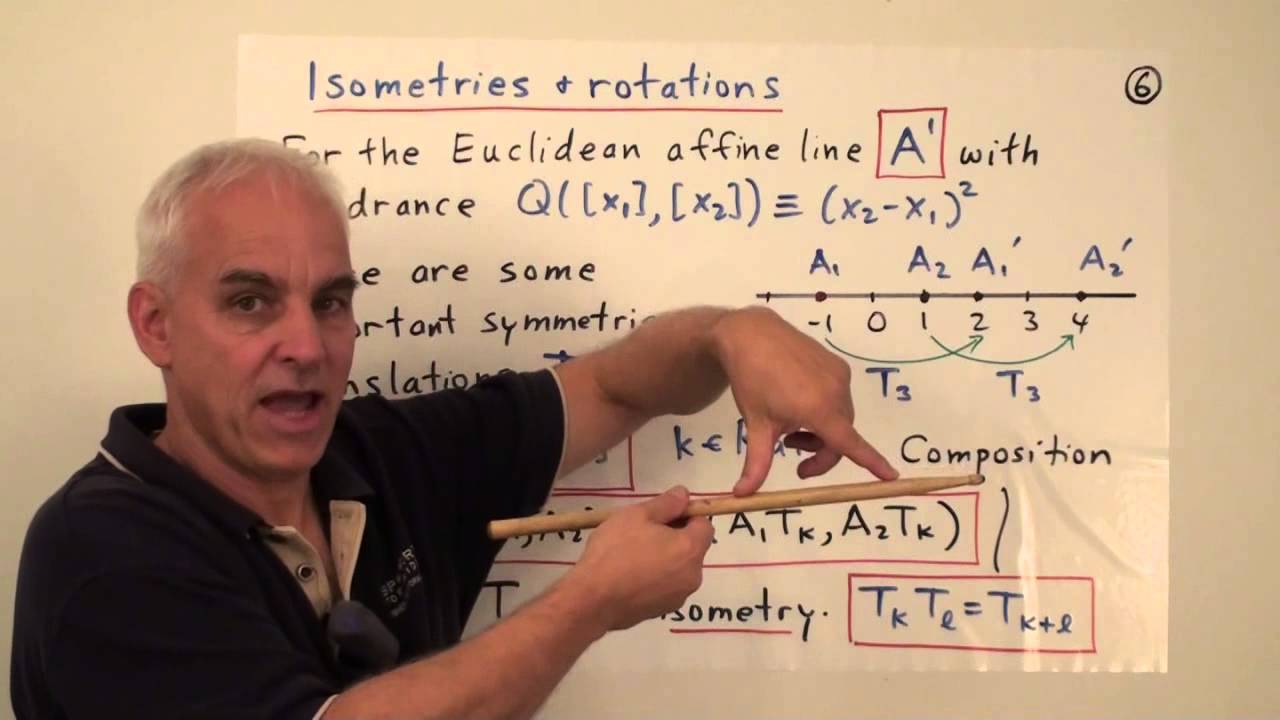
We also introduce rotations and reflections in this one-dimensional situation, and see how the composition of rotations naturally leads to an algebraic structure on the projective line. This multiplication is a one-dimensional version of complex number multiplication.

Video Content:
00:00 Introduction
4:22 The projective TQF (Triple Spread Formula)
9:34 How to establish the P-TQF
15:22 Equal projective- quadrance theorem
18:06 The logistic map
20:32 Isometries and rotations
25:03 Euclidean projective line
30:36 Rotation isometry theorem
35:38 Composition of Rotations

************************

Here are the Insights into Mathematics Playlists:

[Igdrazil]

Rotation, and especialy this purely algebraic définition of rotation allows to define ANGLES purely algebraicaly... ie simply AS A ROTATION! An ANGLE can perfectly be difined as such a ROTATION
And it is all the more a very satisfactory way of difining it. Even in an essential "relativistic" way, because what's really the idea of an "angle" between two lines is in fact the very WHAT makes that these two lines are not equal; different. It is thus a mesure of "relative distinct personality, individuality, uniqueness". But then the key point, as is emphasised in particular in relativistic theories, is crucialy : HOW DO WE KNOW HOW "DIFFERENT" ARE TWO LINES (or projective points)?
Well the only concrete way to evaluate that, exept maybe for The Omniscient, is to take or start at one line, and "ROTATE IT" until it matches with the other one.
And at the end of this "journey", from one line to the other, as far as we have an intrinsic way to keep this "journey" ISOMETRIC (preserving the projective quadrance : q[uR:vR]=q[u:v]), then the fundamental EVALUATION of the DIFFERENCE between those two lines, is in fact THIS ROTATION, that can also be called ANGLE.
Finaly what evaluates the "cost of gazoline" of this "journey" is ONE reasonable and usefull MESURE OF THIS ANGLE-ROTATION. And that cost is QUANTITATIVELY EVALUATED for instance by the euclidian projective quadrance : q[u:uR], (where u is a projective point (vector) of the first line and uR a projective point (vector) of the second line.)
Rotations are thus a kind of "matching agency" for projective points (lines) society