filmov
tv
Algebra 2 - Simplifying square roots with imaginary numbers, x^2 + 1 = 0
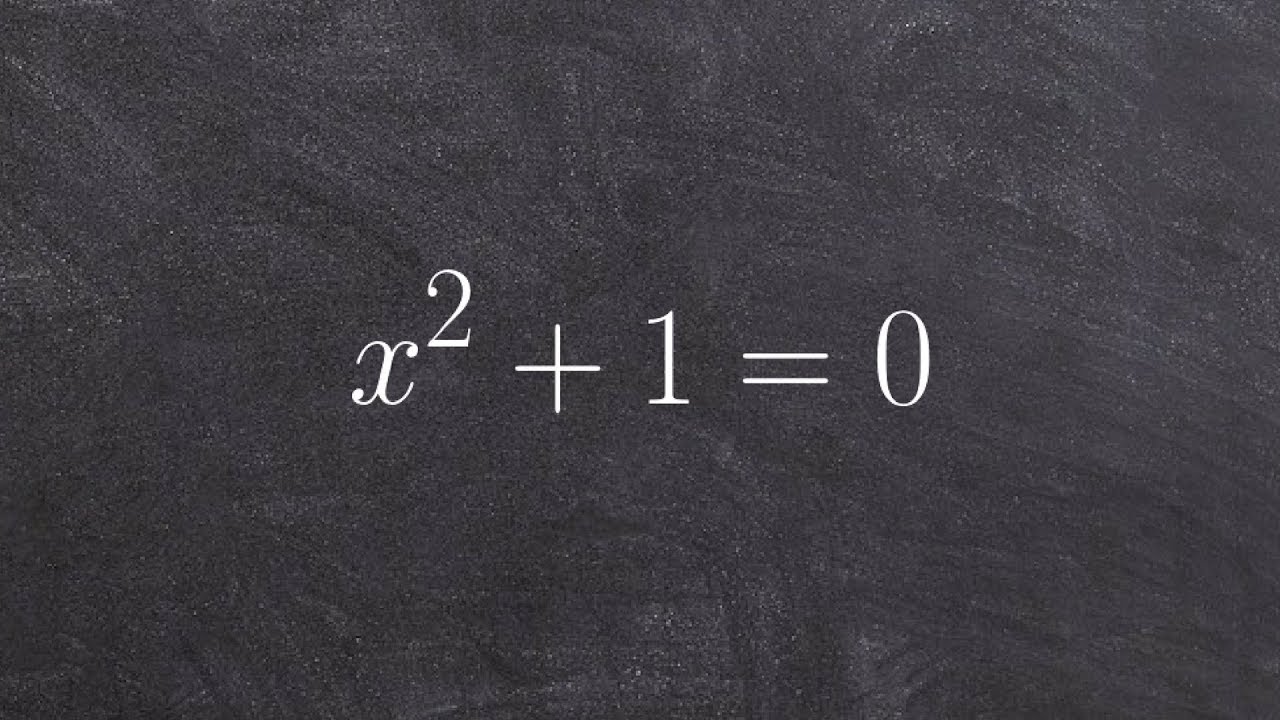
Показать описание
x^2 + 1 = 0
Simplify a radical expression with variables
Algebra 2 - How to simplify the a radical with imaginary numbers, root(-45)
Simplifying Radicals Easy Method
Simplifying Radicals with Variables and Exponents
Algebra 2 - Learn how to simplify a radical with imaginary numbers, 3sqrt(-128)
Algebra 2 - Simplifying square roots with imaginary numbers, x^2 + 1 = 0
Simplifying Radical Expressions 2
Simplifying the square root of a square number and exponent
How to Solve Quadratic Equations by Factoring, Completing the Square and using the Quadratic Formula
Math Antic - Simplifying Square Roots
How To Simplify Radicals
Algebra 2 - Simplifying negative radicals using the the imaginary unit i, sqrt(-24)
Algebra 2 - Simplifying square roots to prepare for imaginary numbers, root(9), root(20)
Simplifying square roots | Exponents, radicals, and scientific notation | Pre-Algebra | Khan Academy
How To Simplify Square Roots
01 - Simplify Square Roots with Factor Trees in Algebra (Radical Expressions), Part 1
Simplifying radicals simplify a square root expression
Algebra 2 - Learn to simplify a radical using the imaginary unit i, sqrt(-25)
Math - Simplifying Radicals
Square Roots with Variables (Simplifying Math)
How to Simplify Radicals (NancyPi)
Learn how to simplify a radical
Simplifying Radicals (Algebra 2)
Multiplying Radicals and Then Simplifying
Комментарии
 0:05:14
0:05:14
 0:02:03
0:02:03
 0:03:42
0:03:42
 0:03:23
0:03:23
 0:02:30
0:02:30
 0:04:42
0:04:42
 0:02:49
0:02:49
 0:01:38
0:01:38
 0:53:54
0:53:54
 0:12:01
0:12:01
 0:13:14
0:13:14
 0:01:46
0:01:46
 0:02:12
0:02:12
 0:03:09
0:03:09
 0:10:46
0:10:46
 0:43:40
0:43:40
 0:02:39
0:02:39
 0:01:30
0:01:30
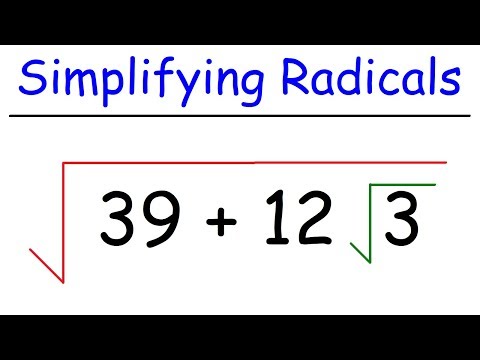 0:10:14
0:10:14
 0:07:50
0:07:50
 0:17:51
0:17:51
 0:04:09
0:04:09
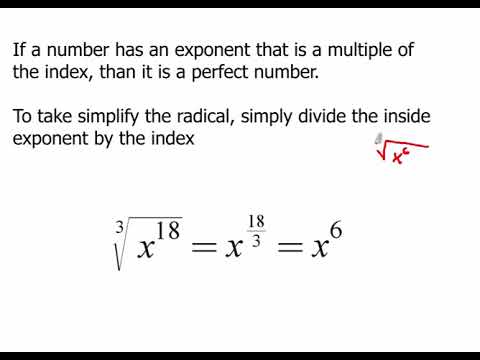 0:07:07
0:07:07
 0:04:05
0:04:05