Modularity and Effective Mordell - Levent Alpoge, Harvard University, USA

Chair for the Talk: Soumya Sankar, Ohio State USA.

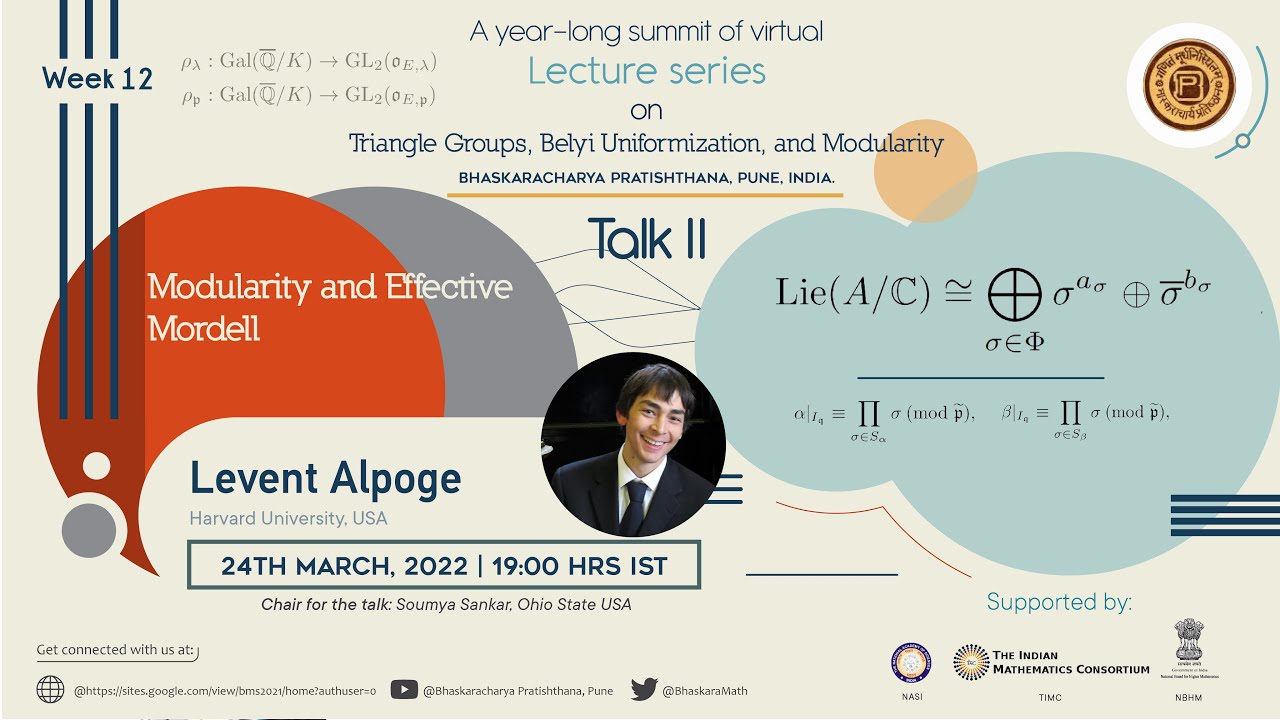
Abstract: I will give a finite-time algorithm that, on input (g,K,S) with g greater than 0, K a totally real number field of odd degree, and S a finite set of places of K, outputs the finitely many g-dimensional abelian varieties A/K which are of GL_2-type over K and have good reduction outside S.

The point of this is to effectively compute the S-integral K-points on a Hilbert modular variety, and the point of that is to be able to compute all K-rational points on complete curves inside such varieties.

This gives effective height bounds for rational points on infinitely many curves and (for each curve) over infinitely many number fields. For example one gets effective height bounds for odd-degree totally real points on x^6 + 4y^3 = 1, by using the hypergeometric family associated to the arithmetic triangle group Δ (3,6,6).

[adaniam]

levent beyle iletişime geçmemin bir yolu var mı? Yardımcı olursanız mutluluk duyarım.