filmov
tv
Classification of PDEs into Elliptic, Hyperbolic and Parabolic

Показать описание
In this tutorial I will teach you how to classify Partial differential Equations (or PDE's for short) into the three categories. This is based on the number of real characteristics that the PDE has. The class of PDE has important consequences. We will also do two worked examples to ensure that you are following the theory. It's really very simple.
The technique is very simple and just involves applying the discriminant (think quadratic equation), B^2-4AC with the coefficients being determined by comparing with a reference equation.
The technique is very simple and just involves applying the discriminant (think quadratic equation), B^2-4AC with the coefficients being determined by comparing with a reference equation.
Classification of PDEs into Elliptic, Hyperbolic and Parabolic
PDE Classification: Elliptic, Parabolic, and Hyperbolic
Classification of PDE- Hyperbolic Parabolic, Elliptic
Classification of Partial Differential Equations of Second Order | Elliptic Parabolic and Hyperbolic
Classification of Second Order Partial Differential Equations into Elliptic,Parabolic and Hyperbolic
But what is a partial differential equation? | DE2
Classification of second order partial differential equation with examples
Lecture 2 Classification of Partial DIfferential Equation
Partial differential equations (PDEs) in engineering mathematics: introduction and classification
Classification of Second-Order PDE | Partial Differential Equation Example & Concepts by GP Si...
Partial Differential Equations Overview
How to classify second order PDE
elliptic partial differential equations|| canonical form|| pde
8.1.2-PDEs: Classification of Partial Differential Equations
Classifications of Second-order Partial Differential Equations | Examples
Hyperbolic,parabolic and elliptical form of partial differential equations
8.1.3-PDEs: Classification Continued--Classifying Second-Order PDEs
Classification of PDEs into Parabolic, Hyperbolic and Elliptic || 2nd order PDE || Math Institute
Nature of PDE || Classification of PDE || Hyperbolic, Parabolic, Elliptic pde's
Classification of PDEs into Elliptic, Hyperbolic and Parabolic with non constant coefficient
Explained: PDE Classification [CFD]
Classification of PDEs | Boundary Value Problems | LetThereBeMath|
Review of governing equations: classification of PDEs
07.01 Classifying PDEs
Комментарии
 0:06:50
0:06:50
 0:04:35
0:04:35
 0:11:43
0:11:43
 0:22:57
0:22:57
 0:28:07
0:28:07
 0:17:39
0:17:39
 0:13:17
0:13:17
 0:07:58
0:07:58
 0:04:26
0:04:26
 0:12:20
0:12:20
 0:26:18
0:26:18
 0:11:59
0:11:59
 0:15:06
0:15:06
 0:10:55
0:10:55
 0:23:37
0:23:37
 0:05:00
0:05:00
 0:08:21
0:08:21
 0:19:05
0:19:05
 0:03:55
0:03:55
 0:03:58
0:03:58
 0:10:51
0:10:51
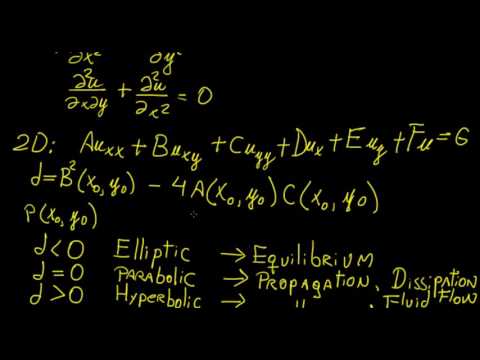 0:15:47
0:15:47
 0:51:06
0:51:06
 0:10:36
0:10:36