filmov
tv
Rolle's Theorem
Показать описание
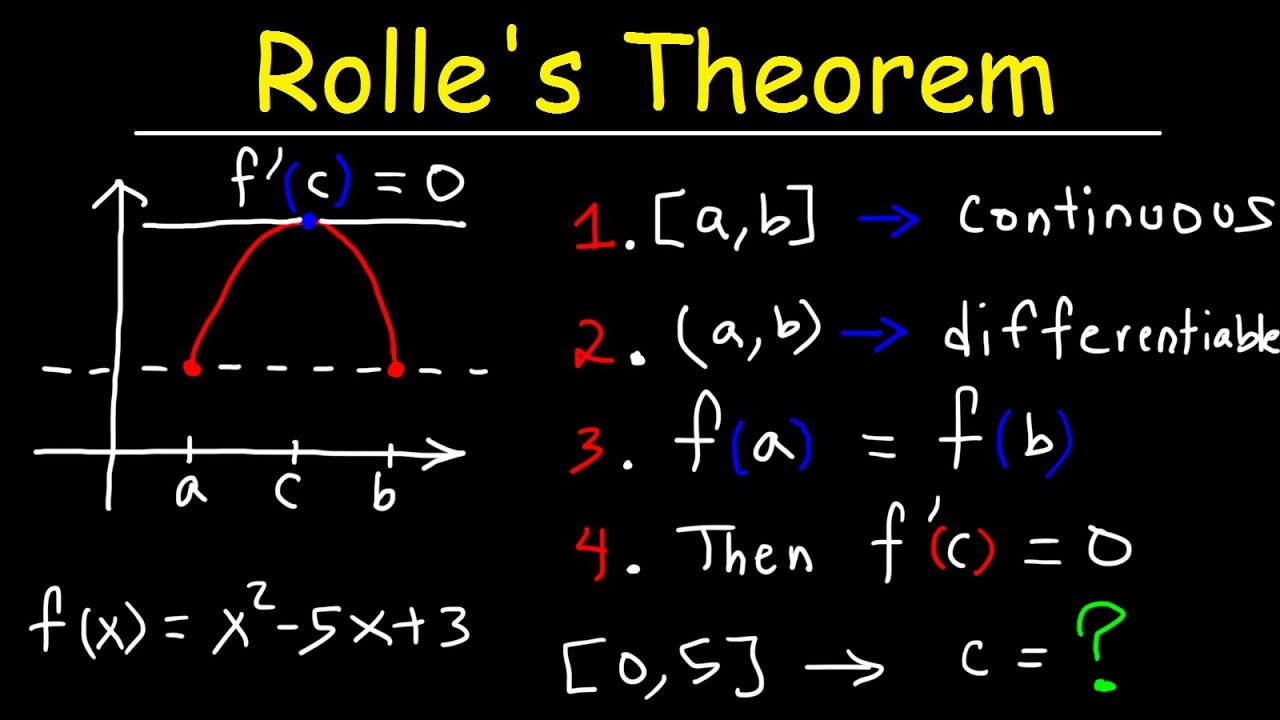
This calculus video tutorial provides a basic introduction into rolle's theorem. It contains plenty of examples and practice problems on how to find the value of c that will satisfy the conclusion of rolle's theorem on the closed interval [a, b]. For rolle's theorem to apply to a function, there are 3 conditions that must be met. (1) The function must be continuous on the closed interval [a, b]. (2) The function must be differentiable on the open interval (a, b). (3) f(a) = f(b). If these conditions are met, then f'(c)=0. There were be some number c in the interval [a, b] where a horizontal tangent line will exist.
___________________________
Introduction to Limits:
Derivatives - Fast Review:
Introduction to Related Rates:
_____________________________
Extreme Value Theorem:
Finding Critical Numbers:
Local Maximum & Minimum:
Absolute Extrema:
Rolle's Theorem:
________________________________
Mean Value Theorem:
Increasing and Decreasing Functions:
First Derivative Test:
Concavity & Inflection Points:
Second Derivative Test:
_________________________________
L'Hopital's Rule:
Curve Sketching With Derivatives:
Newton's Method:
Optimization Problems:
_______________________________________
Final Exams and Video Playlists:
Full-Length Videos and Worksheets:
___________________________
Introduction to Limits:
Derivatives - Fast Review:
Introduction to Related Rates:
_____________________________
Extreme Value Theorem:
Finding Critical Numbers:
Local Maximum & Minimum:
Absolute Extrema:
Rolle's Theorem:
________________________________
Mean Value Theorem:
Increasing and Decreasing Functions:
First Derivative Test:
Concavity & Inflection Points:
Second Derivative Test:
_________________________________
L'Hopital's Rule:
Curve Sketching With Derivatives:
Newton's Method:
Optimization Problems:
_______________________________________
Final Exams and Video Playlists:
Full-Length Videos and Worksheets:
0:19:32
Rolle's Theorem
0:07:24
Rolle's Theorem with Examples
0:11:11
Rolle's Theorem Explained (with proof) | Calculus 1
0:05:12
How to use ROLLE'S THEOREM (KristaKingMath)
0:07:54
Rolle's Theorem
0:06:15
Rolle's Theorem Explained in Hindi l Engineering Mathematics
0:15:54
Real Analysis | Mean Value Theorem | Rolle's Theorem - Proof & Examples
0:08:38
VERIFY ROLLES THEOREM|ROLLES THEOREM|PROVE| DEFINITION|IMPORTANT QUESTION
1:28:17
📚 Rewind Series | Continuity and Differentiability
0:00:54
Rolle's Theorem | Mean Value Theorem | #jeedailyconcepts
0:12:22
State and Prove Rolle's theorem | Real Analysis
0:23:40
The Mean Value Theorem & Rolle's Theorem | Calculus 1 | Math with Professor V
1:07:29
Calculus 1: Lecture 3.2 Rolle's Theorem and the Mean Value Theorem
0:00:15
Rolle's Theorem #realanalysis
0:56:19
SINGLE VARIABLE CALCULUS |Differential Calculus|Rolle's Theorem|Lecture 01|All University
0:00:56
Rolle's Theorem!!
0:07:21
Theorem 7.6C # Rolle's Theorem # verify Rolle's theorem # Real analysis # Tamil
0:13:37
Rolle’s Theorem Proof
0:05:44
Verify Rolle's Theorem and Find a number to satisfy it | ACT
0:12:43
Rolle's Theorem and Mean Value Theorem
0:15:28
Rolle’s Theorem | Proof | State and Prove Rolle’s Theorem | Bsc
0:19:40
Mean Value Theorem
0:14:29
ROLLE'S THEOREM QUESTION | APPLICATION OF ROLLE'S THEOREM | PART - 1
0:10:14
Rolle's Mean Value Theorem | Proof
Комментарии