filmov
tv
⛓Sequence Increasing or Decreasing problem ! ! ! ! !

Показать описание
__________________
In this video you will learn how to show whether the given Sequence 2^n/(n+1)! increases or decreases.
0:22 Understanding the Sequence increase/decrease
1:13 Checking increase/decrease of the given Sequence - showing the (n+1)st term being smaller than the nth term - decreasing sequence
Related Videos to this topic:
Sequence n/(n+1): Convergence using Formal Definition
Sequence cosn/n^2: Convergence using Squeeze Theorem
Sequence (-1)^n · [(e^-n)/n]: Two Methods to Show Convergence to 0 (zero)
Sequence (3n^2-1)/(4n^2+1): Converges?
Sequence ln(2n+1)-ln(n): Converges?
Sequence n2^n/3^n: Converges?
Sequence (e^n+2)/[e^(2n)-1]: Converges?
Sequence ne^-n: Converges?
Sequence (-1)^n · [(n+2)/(3n-1)]: Converges?
Sequence n!/2^n: Converges?
Sequence e^n/n: Increases or Decreases?
Sequence (n+3)/(n+2): Increases or Decreases?
Sequence e^(1/n): Boundary Value
Sequence (1-6n)/(n+3): Boundary Value
Sequence sin(n^2)/(n+1): Boundary Value
Sequence (3n^2-2)/(n^2+1): Boundary Value
______________________
In this video you will learn how to show whether the given Sequence 2^n/(n+1)! increases or decreases.
0:22 Understanding the Sequence increase/decrease
1:13 Checking increase/decrease of the given Sequence - showing the (n+1)st term being smaller than the nth term - decreasing sequence
Related Videos to this topic:
Sequence n/(n+1): Convergence using Formal Definition
Sequence cosn/n^2: Convergence using Squeeze Theorem
Sequence (-1)^n · [(e^-n)/n]: Two Methods to Show Convergence to 0 (zero)
Sequence (3n^2-1)/(4n^2+1): Converges?
Sequence ln(2n+1)-ln(n): Converges?
Sequence n2^n/3^n: Converges?
Sequence (e^n+2)/[e^(2n)-1]: Converges?
Sequence ne^-n: Converges?
Sequence (-1)^n · [(n+2)/(3n-1)]: Converges?
Sequence n!/2^n: Converges?
Sequence e^n/n: Increases or Decreases?
Sequence (n+3)/(n+2): Increases or Decreases?
Sequence e^(1/n): Boundary Value
Sequence (1-6n)/(n+3): Boundary Value
Sequence sin(n^2)/(n+1): Boundary Value
Sequence (3n^2-2)/(n^2+1): Boundary Value
______________________
 0:05:44
0:05:44
 0:02:50
0:02:50
 0:04:11
0:04:11
 0:07:09
0:07:09
 0:03:33
0:03:33
 0:10:54
0:10:54
 0:02:12
0:02:12
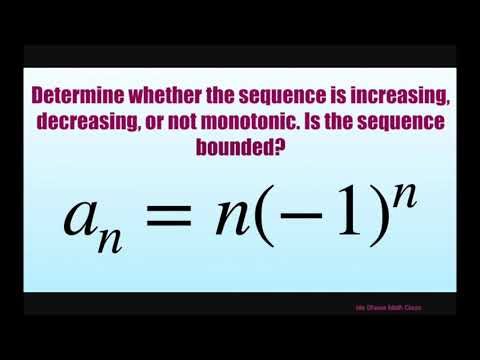 0:01:49
0:01:49
 0:01:57
0:01:57
 0:04:00
0:04:00
 0:08:21
0:08:21
 0:33:09
0:33:09
 0:36:03
0:36:03
 0:02:13
0:02:13
 0:01:49
0:01:49
 0:09:40
0:09:40
 0:13:14
0:13:14
 0:32:04
0:32:04
 0:06:45
0:06:45
 0:12:57
0:12:57
 0:07:02
0:07:02
 0:30:13
0:30:13
 0:15:10
0:15:10
 0:34:22
0:34:22