filmov
tv
Laplace Transforms 10: Examples with the Heaviside Function
Показать описание
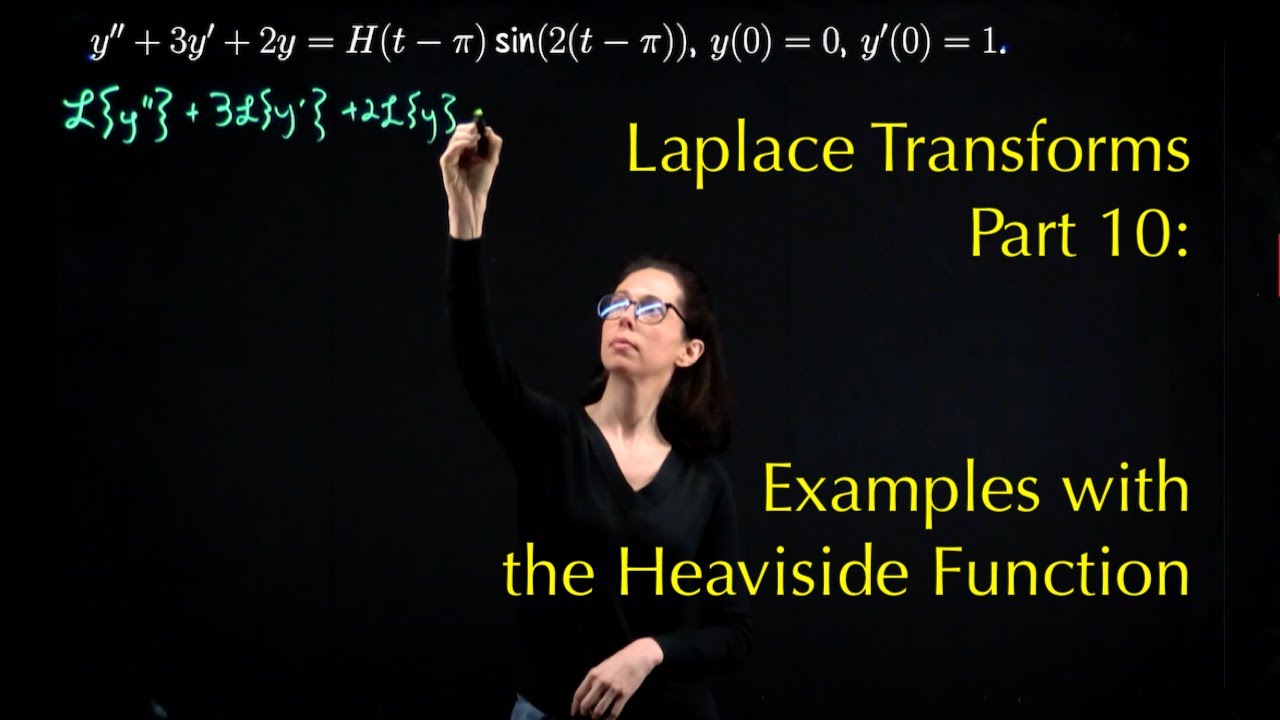
(Video 10 of more to come) In the last video, we met the Heaviside function, also known as the unit step function, and discussed its utility in delaying the start of an external force. This video focuses on applying the Heaviside function to turn on a signal after a certain time. We examine two examples: a basic first-order differential equation and a more complex second-order one.
Error: at 9:14, I should write 3y(0), but luckily, y(0)=0, so it does not affect the final result.
Our first example involves the differential equation dy/dt = H(t - 2). The solution is a piece-wise function that remains constant at one until \( t = 2 \) and then linearly increases. For the second example, we solve a second-order differential equation y'' + 3y' + 2y = H(t - pi)sin(2(t - pi)). This equation indicates the onset of a sinusoidal forcing function at t = pi. We use the Laplace transform to solve this equation, incorporating initial conditions and applying identities for first and second derivatives. The solution involves partial fraction decomposition and the Laplace transform of delayed signals.
#mathematics #math #HeavisideFunction #DifferentialEquations #LaplaceTransform #SignalProcessing #EngineeringMath #ControlSystems #UnitStepFunction #HarmonicOscillator
Error: at 9:14, I should write 3y(0), but luckily, y(0)=0, so it does not affect the final result.
Our first example involves the differential equation dy/dt = H(t - 2). The solution is a piece-wise function that remains constant at one until \( t = 2 \) and then linearly increases. For the second example, we solve a second-order differential equation y'' + 3y' + 2y = H(t - pi)sin(2(t - pi)). This equation indicates the onset of a sinusoidal forcing function at t = pi. We use the Laplace transform to solve this equation, incorporating initial conditions and applying identities for first and second derivatives. The solution involves partial fraction decomposition and the Laplace transform of delayed signals.
#mathematics #math #HeavisideFunction #DifferentialEquations #LaplaceTransform #SignalProcessing #EngineeringMath #ControlSystems #UnitStepFunction #HarmonicOscillator
0:12:05
Intro to the Laplace Transform & Three Examples
0:00:08
I heard you need some Laplace transforms
0:08:30
Examples of Laplace Transformation
0:00:46
How to solve differential equations
0:06:30
Laplace Transform & Region of Convergence Problem Example 1
0:06:27
Laplace Transform of a Piecewise Function (Unit Step Function)
0:08:19
Example of Inverse Laplace's Transform for repeated poles using residual method
0:06:39
9. Laplace Transforms | Most Important Problem#1 | Complete Concept
0:22:27
Laplace Transform and ROC of Standard Signals | Laplace Transform of Impulse Function, Signal System
0:29:13
🔵26 - Definition of Laplace Transform: Solving Basic Laplace Transforms
0:16:52
Signals & Systems - Laplace Transforms - working examples - 10
0:05:31
Laplace Transform (Solved Problem 10)
0:00:44
Inverse Laplace Transform of 1/s^5
0:09:52
Laplace transforms examples table
0:27:54
Laplace Transform 10 – Unit Step Functions | Heaviside Functions | Piecewise Functions with Examples...
0:43:42
Practice Problems on Laplace Transform
0:04:34
inverse laplace transform, example#5, with completing the square
3:10:51
Laplace Transform Ultimate Study Guide
0:17:16
10. APPLICATIONS OF LAPLACE TRANSFORMS TO SOLVE DIFFERENTIAL EQUATIONS : EXAMPLE 1
0:10:10
10 - Solve Differential Equations (ODEs) w/ Laplace Transforms, Part 2
0:04:06
Inverse Laplace Transform, Sect 7.4#7
0:27:55
🔵27 - Elementary Properties of the Laplace Transform with solved examples on each Property
0:25:14
09 - Solve Differential Equations with Laplace Transforms, Part 1
0:21:44
🔵33 - Solving Initial Value Problems using Laplace Transforms method
Комментарии