filmov
tv
Error Correcting Codes 2c: Linear Codes - Parity-Check Matrix

Показать описание
Error Correcting Codes 2c: Linear Codes - Parity-Check Matrix
Error Correcting Codes 2a: Linear Codes - Generator Matrix
Error Correcting Codes 2b: Linear Codes - Minimum Distance
Error Correcting Codes 1: Introduction + Hamming (7,4) Code
Linear Algebra Lecture 13: Error correcting codes
Linear Algebra - Hamming's error correcting codes - 01 - Hamming matrices
Linear Algebra - Hamming's error correcting codes - 02 - Properties of Hamming matrices
Linear Algebra - Hamming's error correcting codes - 04 - Parity bits
Linear Block Codes Example
Ch 10 Linear Block Codes
Error correcting codes
Error Syndromes & Error Correction in Linear Block Code in Digital Communication
FEHLERERKENNUNG/-KORREKTUR (Error Correcting Code) | Digitaltechnik
Linear Block Codes Part 2
Linear Codes Pt 2, Parity check matrix
Detection and Correction of Errors Problem 1 - Codes and Group Codes - Discrete Mathematics
Linear Block Codes: Error detection and Correction Capability and Parity Check Matrix
Linar block coding-calculation parity check matrix and syndrome
Applications of Error Correcting Codes by Luis Alberto Perez
Syndrome, Error Correction and Error Detection
Linear codes
Linear Algebra Exercises 4 - Inverse matrices and error correcting codes
Linear block coding
Complete Example of Linear Block Code in Digital Communication by Engineering Funda
Комментарии
 0:12:32
0:12:32
 0:10:25
0:10:25
 0:07:59
0:07:59
 0:22:31
0:22:31
 0:50:26
0:50:26
 0:17:37
0:17:37
 0:14:52
0:14:52
 0:10:12
0:10:12
 0:21:58
0:21:58
 0:10:18
0:10:18
 0:13:20
0:13:20
 0:07:13
0:07:13
 0:06:08
0:06:08
 0:17:56
0:17:56
 0:07:19
0:07:19
 0:07:24
0:07:24
 0:13:49
0:13:49
 0:04:12
0:04:12
 0:02:59
0:02:59
 0:19:31
0:19:31
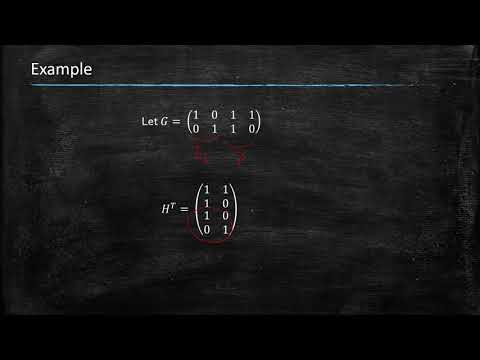 0:21:31
0:21:31
 1:32:25
1:32:25
 0:46:07
0:46:07
 0:19:36
0:19:36