filmov
tv
Two-way ANOVA by hand

Показать описание
In this video third video about two-way ANOVA, we will see how to calculate a two-way ANOVA (between-between) by hand so that we fully understand the ANOVA table. We will calculate the group means, grand mean, sum of squares within, between and for the interaction, degrees of freedom, F-ratios and p-values.
Two-way ANOVA by hand
Two-Way ANOVA - all Equations - calculated by Hand
Statistics: Two way ANOVA
Two-Way ANOVA - Full Course
2-way (between-subjects) ANOVA by hand
Two Way ANOVA (2X3) Balanced Design Hand Calculations Full Details
How to Calculate a Two Way ANOVA (factorial analysis)
Introduction to Two Way ANOVA (Factorial Analysis)
Two Way ANOVA (2X3) Unbalanced Design - Hand Calculations Full Details
2 x 3 factorial ANOVA by hand
How To... Perform a One-Way ANOVA Test (By Hand)
Calculating an ANOVA by hand
How To... Perform a Two-Way ANOVA in Excel 2013
Factorial ANOVA, Two Independent Factors
Perform a Two-Way ANOVA in Excel by Hand - Engineering Statistics
Factorial Anova by hand
How to Calculate a One-Way ANOVA by Hand
One Factor vs. Two Factor ANOVA [One Way vs Two Way]
How to Interpret the Results of A Two Way ANOVA (Factorial)
ANOVA 1: Calculating SST (total sum of squares) | Probability and Statistics | Khan Academy
ANOVA Two way | F Test without Replication | Problem & Solution | Hypothesis Testing Statistics
Two Way ANOVA's
Compute a simple ANOVA by hand
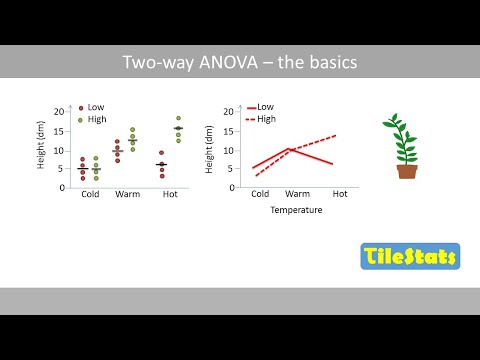
Two-way ANOVA - the basics | interaction | two-way vs one-way
Комментарии
 0:08:48
0:08:48
 0:08:06
0:08:06
 0:06:07
0:06:07
 0:16:21
0:16:21
 0:28:57
0:28:57
 0:59:53
0:59:53
 0:18:02
0:18:02
 0:08:01
0:08:01
 1:02:54
1:02:54
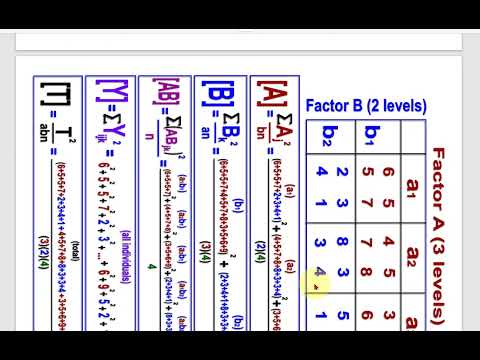 0:35:41
0:35:41
 0:16:28
0:16:28
 0:03:41
0:03:41
 0:06:46
0:06:46
 0:09:10
0:09:10
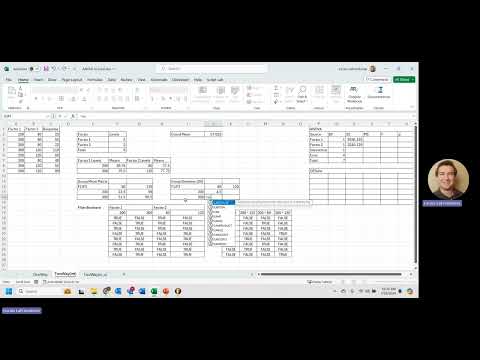 0:20:43
0:20:43
 0:19:50
0:19:50
 0:26:23
0:26:23
 0:03:48
0:03:48
 0:17:41
0:17:41
 0:07:39
0:07:39
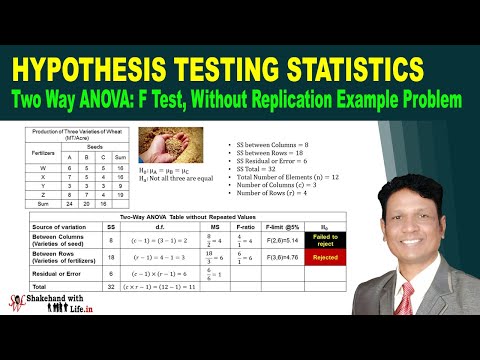 0:28:15
0:28:15
 0:08:31
0:08:31
 0:07:40
0:07:40
 0:15:31
0:15:31