filmov
tv
Finding the derivative using product rule

Показать описание
Welcome to our YouTube video on finding the derivative using the product rule!
In calculus, finding the derivative of a function is an essential skill. The derivative tells us how fast a function is changing at any given point and is used to solve a variety of problems in fields such as physics, engineering, and economics.
In this video, we will be focusing on the product rule, one of the fundamental rules of differentiation. The product rule allows us to find the derivative of the product of two functions. We will walk you through step-by-step on how to use the product rule and provide several examples to help solidify your understanding.
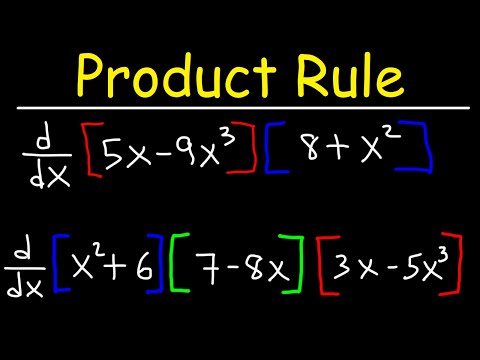
To begin, let’s review the product rule. The product rule states that if we have two functions, f(x) and g(x), their product, h(x) = f(x)g(x), can be differentiated using the following formula:
h'(x) = f'(x)g(x) + f(x)g'(x)
where f'(x) and g'(x) represent the derivatives of f(x) and g(x), respectively.
Now, let’s apply the product rule to a few examples to see how it works in practice.
Example 1: Differentiate x^2sin(x)
To differentiate x^2sin(x), we need to identify our two functions, f(x) and g(x):
f(x) = x^2
g(x) = sin(x)
Next, we need to find the derivatives of f(x) and g(x):
f'(x) = 2x
g'(x) = cos(x)
Now we can plug these into the product rule formula:
h'(x) = f'(x)g(x) + f(x)g'(x)
h'(x) = 2xsin(x) + x^2cos(x)
And there we have it – the derivative of x^2sin(x) using the product rule!
Example 2: Differentiate e^xln(x)
To differentiate e^xln(x), we first need to rewrite the function using the laws of logarithms:
e^xln(x) = x^x
Now we can identify our two functions:
f(x) = e^x
g(x) = ln(x)
Next, we find the derivatives:
f'(x) = e^x
g'(x) = 1/x
Now we can use the product rule:
h'(x) = f'(x)g(x) + f(x)g'(x)
h'(x) = e^xln(x) + e^x/x
And there we have it – the derivative of e^xln(x) using the product rule!
Example 3: Differentiate (2x+1)^3(x^2+1)
To differentiate (2x+1)^3(x^2+1), we identify our two functions:
f(x) = (2x+1)^3
g(x) = x^2+1
Next, we find the derivatives:
f'(x) = 3(2x+1)^2(2)
g'(x) = 2x
Now we can use the product rule:
h'(x) = f'(x)g(x) + f(x)g'(x)
h'(x) = (2x+1)^3(2x) + (2x+1)^2(2)(x^2+1)
And there we have it – the derivative of (2x+1)^3(x^2+1) using the product rule!
As you can see from these examples, the product rule is a powerful tool that allows us to find the derivative of the product of two functions. It is essential to master this rule asWelcome to our YouTube video on finding the derivative using the product rule!
In calculus, finding the derivative of a function is an essential skill. The derivative tells us how fast a function is changing at any given point and is used to solve a variety of problems in fields such as physics, engineering, and economics.
In this video, we will be focusing on the product rule, one of the fundamental rules of differentiation. The product rule allows us to find the derivative of the product of two functions. We will walk you through step-by-step on how to use the product rule and provide several examples to help solidify your understanding.
To begin, let’s review the product rule. The product rule states that if we have two functions, f(x) and g(x), their product, h(x) = f(x)g(x), can be differentiated using the following formula:
h'(x) = f'(x)g(x) + f(x)g'(x)
where f'(x) and g'(x) represent the derivatives of f(x) and g(x), respectively.
Now, let’s apply the product rule to a few examples to see how it works in practice.
Example 1: Differentiate x^2sin(x)
To differentiate x^2sin(x), we need to identify our two functions, f(x) and g(x):
f(x) = x^2
g(x) = sin(x)
Next, we need to find the derivatives of f(x) and g(x):
f'(x) = 2x
g'(x) = cos(x)
Now we can plug these into the product rule formula:
h'(x) = f'(x)g(x) + f(x)g'(x)
h'(x) = 2xsin(x) + x^2cos(x)
And there we have it – the derivative of x^2sin(x) using the product rule!
Example 2: Differentiate e^xln(x)
To differentiate e^xln(x), we first need to rewrite the function using the laws of logarithms:
e^xln(x) = x^x
Now we can identify our two functions:
f(x) = e^x
g(x) = ln(x)
Next, we find the derivatives:
f'(x) = e^x
g'(x) = 1/x
Now we can use the product rule:
h'(x) = f'(x)g(x) + f(x)g'(x)
h'(x) = e^xln(x) + e^x/x
And there we have it – the derivative of e^xln(x) using the product rule!
Example 3: Differentiate (2x+1)^3(x^2+1)
To differentiate (2x+1)^3(x^2+1), we identify our two functions:
f(x) = (2x+1)^3
g(x) = x^2+1
Next, we find the derivatives:
f'(x) = 3(2x+1)^2(2)
g'(x) = 2x
Now we can use the product rule:
h'(x) = f'(x)g(x) + f(x)g'(x)
h'(x) = (2x+1)^3(2x) + (2x+1)^2(2)(x^2+1)
And there we have it – the derivative of (2x+1)^3(x^2+1) using the product rule!
As you can see from these examples, the product rule is a powerful tool that allows us to find the derivative of the product of two functions. It is essential to master this rule as
In calculus, finding the derivative of a function is an essential skill. The derivative tells us how fast a function is changing at any given point and is used to solve a variety of problems in fields such as physics, engineering, and economics.
In this video, we will be focusing on the product rule, one of the fundamental rules of differentiation. The product rule allows us to find the derivative of the product of two functions. We will walk you through step-by-step on how to use the product rule and provide several examples to help solidify your understanding.
To begin, let’s review the product rule. The product rule states that if we have two functions, f(x) and g(x), their product, h(x) = f(x)g(x), can be differentiated using the following formula:
h'(x) = f'(x)g(x) + f(x)g'(x)
where f'(x) and g'(x) represent the derivatives of f(x) and g(x), respectively.
Now, let’s apply the product rule to a few examples to see how it works in practice.
Example 1: Differentiate x^2sin(x)
To differentiate x^2sin(x), we need to identify our two functions, f(x) and g(x):
f(x) = x^2
g(x) = sin(x)
Next, we need to find the derivatives of f(x) and g(x):
f'(x) = 2x
g'(x) = cos(x)
Now we can plug these into the product rule formula:
h'(x) = f'(x)g(x) + f(x)g'(x)
h'(x) = 2xsin(x) + x^2cos(x)
And there we have it – the derivative of x^2sin(x) using the product rule!
Example 2: Differentiate e^xln(x)
To differentiate e^xln(x), we first need to rewrite the function using the laws of logarithms:
e^xln(x) = x^x
Now we can identify our two functions:
f(x) = e^x
g(x) = ln(x)
Next, we find the derivatives:
f'(x) = e^x
g'(x) = 1/x
Now we can use the product rule:
h'(x) = f'(x)g(x) + f(x)g'(x)
h'(x) = e^xln(x) + e^x/x
And there we have it – the derivative of e^xln(x) using the product rule!
Example 3: Differentiate (2x+1)^3(x^2+1)
To differentiate (2x+1)^3(x^2+1), we identify our two functions:
f(x) = (2x+1)^3
g(x) = x^2+1
Next, we find the derivatives:
f'(x) = 3(2x+1)^2(2)
g'(x) = 2x
Now we can use the product rule:
h'(x) = f'(x)g(x) + f(x)g'(x)
h'(x) = (2x+1)^3(2x) + (2x+1)^2(2)(x^2+1)
And there we have it – the derivative of (2x+1)^3(x^2+1) using the product rule!
As you can see from these examples, the product rule is a powerful tool that allows us to find the derivative of the product of two functions. It is essential to master this rule asWelcome to our YouTube video on finding the derivative using the product rule!
In calculus, finding the derivative of a function is an essential skill. The derivative tells us how fast a function is changing at any given point and is used to solve a variety of problems in fields such as physics, engineering, and economics.
In this video, we will be focusing on the product rule, one of the fundamental rules of differentiation. The product rule allows us to find the derivative of the product of two functions. We will walk you through step-by-step on how to use the product rule and provide several examples to help solidify your understanding.
To begin, let’s review the product rule. The product rule states that if we have two functions, f(x) and g(x), their product, h(x) = f(x)g(x), can be differentiated using the following formula:
h'(x) = f'(x)g(x) + f(x)g'(x)
where f'(x) and g'(x) represent the derivatives of f(x) and g(x), respectively.
Now, let’s apply the product rule to a few examples to see how it works in practice.
Example 1: Differentiate x^2sin(x)
To differentiate x^2sin(x), we need to identify our two functions, f(x) and g(x):
f(x) = x^2
g(x) = sin(x)
Next, we need to find the derivatives of f(x) and g(x):
f'(x) = 2x
g'(x) = cos(x)
Now we can plug these into the product rule formula:
h'(x) = f'(x)g(x) + f(x)g'(x)
h'(x) = 2xsin(x) + x^2cos(x)
And there we have it – the derivative of x^2sin(x) using the product rule!
Example 2: Differentiate e^xln(x)
To differentiate e^xln(x), we first need to rewrite the function using the laws of logarithms:
e^xln(x) = x^x
Now we can identify our two functions:
f(x) = e^x
g(x) = ln(x)
Next, we find the derivatives:
f'(x) = e^x
g'(x) = 1/x
Now we can use the product rule:
h'(x) = f'(x)g(x) + f(x)g'(x)
h'(x) = e^xln(x) + e^x/x
And there we have it – the derivative of e^xln(x) using the product rule!
Example 3: Differentiate (2x+1)^3(x^2+1)
To differentiate (2x+1)^3(x^2+1), we identify our two functions:
f(x) = (2x+1)^3
g(x) = x^2+1
Next, we find the derivatives:
f'(x) = 3(2x+1)^2(2)
g'(x) = 2x
Now we can use the product rule:
h'(x) = f'(x)g(x) + f(x)g'(x)
h'(x) = (2x+1)^3(2x) + (2x+1)^2(2)(x^2+1)
And there we have it – the derivative of (2x+1)^3(x^2+1) using the product rule!
As you can see from these examples, the product rule is a powerful tool that allows us to find the derivative of the product of two functions. It is essential to master this rule as
 0:11:11
0:11:11
 0:02:55
0:02:55
 0:14:38
0:14:38
 0:05:35
0:05:35
 0:01:45
0:01:45
 0:09:25
0:09:25
 0:04:44
0:04:44
 0:52:51
0:52:51
 1:55:51
1:55:51
 0:04:30
0:04:30
 0:04:54
0:04:54
 0:01:00
0:01:00
 0:14:30
0:14:30
 0:08:18
0:08:18
 0:01:38
0:01:38
 0:05:26
0:05:26
 0:04:39
0:04:39
 0:09:04
0:09:04
 0:04:37
0:04:37
 0:04:38
0:04:38
 0:01:56
0:01:56
 0:23:31
0:23:31
 0:10:06
0:10:06
 0:18:40
0:18:40