Rakesh | Fixed angle inverse scattering for Riemannian metrics

Days on Diffraction 2024. Mini-symposium “Inverse Problems”. Tuesday, 11 June, 2024
Rakesh (University of Delaware)
Fixed angle inverse scattering for Riemannian metrics

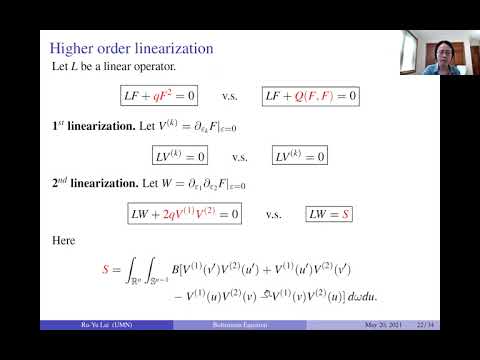
An inhomogeneous acoustic medium, modeled by a Riemannian metric, is probed by a finite number of plane waves and the resultant time dependent waves are measured on the boundary of a ball enclosing the inhomogeneous part of the medium. We describe our partial results about the recovery of the Riemannian metric from the boundary measurements. This is a formally determined inverse problem for the operator $\partial^2_t - \Delta_g$ for a Riemannian metric $g$ on $R^n$, consisting of the recovery of the metric g from the boundary measurements. We show we can distinguish between g and the Euclidean metric using boundary data corresponding to $n(n+1)/2$ different plane wave sources. This talk is based on work done with Lauri Oksanen and Mikko Salo.