Vector SOLENOIDAL example simple steps used

Показать описание

In this video explaining Vector SOLENOIDAL example interesting and very good.
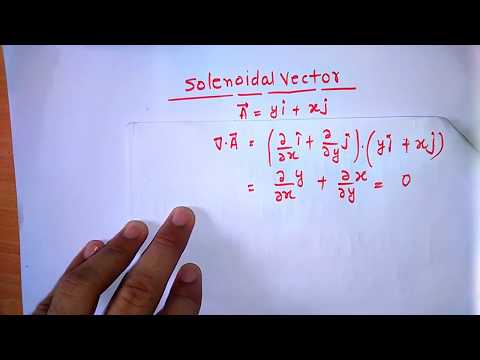
A vector is solenoidal if it has zero divergence which means that the net flow of the vector out of any closed surface is zero. In other words the vector field has no sources or sinks within the region of interest. One example of a solenoidal vector is the magnetic field in a region where there are no magnetic monopoles.

#easymathseasytricks #vectorsolenoidal

18MAT21 MODULE 1:Vector Calculus

18MAT21 MODULE 2:Differential Equation higher order

18MAT21 MODULE 3: Partial differential equations

18MAT21 MODULE 4: Infiinite series & Power series solution

18MAT21 MODULE 5: Numerical methods

18MAT11 Module1: Differential Calculus1

18MAT11 Module2: differential Calculus2

18MAT11 Module4: Ordinary differential equations

Linear Algebra: 18MAT11 MODULE 5

LAPLACE TRANSFORM : 18MAT31

Fourier Transforms Z-transform : 18MAT31 & 17MAT31

Fourier Series: 18MAT31 & 17MAT31

Calculus of Variation & Numerical Methods 18MAT31

Numerical Methods ODE's: 18MAT31 & 17MAT41

Joint Probability & Sampling Theory: 18MAT41 & 17MAT41

Probability Distributions: 18MAT41 & 17MAT41

Calculus of Complex Functions: 18MAT41 & 17MAT41

Curve fitting & Statistical Method 18MAT41 17MAT31