filmov
tv
Prove that the Square of any Odd Integer is Odd

Показать описание
Prove that the Square of any Odd Integer is Odd
If you enjoyed this video please consider liking, sharing, and subscribing.
There are several ways that you can help support my channel:)
************Udemy Courses(Please Use These Links If You Sign Up!)*************
Abstract Algebra Course
Advanced Calculus Course
Calculus 1 Course
Calculus 2 Course
Calculus 3 Course
Calculus Integration Insanity
Differential Equations Course
College Algebra Course
How to Write Proofs with Sets Course
How to Write Proofs with Functions Course
Statistics with StatCrunch Course
Math Graduate Programs, Applying, Advice, Motivation
Daily Devotionals for Motivation with The Math Sorcerer
Thank you:)
If you enjoyed this video please consider liking, sharing, and subscribing.
There are several ways that you can help support my channel:)
************Udemy Courses(Please Use These Links If You Sign Up!)*************
Abstract Algebra Course
Advanced Calculus Course
Calculus 1 Course
Calculus 2 Course
Calculus 3 Course
Calculus Integration Insanity
Differential Equations Course
College Algebra Course
How to Write Proofs with Sets Course
How to Write Proofs with Functions Course
Statistics with StatCrunch Course
Math Graduate Programs, Applying, Advice, Motivation
Daily Devotionals for Motivation with The Math Sorcerer
Thank you:)
Prove that the Square of any Odd Integer is Odd
How to prove that the square of an odd number is also odd - Melissa Maths
How To Prove The Quadratic Formula By Completing The Square
Can You Prove that one of these large numbers is not a perfect square?
direct proof 2: Prove that the product of perfect squares is a perfect square logic
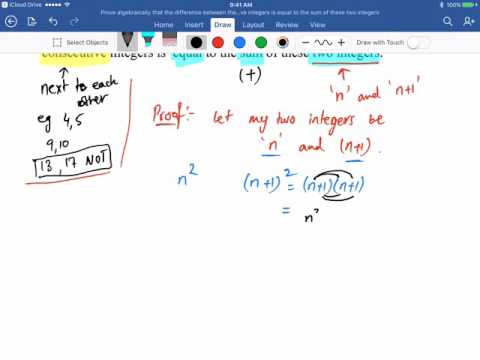
Prove algebraically that the difference between the squares of any two consecutive integers is equal
Prove that the there is No Rational Number Whose Square is 2
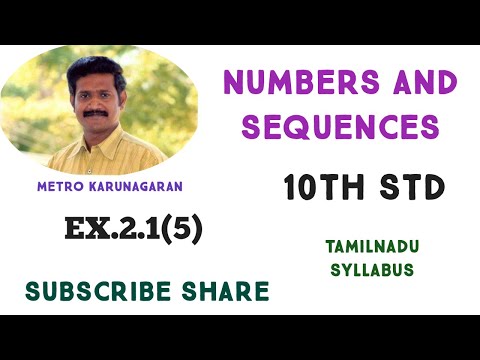
10th Std Maths Ex.2.1(5) Prove that square of any integer leaves the remainder either 0 or 1 when di
Unit 1 Pure Mathematics Trigonometry 5
Prove that the square of any positive integer is of the form 4q or 4q+1 for...|| Q.8 Ex.1.1 Class 10
Prove that sqrt(2) exists [ILIEKMATHPHYSICS]
How to prove the quadratic formula by completing squares
Prove that the square of any positive integer is of the form 5q, 5q+1, 5q+4 ...|| Q.9 Ex.1.1 Class10
Prove that the square of any positive integer of the form 5q+1 is ...|| Class 10 Ex.11 Q.6 RD SHARMA
How to prove any number irrational #shorts #mathshustle
sin square theta + cos square theta = 1 How to prove?
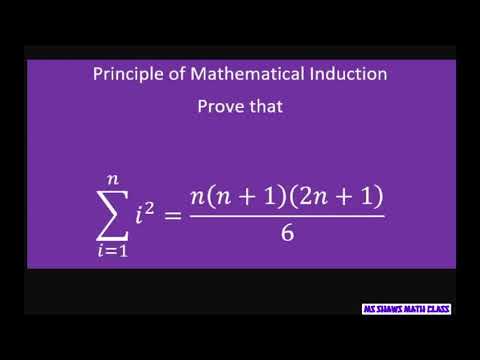
Prove by mathematical induction that the sum of squares of positive integers is n(n+1)(2n+1)/6
Why greatest Mathematicians are not trying to prove Riemann Hypothesis? || #short #terencetao #maths
Prove A Number Irrational in 5 Seconds 🔥 #shorts #ytshorts #maths #class10maths
Prove that sum of the squares of the sides of a rhombus is equal to sum of the squares of diagonals
Prove that root 2 Is a rational number #solutions #realnumbers
direct proof 1: Prove the square of an integer is an integer
Prove that, in a right-angled triangle, the square of hypotenuse is equal to the sum of the squa...
Prove that sqrt(2) + sqrt(3) Cannot be a Rational Number
Комментарии
 0:04:32
0:04:32
 0:00:46
0:00:46
 0:05:41
0:05:41
 0:02:58
0:02:58
 0:06:20
0:06:20
 0:07:04
0:07:04
 0:08:08
0:08:08
 0:06:00
0:06:00
 1:27:09
1:27:09
 0:04:42
0:04:42
 0:16:43
0:16:43
 0:04:53
0:04:53
 0:06:15
0:06:15
 0:02:22
0:02:22
 0:00:16
0:00:16
 0:02:15
0:02:15
 0:08:48
0:08:48
 0:00:38
0:00:38
 0:00:41
0:00:41
 0:06:13
0:06:13
 0:00:16
0:00:16
 0:05:32
0:05:32
 0:05:07
0:05:07
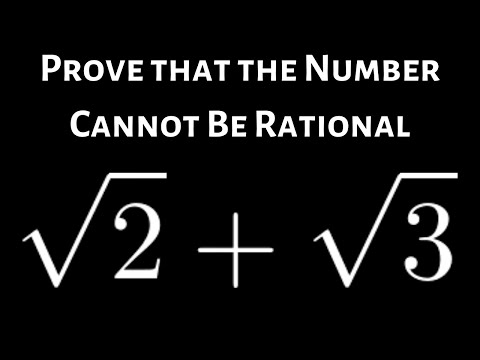 0:05:34
0:05:34