filmov
tv
Calculus 3 - Direction Cosines & Direction Angles of a Vector
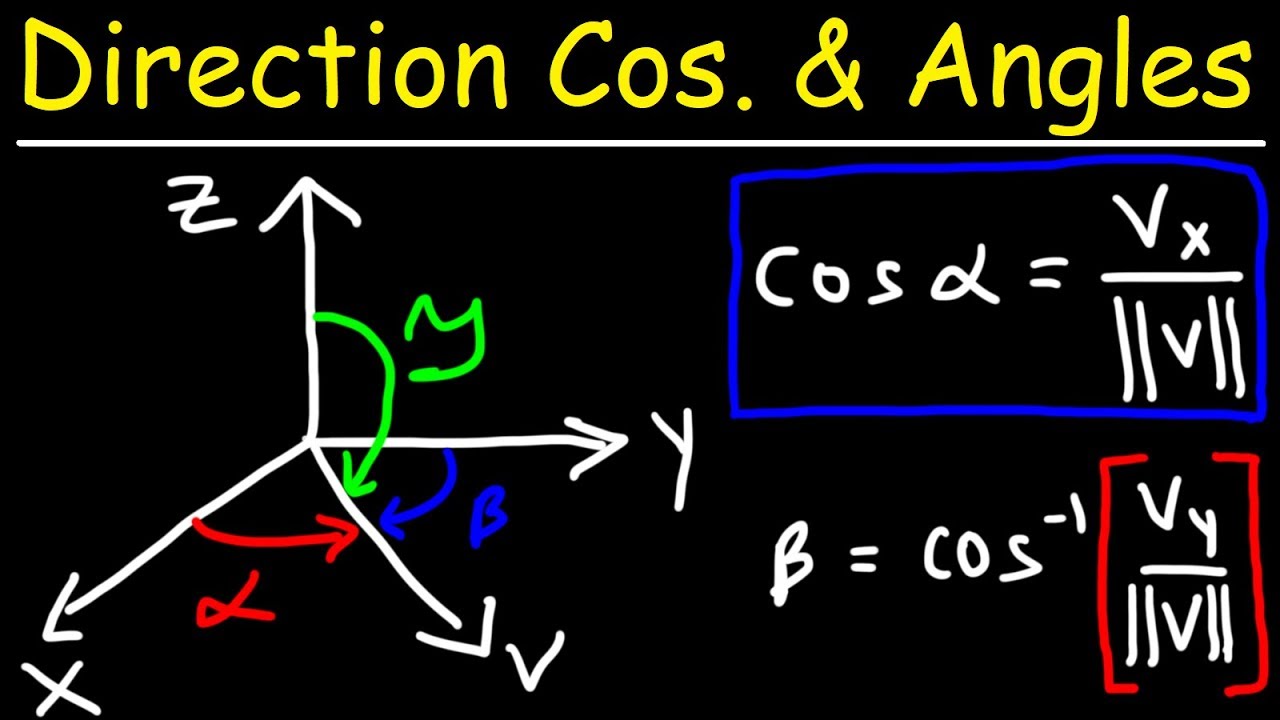
Показать описание
This calculus 3 video tutorial explains how to find the direction cosines of a vector as well as the direction angles of a vector.
3D Distance Formula:
Equation of a 3D Sphere:
Calculus 3 - Intro to Vectors:
Calculus 3 - The Dot Product:
_______________________________
Angle Between Two Vectors:
Parallel & Orthogonal Vectors:
Direction Cosines and Vectors:
Calculus 3 - Vector Projections:
Cross Product of 2 Vectors:
_______________________________
Area - Vector Cross Product:
Triple Scalar Product:
Vector Equations of Lines:
The Equation of a Plane:
Planar Equation - 3 Points:
________________________________
Final Exams and Video Playlists:
Full-Length Videos and Worksheets:
Vectors - Free Formula Sheet:
3D Distance Formula:
Equation of a 3D Sphere:
Calculus 3 - Intro to Vectors:
Calculus 3 - The Dot Product:
_______________________________
Angle Between Two Vectors:
Parallel & Orthogonal Vectors:
Direction Cosines and Vectors:
Calculus 3 - Vector Projections:
Cross Product of 2 Vectors:
_______________________________
Area - Vector Cross Product:
Triple Scalar Product:
Vector Equations of Lines:
The Equation of a Plane:
Planar Equation - 3 Points:
________________________________
Final Exams and Video Playlists:
Full-Length Videos and Worksheets:
Vectors - Free Formula Sheet:
Комментарии
 0:12:50
0:12:50
 0:05:43
0:05:43
 0:06:41
0:06:41
 0:30:21
0:30:21
 0:25:24
0:25:24
 0:07:25
0:07:25
 0:22:41
0:22:41
 0:02:41
0:02:41
 0:05:01
0:05:01
 0:13:42
0:13:42
 0:08:37
0:08:37
 0:05:14
0:05:14
 1:14:11
1:14:11
 0:17:53
0:17:53
 0:08:13
0:08:13
 0:00:05
0:00:05
 0:19:04
0:19:04
 0:07:52
0:07:52
 0:19:14
0:19:14
 0:05:07
0:05:07
 0:00:42
0:00:42
 0:11:43
0:11:43
 0:28:30
0:28:30
 0:03:33
0:03:33