filmov
tv
Unlocking Complex Numbers with Geometry: 2023 AMC12A Problem Solved!

Показать описание
🌟 Dive into the fascinating world of complex numbers with our latest video, where we tackle a challenging problem from the 2023 AMC12A exam using a unique geometric interpretation. 📐✨
In this video, we explore the intriguing problem of finding the maximum imaginary part of a complex number z under the condition
|1 + z + z^2| = 4.
Rather than relying on traditional algebraic methods, we delve into a geometric approach that simplifies the problem and offers a more visual understanding. 🎯🔍
🔑 Key Highlights:
- Transforming the equation \( |1 + z + z^2| = 4 \) into a more geometrically friendly form: \( |(z + 1/2)^2 + 3/4| = 4 \).
- Discovering how this equation represents a circle in the complex plane and using this insight to determine the maximum magnitude of \( |z^2| \).
- Step-by-step walkthrough to visually interpret complex numbers and efficiently solve the problem without getting lost in algebraic complexity.
📚 This video is perfect for students, educators, and math enthusiasts looking to deepen their understanding of complex numbers and enhance their problem-solving skills with geometric insights. Whether you're preparing for the AMC12A or just love diving into intriguing math problems, this video is for you!
👍 Don't forget to like, share, and subscribe for more exciting math content. Drop your questions and insights in the comments below - let's discuss and learn together!
#AMC12A #ComplexNumbers #GeometryInMath #MathProblemSolving #EducationalMath #MathEnthusiasts
In this video, we explore the intriguing problem of finding the maximum imaginary part of a complex number z under the condition
|1 + z + z^2| = 4.
Rather than relying on traditional algebraic methods, we delve into a geometric approach that simplifies the problem and offers a more visual understanding. 🎯🔍
🔑 Key Highlights:
- Transforming the equation \( |1 + z + z^2| = 4 \) into a more geometrically friendly form: \( |(z + 1/2)^2 + 3/4| = 4 \).
- Discovering how this equation represents a circle in the complex plane and using this insight to determine the maximum magnitude of \( |z^2| \).
- Step-by-step walkthrough to visually interpret complex numbers and efficiently solve the problem without getting lost in algebraic complexity.
📚 This video is perfect for students, educators, and math enthusiasts looking to deepen their understanding of complex numbers and enhance their problem-solving skills with geometric insights. Whether you're preparing for the AMC12A or just love diving into intriguing math problems, this video is for you!
👍 Don't forget to like, share, and subscribe for more exciting math content. Drop your questions and insights in the comments below - let's discuss and learn together!
#AMC12A #ComplexNumbers #GeometryInMath #MathProblemSolving #EducationalMath #MathEnthusiasts
Комментарии
 0:06:13
0:06:13
 0:06:34
0:06:34
 0:02:25
0:02:25
 0:00:14
0:00:14
 0:00:46
0:00:46
 0:00:14
0:00:14
 0:07:41
0:07:41
 0:45:56
0:45:56
 0:05:19
0:05:19
 0:00:55
0:00:55
 0:07:55
0:07:55
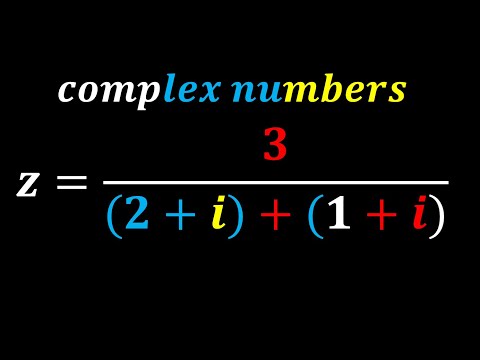 0:07:24
0:07:24
 0:00:25
0:00:25
 0:00:19
0:00:19
 0:00:22
0:00:22
 0:00:47
0:00:47
 0:03:40
0:03:40
 0:20:15
0:20:15
 0:23:02
0:23:02
 0:00:40
0:00:40
 0:00:17
0:00:17
 0:07:09
0:07:09
 0:00:13
0:00:13
 0:46:05
0:46:05