filmov
tv
Greg Moore - Quantum Field Theory And Invariants Of Smooth Four-Dimensional Manifolds

Показать описание
73rd British Mathematical Colloquium
The talk will begin with some very general remarks on the topic of "physical mathematics."
Quantum Field Theory offers an interesting perspective on many topological and geometric invariants in mathematics. In general, the path integrals of QFT have not been defined with full mathematical rigor. Nevertheless, there are well-defined rules for manipulating them, and insights from physics can lead to well-defined, and, sometimes, new and unexpected predictions about concrete mathematical quantities. These predictions are statements amenable to rigorous analysis. This talk focuses on the renowned example of Donaldson and Seiberg-Witten invariants in the theory of four-manifolds. In this case physics predicts an interesting equality between a path integral of an "ultraviolet" (UV) and an "infrared" (IR) quantum field theory. The physical prediction is that these two path integrals are exactly equal. The path integral of the UV theory localizes to an integral over the (finite-dimensional) moduli space of anti-self-dual instantons on a principal G bundle over an oriented, compact four-manifold X without boundary.
The path integral of the IR theory localizes to an integral over the (finite-dimensional, smooth, and compact) moduli space of solutions to the Seiberg-Witten equations on X.
The physical relation between the UV and IR theories allows one to derive the relation between Donaldson and Seiberg-Witten invariants for manifolds with b2+ larger than 0. They key to the analysis is a finite-dimensional integral called the "u-plane integral." The u-plane integral was analyzed, precisely for this purpose, by Moore and Witten in 1997. After reviewing that basic example the talk will - time permitting - describe several recent developments. Some of the recent developments which might be described are:
1. Deeper understanding of the role of mock modular forms,
2. Generalizations that interpolate between Donaldson and Vafa-Witten invariants,
3. Extensions to 5-dimensional super-Yang-Mills and the so-called "K-theoretic Donaldson invariants," and
4. Extensions to families of four-manifolds.
The talk will begin with some very general remarks on the topic of "physical mathematics."
Quantum Field Theory offers an interesting perspective on many topological and geometric invariants in mathematics. In general, the path integrals of QFT have not been defined with full mathematical rigor. Nevertheless, there are well-defined rules for manipulating them, and insights from physics can lead to well-defined, and, sometimes, new and unexpected predictions about concrete mathematical quantities. These predictions are statements amenable to rigorous analysis. This talk focuses on the renowned example of Donaldson and Seiberg-Witten invariants in the theory of four-manifolds. In this case physics predicts an interesting equality between a path integral of an "ultraviolet" (UV) and an "infrared" (IR) quantum field theory. The physical prediction is that these two path integrals are exactly equal. The path integral of the UV theory localizes to an integral over the (finite-dimensional) moduli space of anti-self-dual instantons on a principal G bundle over an oriented, compact four-manifold X without boundary.
The path integral of the IR theory localizes to an integral over the (finite-dimensional, smooth, and compact) moduli space of solutions to the Seiberg-Witten equations on X.
The physical relation between the UV and IR theories allows one to derive the relation between Donaldson and Seiberg-Witten invariants for manifolds with b2+ larger than 0. They key to the analysis is a finite-dimensional integral called the "u-plane integral." The u-plane integral was analyzed, precisely for this purpose, by Moore and Witten in 1997. After reviewing that basic example the talk will - time permitting - describe several recent developments. Some of the recent developments which might be described are:
1. Deeper understanding of the role of mock modular forms,
2. Generalizations that interpolate between Donaldson and Vafa-Witten invariants,
3. Extensions to 5-dimensional super-Yang-Mills and the so-called "K-theoretic Donaldson invariants," and
4. Extensions to families of four-manifolds.
Комментарии
 1:07:09
1:07:09
 1:36:31
1:36:31
 0:58:59
0:58:59
 0:16:03
0:16:03
 0:58:00
0:58:00
 0:48:08
0:48:08
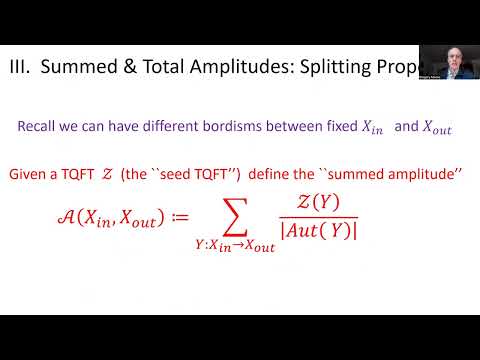 0:52:51
0:52:51
 1:38:55
1:38:55
 1:03:27
1:03:27
 1:00:47
1:00:47
 1:03:04
1:03:04
 1:14:41
1:14:41
 1:32:26
1:32:26
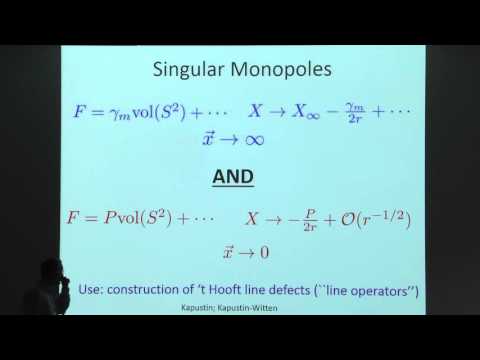 1:06:06
1:06:06
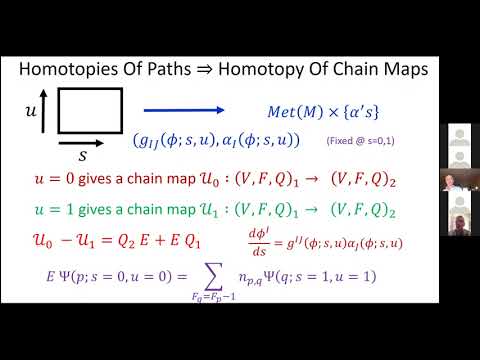 1:49:17
1:49:17
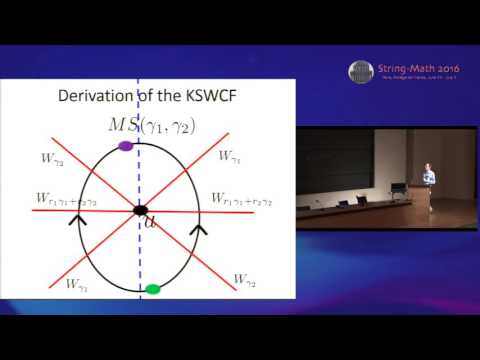 0:55:23
0:55:23
 1:21:10
1:21:10
 1:35:12
1:35:12
 1:30:05
1:30:05
 0:44:28
0:44:28
 1:30:05
1:30:05
 0:17:21
0:17:21
 0:45:00
0:45:00
 1:14:20
1:14:20