filmov
tv
The Poisson Distribution: Derivation

Показать описание
Here, we derive the Poisson distribution, which shows up in a wide variety of phenomena in science and everyday life. You might want to check out "Why the Poisson Distribution is Important (It's Everywhere!!)"
or
"A Tiny Bit of Combinatorics"
before watching this video.
or
"A Tiny Bit of Combinatorics"
before watching this video.
Proof that the Binomial Distribution tends to the Poisson Distribution
The Poisson Distribution: Mathematically Deriving the Mean and Variance
The Poisson Distribution : A Derivation
Poisson distribution derivation. Intuitive example.
The Poisson Distribution: Derivation
An Introduction to the Poisson Distribution
Poisson Distribution Derivation
Introduction to Poisson Distribution - Probability & Statistics
Mean and Variance of Poisson Distribution | Proof in easy way | Very Important
Proof Binomial to Poisson Distribution || Lesson 56 || Probability & Statistics || Learning Monk...
The Poisson Distribution: Deriving and Two Basic Examples
Poisson Distribution derivation
Binomial distributions | Probabilities of probabilities, part 1
Poisson Distribution mean,variance and MGF
The Relationship Between the Binomial and Poisson Distributions
L22.2 Definition of the Poisson Process
Deriving the Poisson distribution
Maximum Likelihood Estimation for the Poisson Distribution
Moment Generating Function of the Poisson Distribution
Poisson Distribution (Mean, Variance, MGF)
Extra Math lecture 2: The derivation of the poisson distribution
Poisson Expectation
Mean of the Poisson distribution using simple method
Derivation of Poisson Distribution
Комментарии
 0:05:25
0:05:25
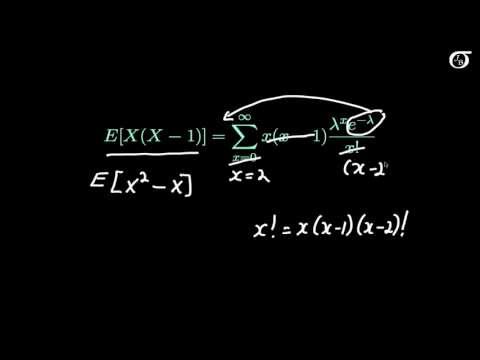 0:09:17
0:09:17
 0:14:53
0:14:53
 0:08:52
0:08:52
 0:16:25
0:16:25
 0:09:03
0:09:03
 0:14:14
0:14:14
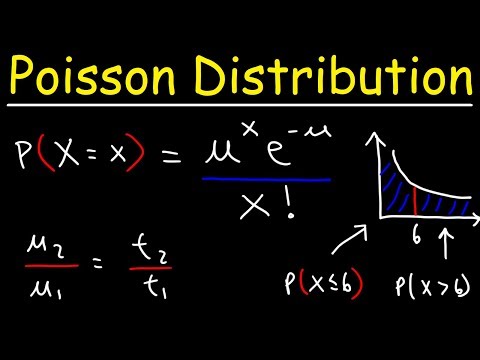 0:16:12
0:16:12
 0:08:49
0:08:49
 0:07:54
0:07:54
 0:15:21
0:15:21
 0:08:36
0:08:36
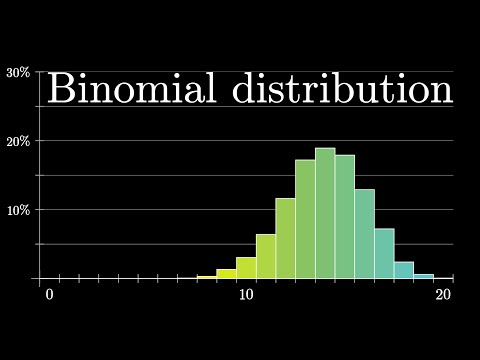 0:12:34
0:12:34
 0:14:30
0:14:30
 0:05:24
0:05:24
 0:05:07
0:05:07
 0:04:55
0:04:55
 0:16:39
0:16:39
 0:19:34
0:19:34
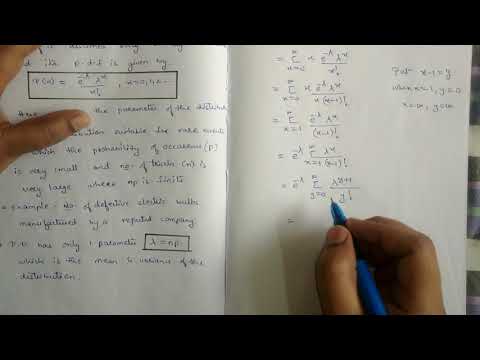 0:14:17
0:14:17
 0:12:22
0:12:22
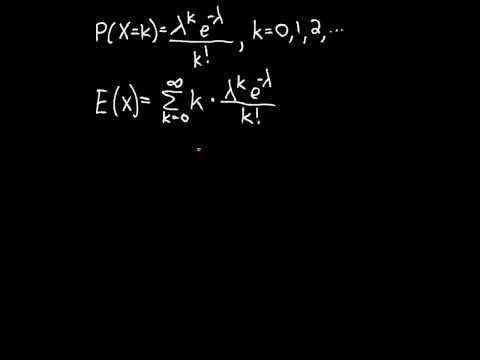 0:04:19
0:04:19
 0:03:06
0:03:06
 0:16:39
0:16:39