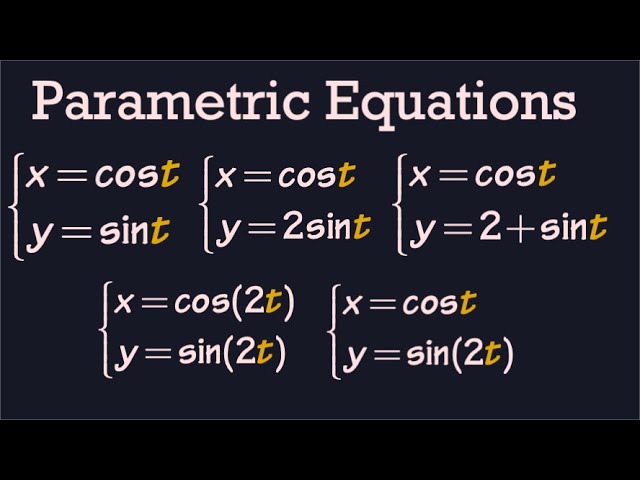
Parametric equations with sine and cosine

We will go over 5 examples of parametric equations with sine and cosine. We will see how to convert parametric equations to rectangular (aka Cartesian). And we will also graph these parametric equations.

[BigDBrian]

the loss of generality comes from the fact that sin(t) can be negative, but in the triangle you used to figure out its length, it can only be positive. I think that's worth stating.

[yurackjung]

This is so much better than my analysis teacher just giving us a ws to figure it out on our own... thanks

[StellarShowtime]

Thanks for helping with a shortcut way of converting parametric equations to rectangular via trig sub!

[jakekeene]

Nice way to wake up, you scared the shit out of me lol.

[SuperStruct]

I've been waiting for this video for so long and I just realized it now, been thinking about an Ood reference for so long

[yorkshire_tea_innit]

What do you do when cos and sin are both on the same equation. e.g.

x=(sin(t)*a)+cos(t)
y=(sin(t)*a)+sin(t)

[alexkidy]

Nice. I had some difficulty to understand the last one.The others are easy. Tks!

[jesussaquin]

That introduction should automatically be a subscription

[__-oqnb]

Yes! Just in time! We just started going over em!!! Thank you!

[iordachebeniamin]

i belive something change at 4:41 but i forgot.I have a feeling i saw something odd

[neilgerace]

The logo of the Australian Broadcasting Corporation is based on a Lissajous curve:

x = cos t;
y = sin 3t

[holyshit]

This parametrization can be rationalized, see how can we derive Euler's substitution
(Cut the curve y^2=ax^2+bx+c with secant line or
Draw bisector of angle complementary to theta in our trig substitution picture to get another right triangle
In this new triangle choose angle Pi/4+theta/2 and calculate its tangent)

[chaosredefined]

x = cos(t), y = sin(2t) = 2sin(t)cos(t) = 2sin(t)*x
x = cos(t), y/2x = sin(t)
x^2 + y^2/(4x^2) = 1
4x^4 - 4x^2 + y^2 = 0

[goose]

You're the goat, love the videos!

[richardfredlund]

so if you had x=cos t and y =sin 3t would you have a triple loop and so on? ... i haven't checked this but it seems logical. (edit: I see from other comments the answer for 3t case is yes and it is Lissajous curve)

[mjz]

sin(t) = ±sqrt(1-x^2), because sin(t) is not necessary non-negative when 0<=t<=2pi.
Therefore, the shape of plot should be like ∞.

[MrRyanroberson]

I made a function in desmos that allows for the rotation in 3-space of 3-up-projections of that last function, so all I can think of are saddles, not bow ties XD

[rizkyagungshahputra]

lim x goes to ∞ cotan(x^(1/lnx))?
i got cotan(e) but when i checked the answer in wolfram, it turned out that there is no limit because there is infinitely many singularity in every neighborhood about x=∞

[DruishQueen]

Okay here is the deal: wear the mask to class. Just give the lecture like always, and be like "what, what are you guys looking at" like totally serious and normal. And as always, that's it.

[zza]

We can make an easy theorem to be faster :
let x and y be such that:
x=φ(t), where φ is a bijection
y=ψ(t)

then φ⁻¹(x)=t

so y=ψ(φ⁻¹(x))