filmov
tv
CMO (Canadian Mathematic Olympiad) 1969, #5
Показать описание
Today's question is "In triangle ABC, CD is the bisector of the angle ACB, prove that (2ab x cos(c/2))/(a+b)=d"-- CMO 1969, #5
Please comment below if you have any questions or any other math problems you would like to share!
The Questions Anna Came Across
I am 14 and I hope I can help more people with math and I hope more people like math!!
Anna Muyan Li
Please comment below if you have any questions or any other math problems you would like to share!
The Questions Anna Came Across
I am 14 and I hope I can help more people with math and I hope more people like math!!
Anna Muyan Li
0:02:43
CMO (Canadian Mathematic Olympiad) 1969, #5
0:13:53
hard Canadian mathematical Olympiad 1969
0:01:38
Olympiad Right Triangle Inequality (Canada 1969 - visual proof)
0:10:50
hard canadian Mathematical Olympiad
0:06:43
Canadian Math Olympiad Q1 Solution - Contradict the Contradiction
0:12:56
Canadian Mathematical Olympiad, problem 1, 2018 - part 1
0:04:37
canadian Mathematical Olympiad
0:30:50
COMC Practice Problems -- Week 01 Prob B -- 2019
0:10:17
Canadian Mathematical Olympiad, 2018, problem 1 - part 2
0:25:15
COMC Practice Problems -- Week 01 Prob A -- 2019
0:06:26
Canadian mathematical Olympiad
0:42:44
COMC Practice Problems -- Week 06 -- 2019
1:00:18
COMC Practice Problems -- Week 02 -- 2019
0:31:06
COMC Practice Problems -- Week 04 -- 2019
0:46:02
COMC Practice Problems -- Week 07 -- 2019
0:33:44
COMC Practice Problems -- Week 03 -- 2019
1:03:52
COMC Practice Problems -- Week 05 -- 2019
0:02:21
Top 20 Country by International Mathematical Olympiad Gold Medal (1959-2019)
0:17:56
A Physics Prof Bet Me $10,000 I'm Wrong
0:10:18
Number of integral solutions (Interesting application of Combinatorics)
0:11:07
MATH299B Video #1 - Functional Equation from Putnam Exam
0:09:03
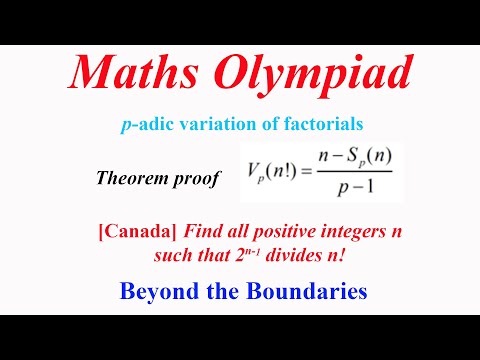
Maths Olympiad: Number theory: p-adic variation of n! formula proof Vp(n!)=n-Sp(n)/p-1 with example
0:05:23
INTERNATIONAL MATHEMATICAL OLYMPIAD 1961
0:03:38
Zeller's Congruence | The Day of the Week
Комментарии