The Problem No Americans Get Right (Though Russians Do) // Math Minute [#51] [NUMBER GEOMETRY]

The esteemed mathematician V.I. Arnold authored a book on problem solving that included the following problem:

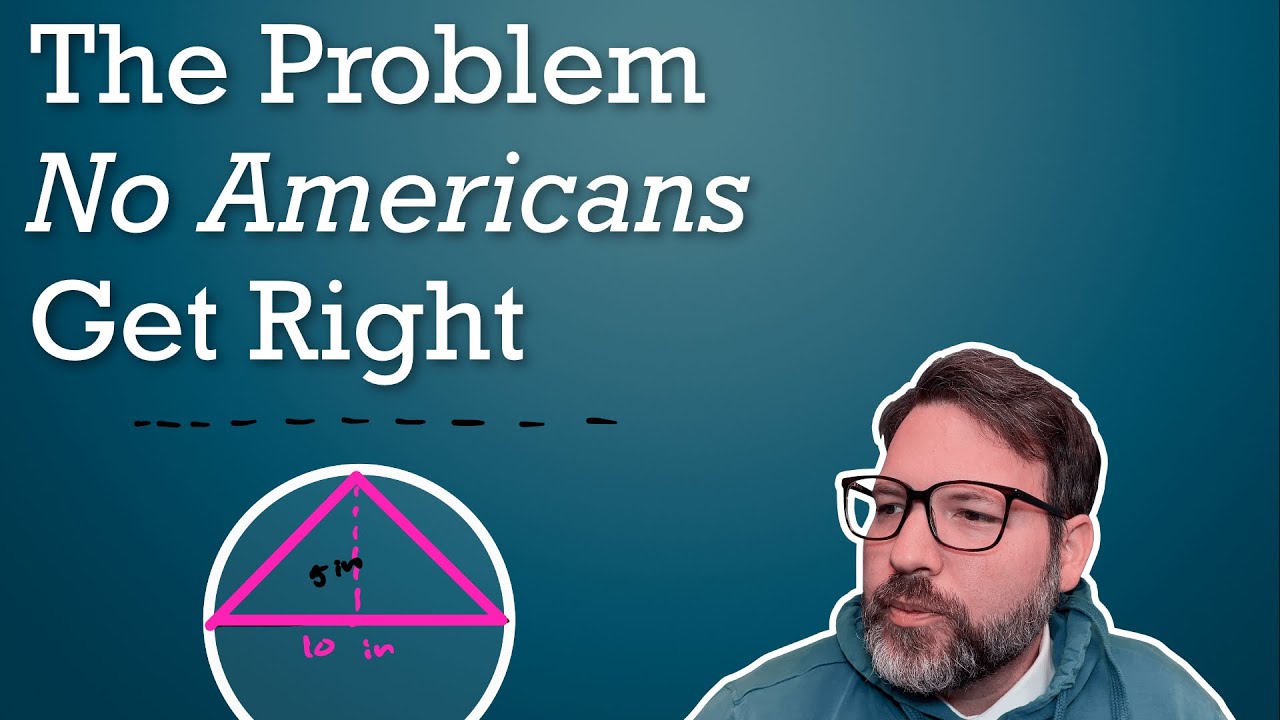
"The hypotenuse of a right-angled triangle (in a standard American examination) is 10 inches, the altitude dropped onto it is 6 inches. Find the area of the triangle."

"American school students had been coping successfully with this problem over a decade. But then Russian school students arrived from Moscow, and none of them was able to solve it as had their American peers (giving 30 square inches as the answer). Why?"

Notice, the problem is not actually about finding the area of the triangle, but explaining the discrepancy between the (so-called) American calculation and the work of their counterpart Russian students. It's a beautiful bit of mathematical problem-solving that really rewards attention to the circumstances of the problem.

Follow Tim Ricchuiti:

Watch more Math Videos:

––––––––––––––––––––––––––––––
Track: Rainy Paradise — Next Route & WOMA [Audio Library Release]
Music provided by Audio Library Plus
––––––––––––––––––––––––––––––

[shellfamily]

I just now found the math minute Playlist. Thx.

[shellfamily]

As a k-12 teacher, I tune in for k-12 content. A playlist of math minutes and math tricks for k-12 students/teachers maybe? I don't know how hard that would be.

[andreizhitkov]

6x8x10 solution would not be viable for the Russians, since the 'altitude' means to them a line perpendicular to the hypotenuse.

[shellfamily]

Does the original statement of the problem indicate the hypotenuse is the diameter?

[KevnReid]

Is anyone else listening to this and wondering what the story is behind the question? Who are these American and Russian students? Does this take place at a particular school? What is the context! Also, how the teaching that each group of students receive differ to produce these results?

...or was the whole scenario just made up to make Russian students look good and American students look bad?

[iso]

hey what type of math did you major in college???

[PubicGore]

Small corrections made here. Also, it is worth noting that every triangle has a unique circumcircle.

Also, since it was not mentioned, the argument used here is a corollary of Thales' theorem, which is a special case of the inscribed angle theorem. I will now state Thales' theorem:

If A, B, and C are distinct points on a circle wherein AC is its diameter, then angle ABC is a right angle.

The rest is just my nitpicking, generalization, and two other proofs that such a triangle is impossible.

At 1:33 you contradicted yourself. You said "what is the greatest distance away that third corner (vertex) of the right triangle can be?" However, you should have said: "what is the shortest altitude this triangle can have?" (Note that for any given triangle, the longest altitude is always perpendicular to the shortest side of the triangle. Since we are considering the altitude of the hypotenuse--the longest side--we know that this its altitude will be the triangle's shortest.) The reason you should have said this is because you failed to specify what you are considering when talking about how far away that point B is. In this case, you meant the hypotenuse so that this distance would always be an altitude. Shortly after. you say: "In other words, what is the greatest altitude possible?" However, that is very different than what you had said previously.

Moreover, we can generalize your argument. At 1:43, we need not consider an isosceles triangle. Why? Consider a triangle ABC wherein AC is the diameter of its circumcircle. Let O be the midpoint of A and C. Let P be the foot of the perpendicular bisector of AC from B. Notice that PB is an altitude of ABC, and is perpendicular to the hypotenuse AC. Now notice that |OB|≥|PB| (notice that equality holds if and only if OB=PB, which is what you considered in your argument). Finally, notice that OB is a radius of the circumcircle, thus |OB|=|AC|/2. In our problem, notice that |PB|=6, and |AC|=10.Then we have that |AC|/2=5=|OB|. Recall that |OB|≥|PB|, thus 5≥6, a clear contradiction, so such a triangle cannot exist.

Another elementary argument involves the geometric mean theorem. Consider a triangle ABC wherein AC is the diameter of its circumcircle. Let P be the foot of the perpendicular bisector of AC from B. Notice that by the geometric mean theorem, we have |PB|=sqrt(|AP|*|PC|). Notice that by construction |AP|+|PC|=|AC|=10, and |AP|, |PC| are both nonnegative. According to the question |PB|=6. Thus 6=sqrt(|AP|*|PC|). Therefore we know that |AP|*|PC|=36. Let x=AP, and y=PC, then we have the following system of equations: x+y=10, xy=36. Notice that this system of equations has no real solutions, thus such a triangle cannot exist.

Here, I will prove that the aforementioned system of nonlinear equations has no real solutions.

Recall: x+y=10
<=> x=10-y.
Recall: xy=36.
<=>x=36/y.
Thus: 36/y=10-y.
<=> 36=10y-y^2
<=>-y^2+10y-36=0
Let f(x)=-x^2+10x-36.
Consider the discriminant of f(x), that is Notice that 144>100, thus 100-144<0, thus sqrt(10^2-4(-1)(-36)) is not a real number; therefore y^2+10y-36 is nonzero for all real numbers y.

Yet another elementary argument involves Pythagorean triples, although this is an elementary number theory argument, so it may be a bit esoteric (although I will not use any non-rudimentary notions of elementary number theory, so any definitions the reader might need to look up will not require specialized knowledge). Consider a triangle with hypotenuse 10, and another side length 6. Notice that there is a Pythagorean triple (6, x, 10), that details the side lengths of this triangle. It is trivial to demonstrate that x=8 by the definition of a Pythagorean triple. Notice that gcd(6, 8, 10)=2, so it is not a primitive Pythagorean triple, let us consider the primitive Pythagorean triple for which the element (6, 8, 10) over the vector space R^3 is a scalar multiple. Recall that if we have a Pythagorean triple (a', b', c') such that gcd(a', b', c')=d, then the primitive Pythagorean triple of which (a', b', c') is a scalar multiple is (a, b, c), where a=a'/d, b=b'/b, and c=c'/d. In other words, (a', b', c')=d(a, b, c). In our case notice that (6, 8, 10)=2(3, 4, 5), so the primitive Pythagorean triple (3, 4, 5) together with a scalar multiple k will generate all natural number tuples satisfying the Diophantine equation x^2+y^2=z^2, which will in turn generate all triangles with natural number side lengths that are similar to the 3-4-5 right triangle, and all such triangles will have side lengths 3k, 4k, and 5k. Now consider the altitude of a 3-4-5 triangle dropped onto the hypotenuse, let its side length be x. Then a new Pythagorean triple (x, y, 3) is generated. Thus we have x^2+y^2=3. Since x and y are both natural numbers, we have that x<3. This same logic applies to all similar triangles by (kx)^2+(ky)^2=9k^2, thus kx<3k, and so since k is a natural number x<3k. In our case k=2, so we have that the altitude x<3(2)=6. So this altitude has a length less than 6, thus the triangle cannot exist. Also, in this argument, we have proven that it is impossible for all similar triangles.