Degree Vs Radian in Angle Measurement

The video clearly demonstrates the Degree and Radian in Angle Measurement.

From Quora, some suggestions.

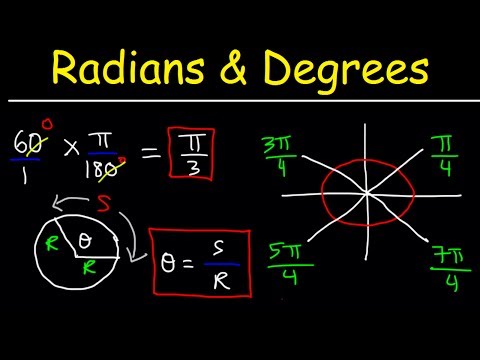
Radian describes the plane angle subtended by an arc s, as the length of the arc divided by the radius r, of the arc. Thus
s=rθ, where θ in radians.
But we consider angular measurements in degrees in common use. It is one of the angular measurements defined so that a full rotation is 360 degrees.
θ (radian) = (π/180)* θ (degree) .
Either radian our degree can be used, depending upon the type of problem. But the use of radian measures is preferred as it is the SI unit of plane angle, and is used in advanced scientific calculations.

Radian by definition is the angle subtended by an arc whose length is equal to the radius of the circle. This unit has a physical relevance.
Whereas degree has no such direct physical relevance, the complete angle of the circle is divided into 360 degrees. Nothing more.
As per usage, its a matter of convenience and intuitiveness of use.

Radians are the natural unit. The ratio of a circle’s circumference to its diameter is π, so the ratio of its circumference to its radius is 2π. Defining “angle” as the ratio of the arc subtended by the angle to the arc’s radius simplifies all later mathematical manipulations. That’s why radians are used.

What is the relation between radian and degree?
Both degree and radian are used to measure angles. They have wide applications; therefore, it is crucial to understand the relationship between degree and radian. Furthermore, we need to understand how degree and radian values are derived from understanding their relationship.



Circumference of a circle = 2πr

(And a circle is divided into 360 equal parts = 360° = 2πr

and one part is equal to 1°,so,360° is

equivalent to its circumference)

= 180° = πr

( Now, radian is equal to the length of the radius = 180° = π × Radian

here “r” in the equation.) = 180° / π = Radian



=180° / 3.14 = Radian

=57.33° = Radian.

Now that it is clear from the above equation how radians and degrees are interconnected, it is vital to understand how to convert degrees into radians and vice versa.

Degree in radian
A full revolution of a circle denotes 360°, therefore

= 360° = 2π × radian

= 1° = 2π×radian / 360°

= 1° = π / 180°×radian

Now, the degree value can be converted into radian by multiplying the value by π / 180°.

For example,

The value of 30° is equal to

=30 × π / 180°

= π/6 radian

Radian to degree
As,

Circumference of a circle = 2πr

(And a circle is divided into 360 equal parts = 360° = 2πr

and one part is equal to 1°,so,360° is equivalent to its circumference)

= 180° = πr

(Now, radian is equal to the length of the radius = 180° = π × Radian here “r” in the equation.) = 180° / π = Radian

The value of radian can be converted in degrees by multiplying 180° / π.

For example,

π/6 radian = π/6 × 180° / π

=180°/6

= 30°.