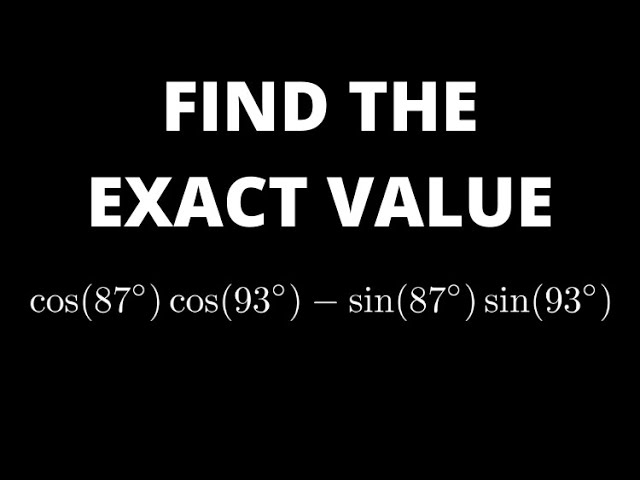
Find the Exact Value using the Sum and Difference Identities

Find the Exact Value using the Sum and Difference Identities

If you enjoyed this video please consider liking, sharing, and subscribing.

There are several ways that you can help support my channel:)

************Udemy Courses(Please Use These Links If You Sign Up!)*************
Abstract Algebra Course

Advanced Calculus Course

Calculus 1 Course

Calculus 2 Course

Calculus 3 Course

Calculus 1 Lectures with Assignments and a Final Exam

Calculus Integration Insanity

Differential Equations Course

College Algebra Course

How to Write Proofs with Sets Course

How to Write Proofs with Functions Course

Trigonometry 1 Course

Statistics with StatCrunch Course

Math Graduate Programs, Applying, Advice, Motivation

Daily Devotionals for Motivation with The Math Sorcerer

Thank you:)

[josephtraverso]

I tried finding another way without using the identity…
using:
Cos(87) = Sin(3)
Cos(93) = -Sin(3)

Sin(87) = Cos(3)
Sin(93) = Cos(3)

We now obtain:

-Sin^2(3) - Cos^2(3)

We recognize the equivalent 1 and factor it out to get -1


You can also generalize this result. In this case, the offset from 90 is 3 degrees but let’s denote it by x.



-Cos^2(90+x) - Sin^2(90+x)
= -1

I love your channel so much, thank you for making me happy with each video ❤️

[cipherbenchmarks]

What tablet you use for writing on pc?

[Quartzite]

Using the identity Cos(x)cos(y)- sin(x)sin(y) = Cos(x+y)