filmov
tv
Fold the AM-GM inequality

Показать описание
This is a short, animated visual proof demonstrating the arithmetic mean geometric mean inequality using paper folding.
Thanks!
For other visual proofs of this fact see:
This animation is based on a visual proof by Yukio Kobayashi from the 2002 issue of the Mathematical Gazette (page 293).
#manim #shorts #mathshorts #mathvideo #math #mathematics #amgminequality #mtbos #animation #theorem #pww #proofwithoutwords #visualproof #proof #iteachmath #calculus #inequality
To learn more about animating with manim, check out:
Thanks!
For other visual proofs of this fact see:
This animation is based on a visual proof by Yukio Kobayashi from the 2002 issue of the Mathematical Gazette (page 293).
#manim #shorts #mathshorts #mathvideo #math #mathematics #amgminequality #mtbos #animation #theorem #pww #proofwithoutwords #visualproof #proof #iteachmath #calculus #inequality
To learn more about animating with manim, check out:
Fold the AM-GM inequality
Folding the AM-GM Inequality V (quick visual proof)
AM-GM Inequality VI (visual proof)
AM-GM Inequality IV (visual proof)
AM-GM Inequality III (visual proof)
Math Induction Trick RARELY taught in class!
Arithmetic Mean-Geometric Mean Visual Proof Compilation
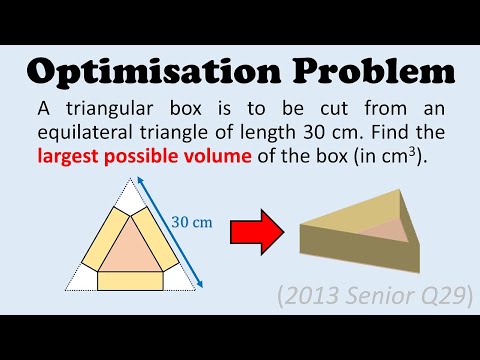
2 Methods to solve an Olympiad Optimisation Problem
RMS 8.8 Solving multi step equat inequalities
ALGEBRAIC & GEOMETRIC multiplicity (AM & GM) |Eigen values and eigen vectors |Dr Maths
Geometric Means G M P0-4 #jeeadvanced #EnrichDigitalAcademy
Bro’s hacking life 😭🤣
Euler Inequality || Geometry || ISI Entrance TOMATO 440
11 years later ❤️ @shrads
Inequality and Its Measurement
Sum of Squares and Cauchy Schwarz || Math Olympiad
Point Addition on Elliptic Curve (not tangent)
Math proof by picture #shorts
NYNTS - September 30, 2021 - Mel Nathanson - Egyptian fractions and Muirhead's inequality
What is the largest cone you can fold from a circular sector?
Which is Larger: Four Lunes vs. Inscribed Square (visual proof)
Solving the hardest question of a British Mathematical Olympiad
3 is less than 2 [Bogus Proofs]
Beautiful Fibonacci problem from Putnam 2021 Math Competition! (B1, B2, B4)
Комментарии
 0:00:54
0:00:54
 0:00:56
0:00:56
 0:02:16
0:02:16
 0:01:56
0:01:56
 0:01:45
0:01:45
 0:11:07
0:11:07
 0:05:01
0:05:01
 0:06:32
0:06:32
 0:08:56
0:08:56
 0:05:37
0:05:37
 0:27:11
0:27:11
 0:00:20
0:00:20
 0:06:12
0:06:12
 0:00:11
0:00:11
 0:53:54
0:53:54
 0:09:32
0:09:32
 0:00:25
0:00:25
 0:00:26
0:00:26
 1:08:16
1:08:16
 0:04:28
0:04:28
 0:01:56
0:01:56
 0:11:26
0:11:26
 0:02:42
0:02:42
 0:23:54
0:23:54