filmov
tv
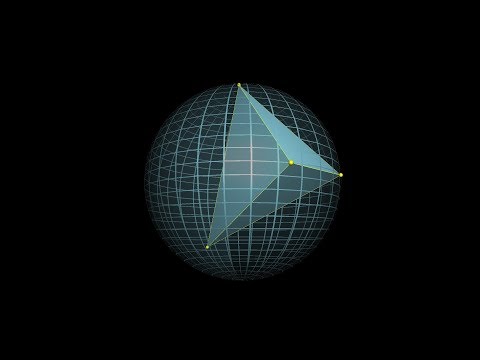
A very interesting Putnam problem

Показать описание
A casual yet satisfying Putnam problem.
My complex analysis lectures:
Support my work and get early access to content via Patreon:
You can follow me on Instagram for write ups that come in handy for my videos and DM me in case you need math help:
My LinkedIn:
Advanced MathWear:
#math #putnam #competition #numbertheory
My complex analysis lectures:
Support my work and get early access to content via Patreon:
You can follow me on Instagram for write ups that come in handy for my videos and DM me in case you need math help:
My LinkedIn:
Advanced MathWear:
#math #putnam #competition #numbertheory
A very interesting Putnam problem
The hardest problem on the hardest test
An Ultraconvex Putnam Problem.
An Interesting Substitution | Putnam 1980 A3
A 1957 Putnam exam problem
Putnam 1989, B2; An Abstract Algebra Putnam Math Competition Problem
Putnam 2023, B1; Interesting Combinatorics Problem from the 2023 Putnam Math Competition.
Cracking Putnam problem with ease. With @letsthinkcritically
An Unintuitive Solution To A Beautiful Putnam Problem
Putnam 2023, A3; A Very Challenging Putnam Calculus Problem!
An awesome Putnam problem with a number theory twist!
(1985) Putnam Mathematical Problem
Positioned as the hardest question on a Putnam exam (#6, 1992)
The Easiest Putnam Problem ever | Putnam B1 1988
Complex Putnam Problem
An Inequality Helps Solve this Problem Very Quickly | Putnam 2005 B2
The Hardest Problem on the Hardest Math exam? | Putnam B6 2016
Putnam Exam | 2018: A1
2017 Raytheon MATHCOUNTS National Competition
Power Series of a Rational Number - 2017 Putnam problem B3
An Infinite Sum that Will Leave You Speechless: Tackling a Putnam Problem
When mathematicians get bored (ep1)
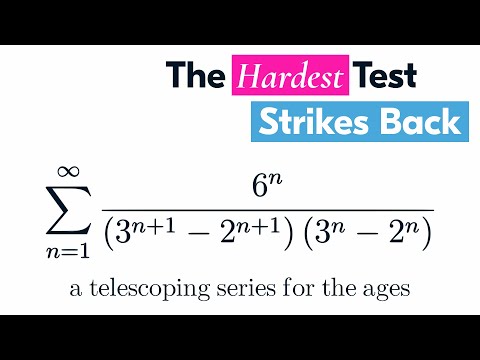
The hardest test had this 'easy' question with a surprising answer
An Easy Real Analysis Problem from the Putnam Exam
Комментарии
 0:09:07
0:09:07
 0:11:15
0:11:15
 0:26:46
0:26:46
 0:05:33
0:05:33
 0:08:27
0:08:27
 0:07:12
0:07:12
 0:05:45
0:05:45
 0:02:56
0:02:56
 0:11:54
0:11:54
 0:13:43
0:13:43
 0:08:53
0:08:53
 0:05:36
0:05:36
 0:01:00
0:01:00
 0:06:01
0:06:01
 0:08:10
0:08:10
 0:16:08
0:16:08
 0:07:51
0:07:51
 0:08:47
0:08:47
 0:56:01
0:56:01
 0:16:45
0:16:45
 0:21:54
0:21:54
 0:00:37
0:00:37
 0:07:22
0:07:22
 0:06:00
0:06:00