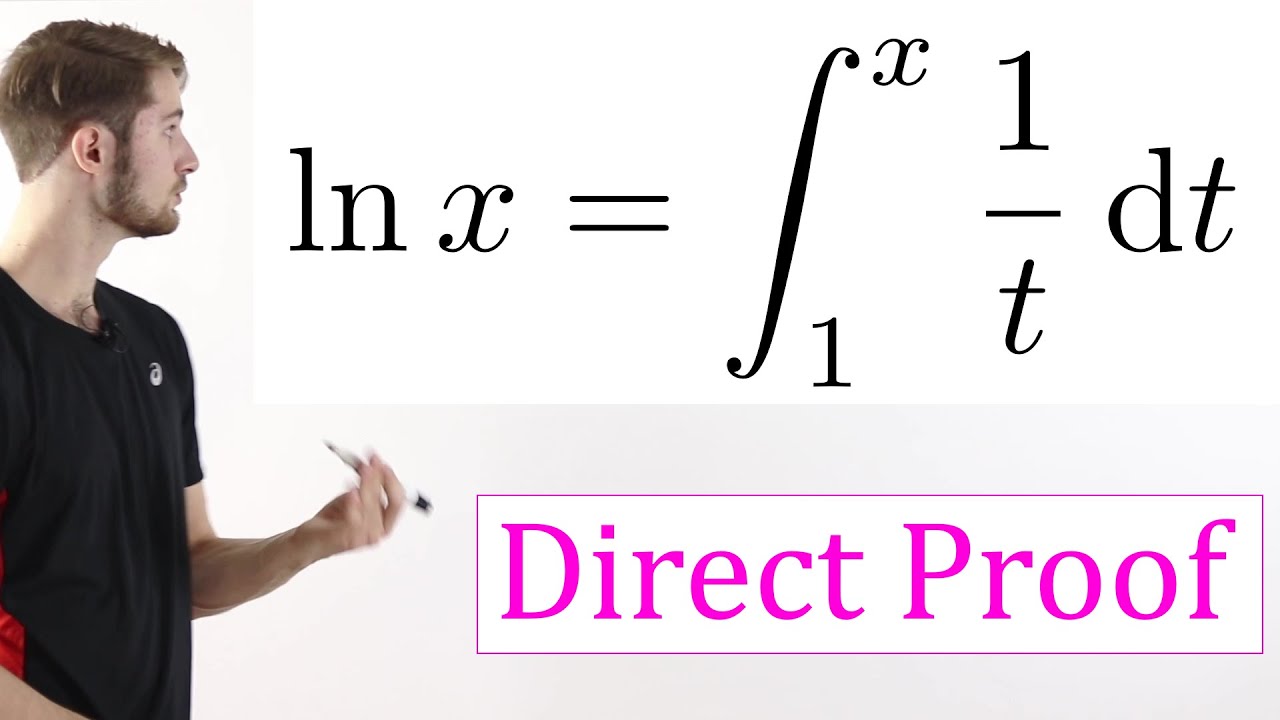
Integral Formula for Natural Log (without knowing the derivative)

This video proves that the natural log equals the integral from 1 to x of 1/t dt under the assumption that ln(x) is the inverse function to the exponential e^x. We can do this without already knowing the derivative of the natural log!

More details on why the integral is the inverse of e^x:
We proved in the video that any right inverse to e^x must equal that integral. However, we didn't prove that e^x has a right inverse in the first place.
We know that e^x : R → R+ is a strictly increasing function whose output can be made arbitrarily large or arbitrarily small. Therefore e^x is a bijection R → R+. Every bijective function has a two-sided inverse (see [1] below). Therefore e^x has a two-sided inverse, which in particular is a right inverse. I proved in video [2] that injective functions have at most one right inverse. Therefore the right inverse to e^x is unique if it exists. But we already know that there exists one right inverse that is also a two-sided inverse. We conclude that there exists exactly one right inverse to e^x and that this right inverse is also a two-sided inverse. Hence the integral in the video is a two-sided inverse to e^x.

Subscribe to see more new math videos!

Music: OcularNebula - The Lopez

[MuPrimeMath]

If you’re interested in complex analysis:

In this video I assumed that x is a real number. But we can also use this argument to define log functions

log z = ∫ᵧ 1/z dz

in the complex plane because the same derivative argument applies for complex numbers. This is very important because it lets us define log functions that are holomorphic on certain subsets of the complex plane!

[ruud]

Hey, you're back! That's good. I like your approach to math.

[Asterism_Desmos]

I like these compressed integrals for some reason, it’s so simple and elegant.

[kingbeauregard]

Glad you're back; the beard's looking righteous!

[BuddyNovinski]

Bravo, Haydn! Early birthday wishes, and I don't mean William McKinley's. I wish you'd been around a half century ago so I would've had you for my professor around 1976-7. I'm back to flood out my frustrations, for I should be good in math. I scored quite high about a half century ago in aptitude tests, and I've always wondered what had happened to my skill. Seven years ago, I wrote on my LinkedIn the major reasons we don't do well in math, and you prove at least the theory that the professors don't teach it well. By the way, the beard looks good now that the acne has healed . My acne worsened when I was your age.

[robshaw]

You’re back — you must be in grad skool by now, right?

[evplayspiano]

at 3:40, why can't we just use the idea that since the two sides have the same base, the exponents [f(1) and 0] have to be equal?

[MichaelRothwell]

How do we know that f, defined as the right inverse of eˣ, is differentiable?

[baobin]

-without knowing the derivative
- 1:13, takes the derivative

[matthewjames]

What would a "left inverse" of e^x be?

[dr.rahulgupta]

Sir, To integrate 1/ t wrt t , put t = e^x or dt = e^ x . dx and you get the result.

[vibsc]

I am a simple boy
I will differentiate both sides
So LHS=RHS
Because 1/x=derivative of upper limit is 1 and 1*1/x
Putting upper limit inyo value of t
And value of lower limit derivative is 0
Therefore 1/x=1/x hence proved haha