filmov
tv
Start Learning Numbers - Part 1 - Natural Numbers (in Set Theory)

Показать описание
Thanks to all supporters! They are mentioned in the credits of the video :)
This is my video series about Start Learning Numbers. I hope that it will help everyone who wants to learn about the construction of numbers. This is something one needs as a good foundation for all advanced mathematics.
#StartLearningMathematics
#Mathematics
#LearnMath
#calculus
x
I hope that this helps students, pupils and others. Have fun!
(This explanation fits to lectures for students in their first and second year of study: Mathematics for physicists, Mathematics for the natural science, Mathematics for engineers and so on)
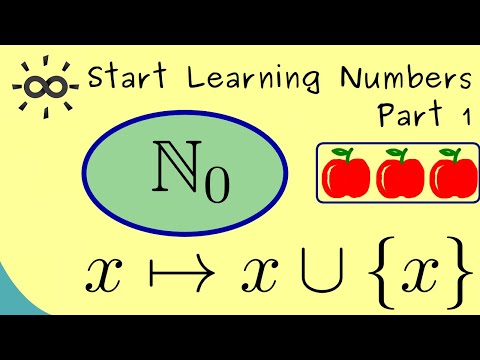
Start Learning Numbers - Part 1 - Natural Numbers (in Set Theory)
Start Learning Numbers - Part 1 - Natural Numbers (in Set Theory) [dark version]
Start Learning Numbers - Part 9 - Rational Numbers (Construction)
Start Learning Numbers - Part 3 - Natural Numbers (Induction and Associativity)
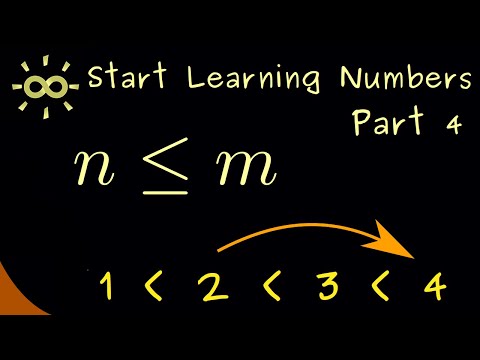
Start Learning Numbers - Part 4 - Natural Numbers (Ordering)
Start Learning Numbers - Part 7 - Integers (Addition and Inverses)
Start Learning Numbers - Part 3 - Natural Numbers (Induction and Associativity) [dark version]
Start Learning Numbers - Part 10 - Rational Numbers (Addition and Multiplication)
123 song | learn numbers | count to 10 | numbers rhymes in english for preschool #fun @Numberblocks
Start Learning Numbers - Part 5 - Natural Numbers (Multiplication)
Start Learning Numbers - Part 11 - Rational Numbers (Ordering)
Start Learning Numbers - Part 4 - Natural Numbers (Ordering) [dark version]
Start Learning Numbers - Part 6 - Integers (Construction)
Start Learning Numbers - Part 7 - Integers (Addition and Inverses) [dark version]
Start Learning Numbers - Part 11 - Rational Numbers (Ordering) [dark version]
Start Learning Numbers - Part 8 - Integers (Multiplication)
Start Learning Complex Numbers - Part 1 - Introduction
Learn German | Numbers (Part 1) | Zahlen | German for beginners | A1 - Lesson 3
Start Learning Numbers - Part 5 - Natural Numbers (Multiplication) [dark version]
Learn numbers 1-10 with Vlad & Niki and baby Chris
Learn Filipino - Filipino in Three Minutes - Numbers Part 1
Start Learning Complex Numbers - Part 3 - Absolute Value, Conjugate, Argument
Snowball Prime Numbers #shorts
Learn Numbers up to 20 for Preschool and Kindergarten | Counting for Kids | Kids Academy
Комментарии
 0:07:00
0:07:00
 0:06:47
0:06:47
 0:07:04
0:07:04
 0:09:29
0:09:29
 0:05:26
0:05:26
 0:09:46
0:09:46
 0:09:15
0:09:15
 0:07:53
0:07:53
 0:02:49
0:02:49
 0:08:08
0:08:08
 0:06:09
0:06:09
 0:05:12
0:05:12
 0:10:30
0:10:30
 0:09:33
0:09:33
 0:05:56
0:05:56
 0:04:34
0:04:34
 0:06:19
0:06:19
 0:06:58
0:06:58
 0:07:54
0:07:54
 0:06:13
0:06:13
 0:04:41
0:04:41
 0:11:41
0:11:41
 0:00:41
0:00:41
 0:15:03
0:15:03