filmov
tv
Lissajous Figures Explained | SHM | A question from JEE Main 2018 | JEE Physics | IIT JEE

Показать описание
Lissajous figure, also called Bowditch Curve, pattern produced by the intersection of two sinusoidal curves the axes of which are at right angles to each other
In JEE Main 2018 a question was asked on Lissajous Figures in Physics. It is a concept of Superposition of SHM.
Question
Two simple harmonic motions, as shown below, are at right angles. They are combined to form Lissajous figures. x(t) =A sin (at+δ) y(t) =B sin (bt) Identify the correct match below. Parameters Curve
#LissajousFigures #PhDSeries #Eduniti #JEEMain #IITJEE #JEEAdvanced #JEE2022 #JEE2023 #JEEMain2022 #SHM
#Physics #IITJEEPhysics #Class11
Lissajous Figures Explained | SHM | A question from JEE Main 2018 | JEE Physics | IIT JEE
SHM| RARE CONCEPT | ORIGINAL CHALLENGE | LISSAJOUS FIGURE | MYSTERY UNRAVELLED | LUCID EXPLANATION
Discover the Mesmerizing Le Joujou Figures
Lissajous Figure | Theory And Derivation | Simplest Language
Superposition of Oscillations : Lissajous Figures
Sand pendulums - Lissajous patterns - part one // Homemade Science with Bruce Yeany
Lissajous Figures/Bowditch Curve -5 Simple Harmonic Motion
lissajous figures simple harmonic motion
Understanding Oscilloscopes – Lissajous Patterns
Lissajous figure
Lissajous figures Derivation with explanation For Bsc 1st year physics | Bsc most important topic
Lissajous figure Meaning
Lissajous Figure- Graphical Method। BSc Physics। Two Simple Harmonic Oscillations Superposition
Lissagous Figures | Combination of Simple Harmonic Motions
Combination of SHM | Lissajous figures | SHM
Lissajous Figures : Part - 7
Lissajous Figures / BS Phys Sem-I / Waves, Oscillations and Optics / Oscillations / Chapter 01
Lissajous Curve Table animation | Manim Animations
Math Animation: Lissajous Figure, SHM, Iteration
lissajous figure
SHM || Superposition of two perpendicular SHM || Lissajous figures
Lissajous Figure- Graphical Method। BSc Physics। Two Simple Harmonic Oscillation Frequency Ratio 1:2...
Lissajous Figures in the Sand -- xmdemo 050
Lissajous figures in different modes
Комментарии
 0:10:12
0:10:12
 0:17:55
0:17:55
 0:17:48
0:17:48
 0:19:37
0:19:37
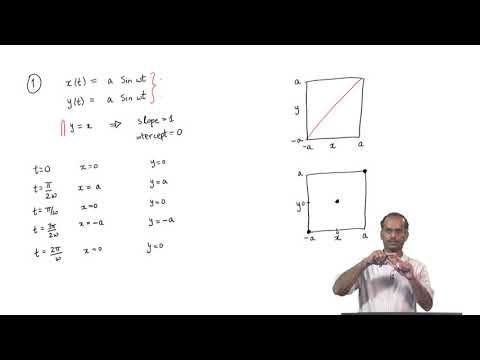 0:37:09
0:37:09
 0:09:52
0:09:52
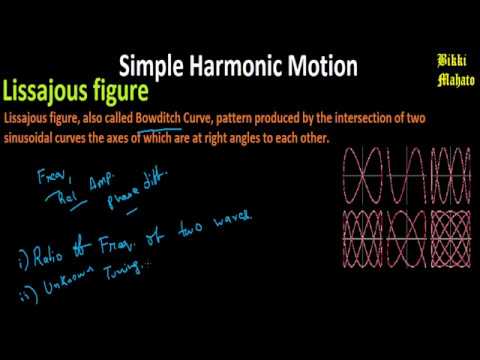 0:02:30
0:02:30
 0:30:44
0:30:44
 0:10:06
0:10:06
 0:00:53
0:00:53
 0:16:41
0:16:41
 0:00:38
0:00:38
 0:25:37
0:25:37
 0:12:09
0:12:09
 0:18:32
0:18:32
 0:04:59
0:04:59
 0:17:38
0:17:38
 0:01:31
0:01:31
 0:02:12
0:02:12
 0:00:50
0:00:50
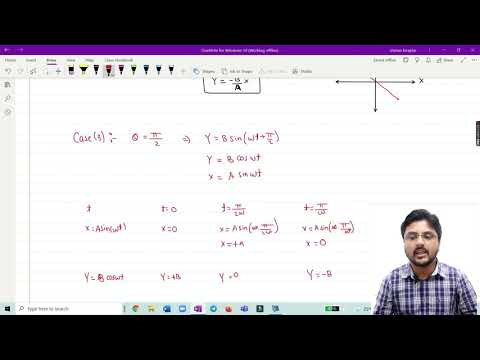 0:22:47
0:22:47
 0:29:24
0:29:24
 0:03:43
0:03:43
 0:00:20
0:00:20