filmov
tv
Applying the recursive formula to a geometric sequence
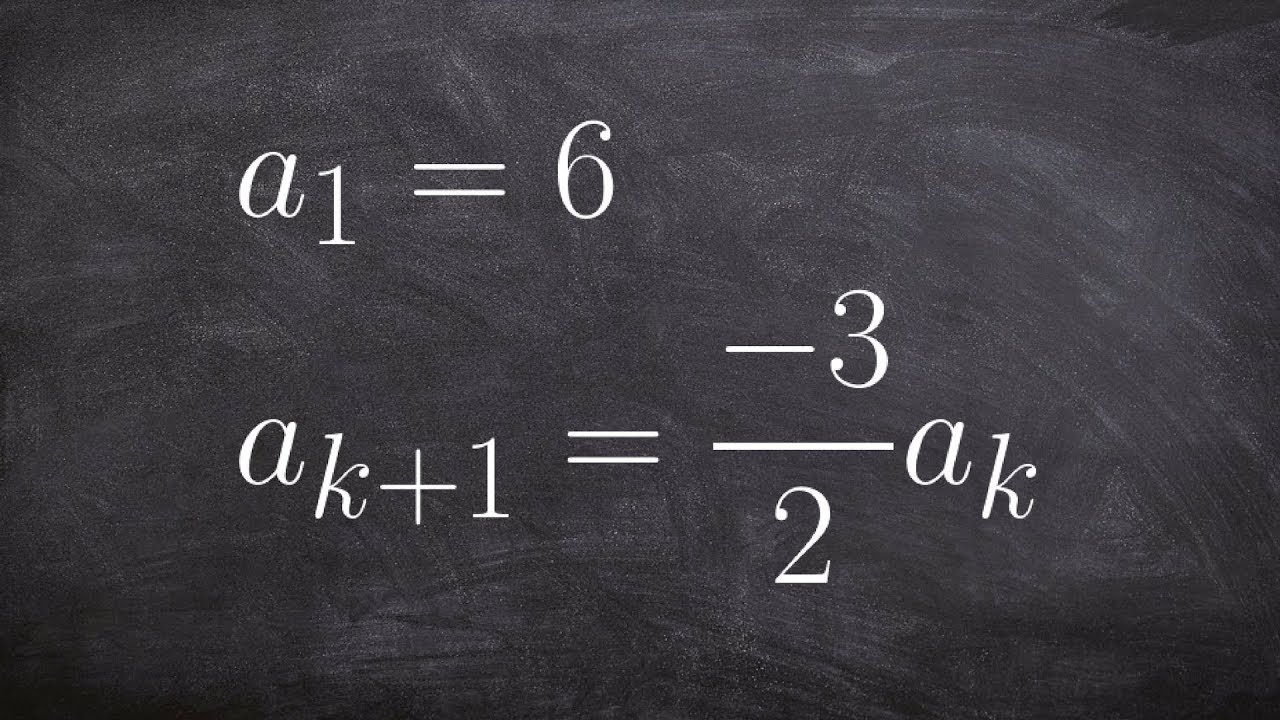
Показать описание
👉 Learn all about recursive sequences. Recursive form is a way of expressing sequences apart from the explicit form. In the recursive form of defining sequences, each term of a sequence is expressed in terms of the preceding term unlike in the explicit form where each term is expressed in terms of the first term.
The general form of the recursive arithmetic sequence is given by A_(k+1) = A_k + d, where A_(k+1) is the next term of the sequence, A_k is the current term and d is the common difference. The general form of the recursive geometric sequence is given by A_(k+1) = (A_k)r, where A_(k+1) is the next term of the sequence, A_k is the current term and r is the common ratio.
In the recursive form of sequence, it is usually very important to indicate an initial value for the sequence.
Organized Videos:
✅ Sequences
✅Sequences | Learn About
✅Determine The First Five Terms of The Arithmetic Sequence
✅How to Write The Formula for a Arithmetic Sequence
✅Find the nth Term of an Arithmetic Sequence
✅Find the First Five Terms of a Geometric Sequence
✅How to Write The Formula for a Geometric Sequence
✅Find the nth Term of a Geometric Sequence
✅How to Determine Arithmetic or Geometric Sequence
✅Find the First Five Terms of a Sequence
✅How to Write The Formula for a Sequence
✅Find the nth Term of a Sequence
✅How to Simplify Factorials
✅Recursive Sequences
✅Prove the Sum by Induction
✅Find the Given Term of Binomial Expansion
✅Binomial Expansion | Learn About
✅How to Expand a Binomial
Connect with me:
#sequences #brianmclogan
The general form of the recursive arithmetic sequence is given by A_(k+1) = A_k + d, where A_(k+1) is the next term of the sequence, A_k is the current term and d is the common difference. The general form of the recursive geometric sequence is given by A_(k+1) = (A_k)r, where A_(k+1) is the next term of the sequence, A_k is the current term and r is the common ratio.
In the recursive form of sequence, it is usually very important to indicate an initial value for the sequence.
Organized Videos:
✅ Sequences
✅Sequences | Learn About
✅Determine The First Five Terms of The Arithmetic Sequence
✅How to Write The Formula for a Arithmetic Sequence
✅Find the nth Term of an Arithmetic Sequence
✅Find the First Five Terms of a Geometric Sequence
✅How to Write The Formula for a Geometric Sequence
✅Find the nth Term of a Geometric Sequence
✅How to Determine Arithmetic or Geometric Sequence
✅Find the First Five Terms of a Sequence
✅How to Write The Formula for a Sequence
✅Find the nth Term of a Sequence
✅How to Simplify Factorials
✅Recursive Sequences
✅Prove the Sum by Induction
✅Find the Given Term of Binomial Expansion
✅Binomial Expansion | Learn About
✅How to Expand a Binomial
Connect with me:
#sequences #brianmclogan
Комментарии
 0:02:54
0:02:54
 0:07:18
0:07:18
 0:06:16
0:06:16
 0:02:39
0:02:39
 0:04:17
0:04:17
 0:06:22
0:06:22
 0:04:30
0:04:30
 0:04:15
0:04:15
 0:16:42
0:16:42
 0:11:15
0:11:15
 0:02:30
0:02:30
 0:09:54
0:09:54
 0:05:58
0:05:58
 0:07:33
0:07:33
 0:21:03
0:21:03
 0:01:40
0:01:40
 0:01:16
0:01:16
 0:04:03
0:04:03
 0:09:03
0:09:03
 0:05:59
0:05:59
 0:09:40
0:09:40
 0:06:30
0:06:30
 0:09:16
0:09:16
 0:03:12
0:03:12