Compact iff Sequentially Compact full proof, Real Analysis II

This lecture proves the equivalence between compactness and sequential compactness in any metric space, showing that a set is compact if and only if it is sequentially compact.

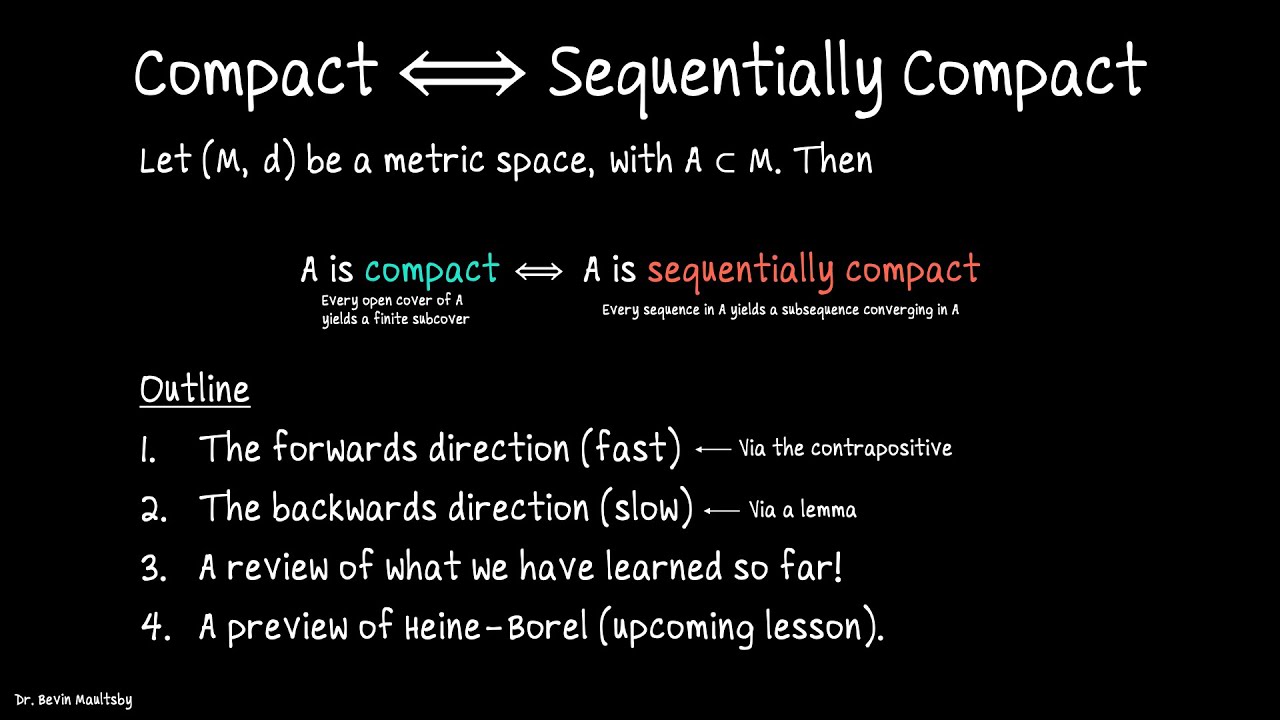
First, we review the definitions: a set is compact if every open cover has a finite subcover, and sequentially compact if every sequence has a converging subsequence whose limit is within the set. The goal of the lecture is to prove that these two properties are equivalent.

The forward direction is proved via the contrapositive: if a set is not sequentially compact, it is not compact. We construct an open cover with no finite subcover by isolating sequence points with neighborhoods. The proof concludes by showing that if the sequence lacks a convergent subsequence, the set fails to be compact.

The backward direction involves proving a lemma through contradiction. It shows that for a sequentially compact set, a special radius can be chosen around each point such that the neighborhoods lie within a single member of the open cover. Using the fact that sequentially compact sets are totally bounded, we identify a finite subcover, confirming that the set is compact.

We arrive at the major conclusion: compactness implies the set is closed and bounded, setting the stage for the Heine-Borel theorem in Euclidean spaces, where compactness is equivalent to a set being closed and bounded.

#Mathematics #RealAnalysis #AdvancedCalculus #Topology #BolzanoWeierstrass #MetricSpaces #HeineBorel #SetTheory #ClosedSets