Number Theory | Chinese Remainder Theorem: Example 4

Показать описание

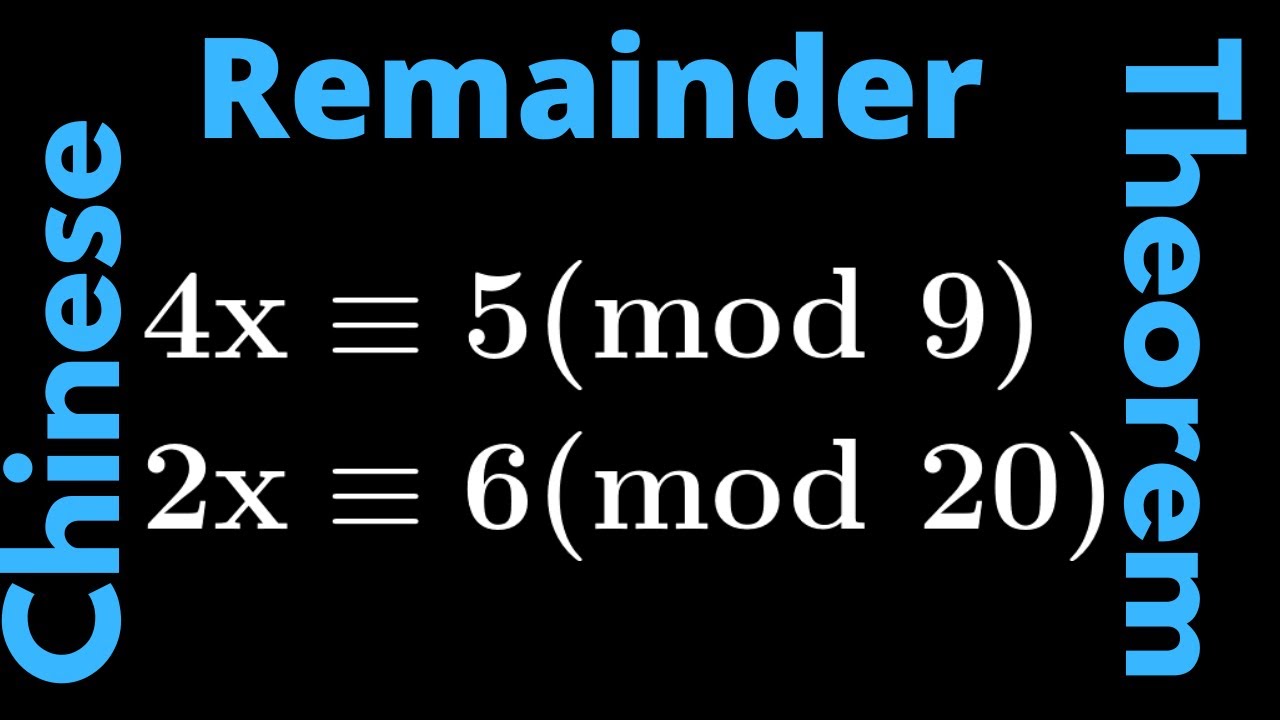
We solve a system of linear congruences using the method outline in the proof of the Chinese Remainder Theorem.

Рекомендации по теме

Комментарии

could you please explain why you added 90 in the end

tisyarawat

A trick: in this cases is much faster to solve with substitution. 2x=6 m20 means x=3 m10, so x=10n+3. Substituting in the first we get 40n+12=5 m9, or 4n=2 m9. Then you can divide, 2n=1 m9, hence n=5 m9. So n=9k+5. Substituting we get x=10n+3=90k+53.

giuseppebassi

Professor M. Penn, thank you for a powerful example that uses the Chinese Remainder Theorem. I am totally lost in your example with the numbers.

georgesadler

I'm so thankful for this channel! this explains my number theory so well :D You are the only one that I found on Extended Chinese Remainder Theorem, so thank you!

kevingepulle

Thank you so much.. Your explanation has a great help in my studies.

MrJPaul

You completely lost me when you start plugging in numbers into variables that were never mentioned

youuu

Can you please tell me how you found the inverse so quick, I mean how can we find it for bigger moduli. Isn't there any formula or what?

donducarmelduhezagire

Good Video!!
First part . I operated it like below.
4X≡5 times2 then 8X≡10 that is
－X≡1, X≡－1≡8

voicebymathematicschannel

Thank you, super helpful for the problem I was doing.

mrbradley

Your Videos are so helpful. Thanks a lot

schirox

Thank you..it was at first Abit hard to understand but its clear now.. thanks Sir!

khahamdebbarma

I am Indian but I like your teaching and help me for higher studies

abhishekgkworld

I don't get the point at the end: there is one solution: x = 53 (mod 90)

keinKlarname

I don't get what you meant by multiplying by 7

randobravo

Si les deux modulos sont des entiers successifs, il existe, dans ce cas, un truc accélérateur.
Soit le système
x = a [m]
x = b [m+1]
Alors, le déterminant d'ordre 2x2
|a m |
|b m+1|
est une solution (modulo m(m+1) puisque m et m+1 sont premiers entre eux).
L'énoncé permet d'écrire
x = -1 [9]
x = 3 [10]
Donc X = -10-27 = -37 = 53 [90]

michelmegabacus

How does those 2, 2, 2 got cancelled ?!?

somasahu

Please explain 0:47 part I dont understand it

learneducationfiles

Great video! But I want to understand how N1 and N2 became suddenly equal to 10 and 9 respectively?

Snapeserverussnape

Great sir...you have same name with me "Penn"

Princesstherine

Bonjour Monsieur
je vous informe que de tels exercices, je les résouds beaucoup plus facilement au moyen du schéma d'Ouragh . En voici la preuve . A partir du système
4x_=5[9] et x_=3[10] on aura

et donc
Cordialement

ouraghyoussef

Number Theory | Chinese Remainder Theorem: Example 4

The Chinese Remainder Theorem made easy

The Chinese Remainder Theorem (Solved Example 1)

Number Theory | Chinese Remainder Theorem: Example 4

Number Theory | Chinese Remainder Theorem Proof

Chinese Remainder Theorem

Number Theory | Chinese Remainder Theorem: Example 2

Number Theory | Chinese Remainder Theorem: Example 1

Number Theory | Chinese Remainder Theorem: Example 3

Chinese Remainder Theorem | Sun Tzu's Theorem

Chinese Remainder Theorem -- Number Theory 11

The Chinese Remainder Problem

Chinese Remainder Theorem and Cards - Numberphile

Chinese Remainder Theorem

Congruences |Part 8| Chinese Remainder Theorem

Chinese remainder Theorem | Proof of Chinese Remainder Theorem | Number Theory |Ug Maths |BSc maths

Learn Chinese Remainder Theorem using Sun Tzu's Problem | Number Theory for Math Olympiad

Number Theory : Chinese Remainder Theorem Example and Solution

Number Theory | Solving Quadratic Congruences with Hensel's Lemma

Intro to Chinese Remainder Theorem and Euler's Totient Theorem via a Challenging Problem

Number Theory | A Generalization of the Chinese Remainder Theorem

Chinese remainder theorem example

Number Theory - The Chinese Remainder Theorem

13. Linear Congruency | Chinese Remainder Theorem | Examples | Number Theory | Ravina Tutorial

Congruence, Chinese Remainder Theorem, Common solution of linear congruence, Number Theory, Lec-85