filmov
tv
Calculus 2, Lecture 37A, Competing Species Model Done by Hand (with Nullclines) and on Mathematica
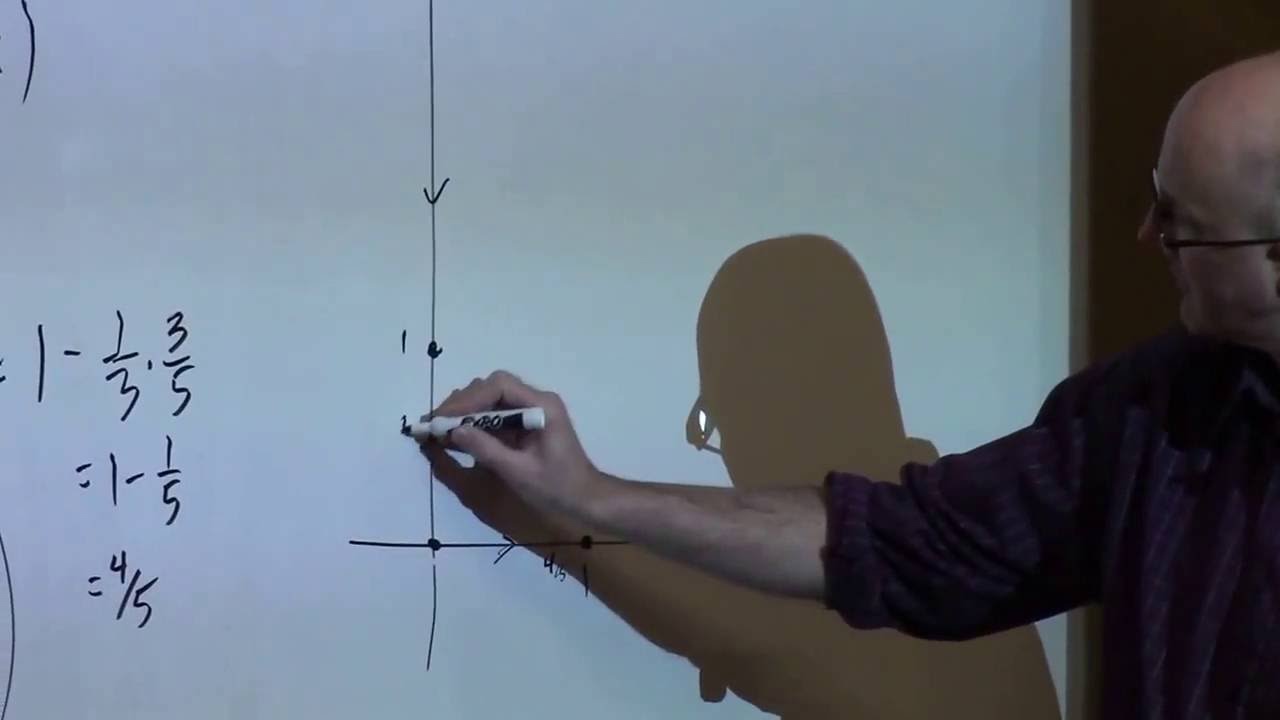
Показать описание
Calculus 2, Lecture 37A. Use nullclines to do a phase plane analysis by making a phase portrait of a competing species model. Get as far as we can by hand and then use NDSolveValue and VectorPlot on Mathematica to confirm the results. Make the phase portrait "physical" by using vectors and Manipulate to simulate the motion of solution curves in the phase plane.
(0:00) Course evaluation information.
(1:04) Lecture plan.
(2:21) Competing species model (interaction terms have negative coefficients) analysis.
(3:48) Behavior of the population of each species in the absence of the other (logistic model).
(8:42) Find the other equilibrium point strictly in the first quadrant when x and y are both positive (solve a system of two linear equations).
(12:30) Find the nullclines (x- and y-nullclines).
(17:05) Use the sign of f(x,y) and g(x,y) to determine the directions of solution curves.
(19:57) Draw some approximate solution curves, crossing nullclines correctly and draw a general qualitative real-life conclusion.
(22:30) Confirm the phase plane with Show, VectorPlot, ContourPlot, and ListPlot on Mathematica.
(26:52) Use NDSolveValue and ParametricPlot on Mathematica to plot solution curves.
(30:16) Use Manipulate to make an animation and Graphics and Arrow to make position, velocity, and acceleration vectors to the picture.
(35:50) Graph the speed and distance traveled functions for the curve's motion through the phase plane.
(0:00) Course evaluation information.
(1:04) Lecture plan.
(2:21) Competing species model (interaction terms have negative coefficients) analysis.
(3:48) Behavior of the population of each species in the absence of the other (logistic model).
(8:42) Find the other equilibrium point strictly in the first quadrant when x and y are both positive (solve a system of two linear equations).
(12:30) Find the nullclines (x- and y-nullclines).
(17:05) Use the sign of f(x,y) and g(x,y) to determine the directions of solution curves.
(19:57) Draw some approximate solution curves, crossing nullclines correctly and draw a general qualitative real-life conclusion.
(22:30) Confirm the phase plane with Show, VectorPlot, ContourPlot, and ListPlot on Mathematica.
(26:52) Use NDSolveValue and ParametricPlot on Mathematica to plot solution curves.
(30:16) Use Manipulate to make an animation and Graphics and Arrow to make position, velocity, and acceleration vectors to the picture.
(35:50) Graph the speed and distance traveled functions for the curve's motion through the phase plane.
 0:38:11
0:38:11
 0:11:18
0:11:18
 0:12:08
0:12:08
 0:06:42
0:06:42
 0:30:47
0:30:47
 0:06:36
0:06:36
 0:06:46
0:06:46
 0:26:42
0:26:42
 0:01:25
0:01:25
 0:00:47
0:00:47
 0:33:54
0:33:54
 0:39:12
0:39:12
 0:52:14
0:52:14
 1:27:04
1:27:04
 0:01:50
0:01:50
 0:09:40
0:09:40
 0:07:53
0:07:53
 0:32:30
0:32:30
 0:04:24
0:04:24
 0:00:06
0:00:06
 0:03:22
0:03:22
 0:38:00
0:38:00
 1:22:52
1:22:52
 0:02:35
0:02:35