filmov
tv
Prove the Double Angle Trigonometric Identities: Step-by-Step Explanation
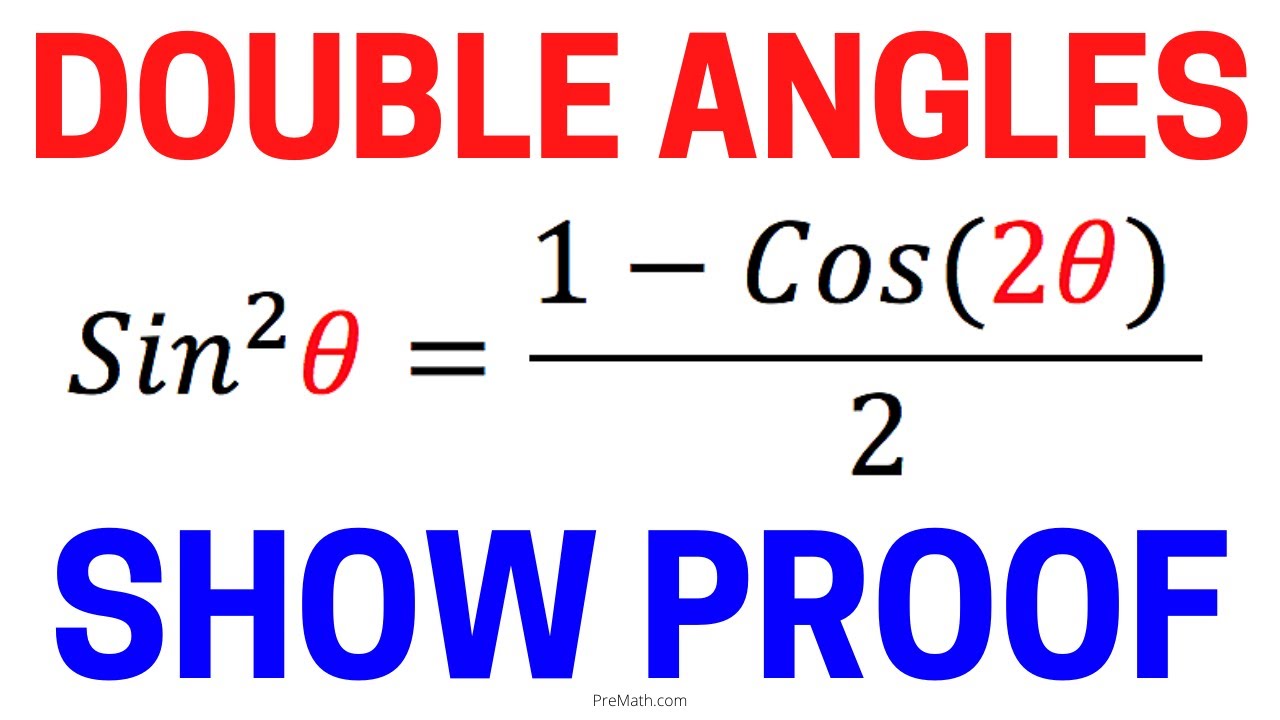
Показать описание
A proof to remember: Double Angle Formulas I (visual proof)
Trig Double Angle Formulas from Semicircle (visual proof)
Prove the Double Angle Trigonometric Identities: Step-by-Step Explanation
Trigonometry - Proof of the Double Angle Formulae : ExamSolutions
Verifying Trigonometric Identities With Double Angle Formulas
The Double-Angle Formulas | proof without words| mathocube|
Proving the Double Angle Trig Identities (sin2x=2sinxcosx)
Double Angles: Prove this Trigonometric Identity
Trigonometry | Double Angle Formulae - Proof, Worked Examples and Trial Questions
Prove Trigonometric Identity Using Double Angle Formula
Double Angle Formula
Proof: Double Angle Trig Identities (SIN, COS, and TAN)
Proving the Double and Half Angle Formulas for Trigonometry (Precalculus - Trigonometry 27)
PreCalculus - Trigonometry: Trig Identities (23 of 57) Double Angle Formula Proved: Sine
Double Angle Identities & Formulas of Sin, Cos & Tan - Trigonometry
How to prove equations using double-angle identities
Proof of Double angle Trigonometric identities Advanced level pure Mathematics ZIMSEC by Trotter
PreCalculus - Trigonometry: Trig Identities (24 of 57) Double Angle Formula Proved: Cosine
Proving a Trigonometric Identity Using Double Angle Identities (Example)
Solve Trigonometric Equation Double Angle Formula
Verify Trigonometric Identities Double Angle
deriving the double angle formulas
Simplifying a Trigonometric Expansion with Double Angle Identities
Double Angle Trig Identity: “Proof” via Areas
Комментарии
 0:02:01
0:02:01
 0:03:44
0:03:44
 0:04:16
0:04:16
 0:08:25
0:08:25
 0:15:19
0:15:19
 0:02:36
0:02:36
 0:02:37
0:02:37
 0:04:45
0:04:45
 0:19:21
0:19:21
 0:03:33
0:03:33
 0:00:54
0:00:54
 0:01:35
0:01:35
 0:27:17
0:27:17
 0:02:06
0:02:06
 0:18:16
0:18:16
 0:06:35
0:06:35
 0:04:23
0:04:23
 0:01:15
0:01:15
 0:04:07
0:04:07
 0:04:42
0:04:42
 0:02:15
0:02:15
 0:07:49
0:07:49
 0:08:00
0:08:00
 0:00:39
0:00:39