filmov
tv
Session 10: Absolute maxima and minima of a function over closed and bounded domains.

Показать описание
Join this channel to get access to perks:
Hessian matrix, local maxima, local minima
Hessian matrix, local maxima, local minima
Session 10: Absolute maxima and minima of a function over closed and bounded domains.
14.7 Abs max and min | Anas Abu Zahra
Absolute Maximum and Minimum Over a Box
Race Highlights | 2021 Abu Dhabi Grand Prix
Lecture 9: Questions on finding absolute maxima & minima on bounded domain
Interactive Lec 09 Session 01 Absolute Maxima Minima
*QUICK* How To LEVEL UP XP FAST in Fortnite CHAPTER 5 SEASON 4! (INSANE AFK XP Glitch Map Code!)
Find absolute max and min of a function on a closed interval.
Labtube-(Calculus 1000)-Global Max/min
I took Minecraft's Most Popular Challenge to its Absolute Limits
Absolute max/min over a rectangle
Absolute max/min over a disc
Every Weapon in CODM Ranked From WORST to BEST (Season 10)
Pushing your Season of Discovery Rogue to the ABSOLUTE LIMIT
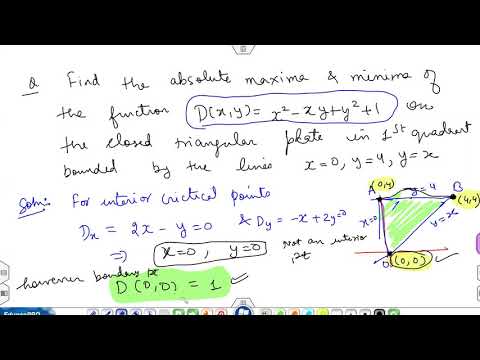
Video3166 - Calculus 3 - Absolute Maximum/Minimum - Bounded Region - Part 1/2
Top 10 Solo Showdown Brawlers! (Season 31)
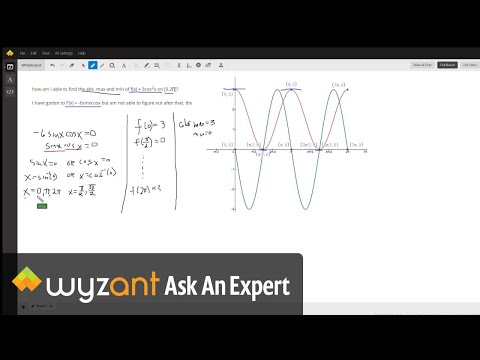
Find absolute max and min on [0,2pi] for f(x) = 3cos^2(x).
Absolute Maximum and Minimum || Part 2
Preview - Absolute Power Part 2
Calculus 3rd secondary Egypt 2022 | Unit-3 | Lesson-10 | Absolute maximum and absolute minimum
Session 20: Absolute Maximum Shear Stress - In-class, Live Session
Find absolute minimum and absolute maximum of f(x) on [0,23]
Find absolute max/min values of a function on 0 to pi.
CEEN311 LE10 Absolute Maximum Shear Stress
Комментарии
 0:19:17
0:19:17
 0:23:50
0:23:50
 0:11:42
0:11:42
 0:08:08
0:08:08
 0:32:36
0:32:36
 0:40:07
0:40:07
 0:01:47
0:01:47
 0:02:39
0:02:39
 0:38:51
0:38:51
 0:35:19
0:35:19
 0:10:10
0:10:10
 0:12:37
0:12:37
 0:27:16
0:27:16
 0:12:37
0:12:37
 0:11:10
0:11:10
 0:34:53
0:34:53
 0:03:00
0:03:00
 0:19:11
0:19:11
 0:00:42
0:00:42
 0:42:37
0:42:37
 1:19:46
1:19:46
 0:06:24
0:06:24
 0:05:22
0:05:22
 0:04:36
0:04:36