filmov
tv
[Discrete Mathematics] Integer Partitions

Показать описание
We talk about the number of ways to partition an integer.
*--Playlists--*
*--Recommended Textbooks--*
We're going to use generating functions to figure out how many ways we can partition an integer. Then, we'll look at a cool proof.
Hello, welcome to TheTrevTutor. I'm here to help you learn your college courses in an easy, efficient manner. If you like what you see, feel free to subscribe and follow me for updates. If you have any questions, leave them below. I try to answer as many questions as possible. If something isn't quite clear or needs more explanation, I can easily make additional videos to satisfy your need for knowledge and understanding.
*--Playlists--*
*--Recommended Textbooks--*
We're going to use generating functions to figure out how many ways we can partition an integer. Then, we'll look at a cool proof.
Hello, welcome to TheTrevTutor. I'm here to help you learn your college courses in an easy, efficient manner. If you like what you see, feel free to subscribe and follow me for updates. If you have any questions, leave them below. I try to answer as many questions as possible. If something isn't quite clear or needs more explanation, I can easily make additional videos to satisfy your need for knowledge and understanding.
[Discrete Mathematics] Integer Partitions
Integer Partitions (Discrete Maths)
Recurrence for partitions into k parts (visual proof)
What are interesting integer partition problems?
Generating functions for integer partitions
Discrete Math 2 - Tutorial 22 - Partition of integers
[Introduction to Combinatorics] Lecture 5. Integer partitions
GTA Session 12 - PARTITION OF INTEGERS
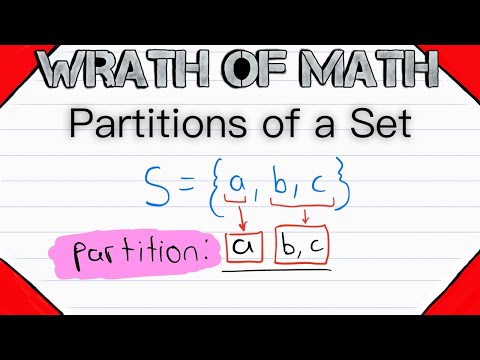
Partitions of a Set | Set Theory
Discrete Math 2 - Tutorial 23 - Partition of Integers Ex.
Introduction to Integer Partitions -- Number Theory 28
Generating Functions Part 6: Integer Partitions 1
Young Diagrams of Integer Partitions up to 12 (synthwave; enumeration)
Integer Partitions Part 1. MATH 222, Discrete and Combinatorial Math, University of Victoria.
Partitions
Discrete Math - Rose - Partition Numbers
Discrete Math - Partition Numbers - Part 1 of 2
Integer Partitions Part 2. MATH 222, Discrete and Combinatorial Math, University of Victoria.
Integer partitions
L04.7 Partitions
Partitions of an integer: each part a limited # of times (Tut 9, 1a)
What does a ≡ b (mod n) mean? Basic Modular Arithmetic, Congruence
Equivalence Classes and Partitions
Math 532 - 6.2 - Partitions into k parts
Комментарии
![[Discrete Mathematics] Integer](https://i.ytimg.com/vi/XN3Vy2M5ov8/hqdefault.jpg) 0:17:15
0:17:15
 0:08:06
0:08:06
 0:03:40
0:03:40
 0:00:45
0:00:45
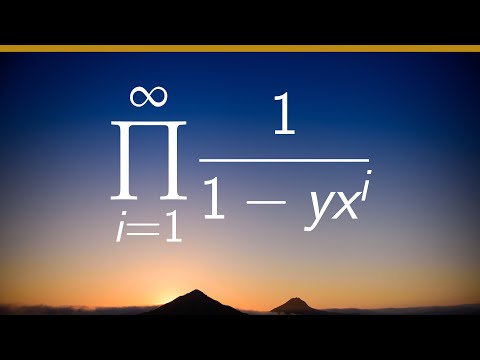 0:16:11
0:16:11
 0:08:07
0:08:07
![[Introduction to Combinatorics]](https://i.ytimg.com/vi/2xZhIwc3tK0/hqdefault.jpg) 0:16:34
0:16:34
 0:05:56
0:05:56
 0:07:59
0:07:59
 0:05:40
0:05:40
 0:25:03
0:25:03
 0:12:15
0:12:15
 0:02:53
0:02:53
 0:21:03
0:21:03
 0:03:33
0:03:33
 0:19:14
0:19:14
 0:26:56
0:26:56
 0:18:38
0:18:38
 0:31:22
0:31:22
 0:05:20
0:05:20
 0:03:48
0:03:48
 0:05:45
0:05:45
 0:13:10
0:13:10
 0:10:31
0:10:31