filmov
tv
Fraunhofer Diffraction Explained

Показать описание
If you want to see more of these videos, or would like to say thanks for this one, the best way you can do that is by becoming a patron - see the link above :). And a huge thank you to all my existing patrons - you make these videos possible.
In this video, I describe the process of Fraunhofer diffraction (also known as far-field diffraction) in terms of the Fourier Transform and Fourier Optics. I go over the assumptions that underlie Fraunhoffer diffraction (both the paraxial approximation and the small-aperture approximation), and give the mathematical form that it takes.
This is part of my graduate series on optoelectronics / photonics, and is based primarily on Coldren's book on Lasers as well as graduate-level coursework I have taken in the EECS department at UC Berkeley.
Hope you found this video helpful, please post in the comments below anything I can do to improve future videos, or suggestions you have for future videos.
In this video, I describe the process of Fraunhofer diffraction (also known as far-field diffraction) in terms of the Fourier Transform and Fourier Optics. I go over the assumptions that underlie Fraunhoffer diffraction (both the paraxial approximation and the small-aperture approximation), and give the mathematical form that it takes.
This is part of my graduate series on optoelectronics / photonics, and is based primarily on Coldren's book on Lasers as well as graduate-level coursework I have taken in the EECS department at UC Berkeley.
Hope you found this video helpful, please post in the comments below anything I can do to improve future videos, or suggestions you have for future videos.
Fraunhofer Diffraction Explained
Diffraction: Why Does It Happen? (Physics Explained for Beginners)
Interference, Reflection, and Diffraction
Fresnel Diffraction Explained
Diffraction interference patterns with phasor diagrams
fresnel and fraunhofer diffraction
Fraunhofer Diffraction due to single slit
Fraunhofer Diffraction due to Single Slit
Fresnel Diffraction 🆚️ Fraunhofer Diffraction #Physics_Concept #Shorts #physics #neet #neet2023
Maxima in Single slit diffraction | Wave optics | Physics | Khan Academy
Diffraction Pattern of Light by Single Slit Using Two Blades....
Single-Slit Fraunhofer Diffraction
Fraunhofer|Diffraction|Single|Slit|Physics 12|Tamil|Muruga MP
FRAUNHOFER DIFFRACTION AT SINGLE SLIT. SHORT VIDEO. EASILY EXPLAINED.
Physics - Optics: Single Slit Diffraction (1 of 15) Basics
Diffraction of light || Animated explanation in || Wave Optics|| Physics 12th class
Fraunhoffer Diffraction at a Single Slit
40.Chapter - 10 | Wave Optics | Diffraction From Single Slit | Physics Baba 2.O
diffraction due to single slit #diffraction #physics #bsc #optics #physicaloptics
fraunhofer diffraction due to single slit
Wave Optics: Fraunhofer and Fresnel diffraction
Fraunhofer diffraction। fraunhofer diffraction in hindi
PHYS 201 | Slit Diffraction 3 - Fraunhofer Diffraction
Fresnel diffraction of a circular aperture
Комментарии
 0:13:35
0:13:35
 0:15:43
0:15:43
 0:06:18
0:06:18
 0:09:27
0:09:27
 0:17:31
0:17:31
 0:12:12
0:12:12
 0:17:18
0:17:18
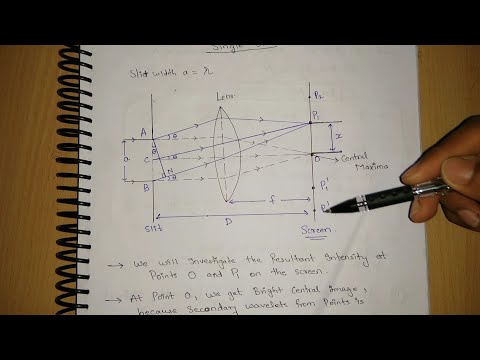 0:12:51
0:12:51
 0:00:51
0:00:51
 0:08:47
0:08:47
 0:00:26
0:00:26
 0:06:48
0:06:48
 0:27:17
0:27:17
 0:00:24
0:00:24
 0:05:22
0:05:22
 0:02:11
0:02:11
 0:11:15
0:11:15
 0:22:08
0:22:08
 0:00:13
0:00:13
 0:21:26
0:21:26
 0:05:10
0:05:10
 0:05:39
0:05:39
 0:07:41
0:07:41
 0:00:21
0:00:21