Locker Problem

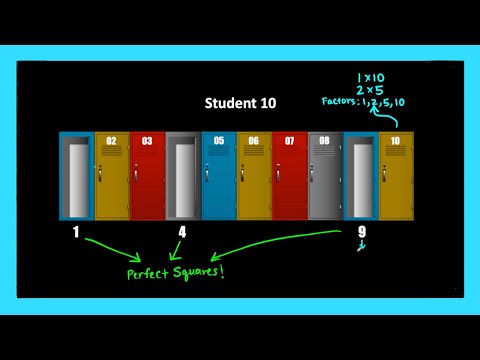
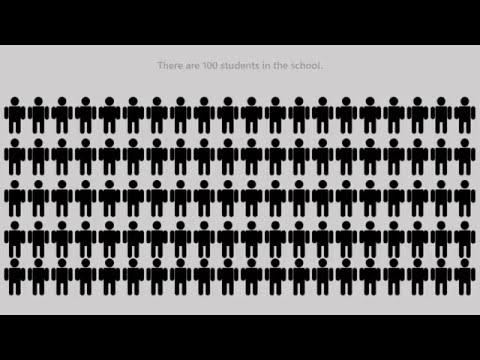
A classic math teaser - the locker problem. Imagine a row of 100 closed lockers numbered sequentially. 100 people walk by the lockers. The first person changes the state of every locker -- in this case they open every locker. The second person changes the state of every second locker -- they will close lockers 2, 4, 6, 8, and so on. The third person changes every 3rd locker -- closing locker 3, opening 6, and so on. This continues until all 100 people walk by. The 100th person just touches the 100th locker. Which lockers are open?