filmov
tv
Is Subset an Equivalence Relation? | Set Theory
Показать описание
Is the subset relation on all sets and equivalence relation? If so, it must be reflexive, symmetric, and transitive! We'll prove in today's set theory lesson that subset is a reflexive relation and a transitive relation, but it is not symmetric. If A is a subset of B, we cannot conclude that B is a subset of A.
For an example of sets that violate the symmetric property, consider any proper subset A of a set B. If A is a proper subset, then A is a subset of B and B will not be a subset of A. For a more specific example, consider A = integers and B = real numbers.
I hope you find this video helpful, and be sure to ask any questions down in the comments!
+WRATH OF MATH+
Follow Wrath of Math on...
For an example of sets that violate the symmetric property, consider any proper subset A of a set B. If A is a proper subset, then A is a subset of B and B will not be a subset of A. For a more specific example, consider A = integers and B = real numbers.
I hope you find this video helpful, and be sure to ask any questions down in the comments!
+WRATH OF MATH+
Follow Wrath of Math on...
0:07:21
Is Subset an Equivalence Relation? | Set Theory
0:06:29
Equivalence Relation
0:04:36
Equivalence Relations - Reflexive, Symmetric, and Transitive
0:07:19
Equivalence Classes
0:00:49
verify equivalence relation. P(X) is a set of all Subsets of X. A, B ∈ P. ARB if and only if A ⊂ B...
0:08:21
(Abstract Algebra 1) Definition of an Equivalence Relation
0:22:30
Discrete Math - 9.5.1 Equivalence Relations
0:02:25
equivalence relation reflexive transitive mathematics number of subsets of a set power set iit jam
0:00:15
Show that R={(x,y): 5 divides x-y} is an equivalence relation on Z
0:17:26
Discrete Math 9.5.1 Equivalence Relations
0:03:58
Basic Set theory: Proof of the reflexivity of the subset relation.
0:15:36
RELATIONS - DISCRETE MATHEMATICS
0:08:53
Two Equivalence Classes [a] and [b] Are Equal If and Only If a is Related to b
0:00:58
Proving two equivalence classes are equal
0:07:04
Abstract Algebra | Equivalence Relations
0:07:02
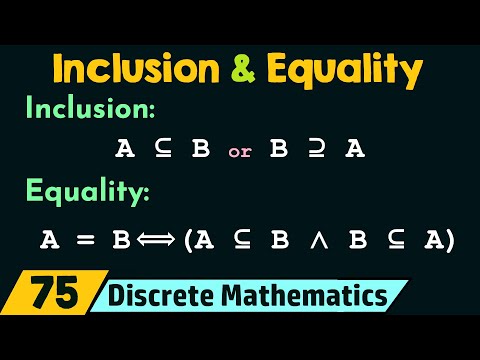
Inclusion and Equality
0:05:01
What is an Equivalence Relation? | Reflexive, Symmetric, and Transitive Properties
0:17:34
Equivalence Relation | Disjoint Subsets | Part 2
0:14:38
Abstract Algebra | Partitions and Equivalence Relations
0:06:39
Types of Relations (Part 1)
0:03:20
#mathphile#relationsfunctions Any relation is a subset of the largest equivalence relation on set A!
0:02:21
Q8 Misc Ex Ch 1 R&F XII Maths Given a non empty set X, consider P(X) which is the set of all sub...
0:07:39
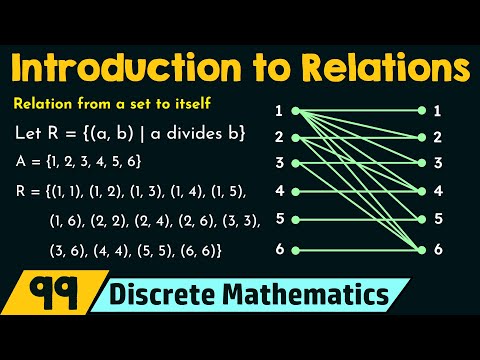
Introduction to Relations
0:12:38
Subset Relation is a Partial Order
Комментарии