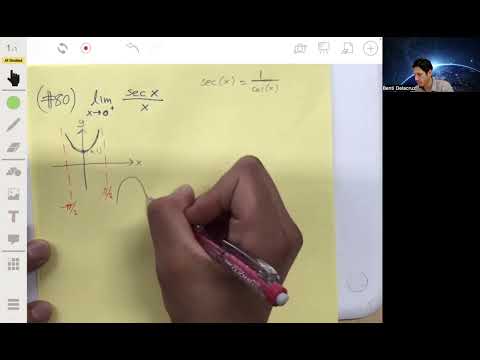
Find the Limit of sec(x) as x approaches negative pi/2 from the left

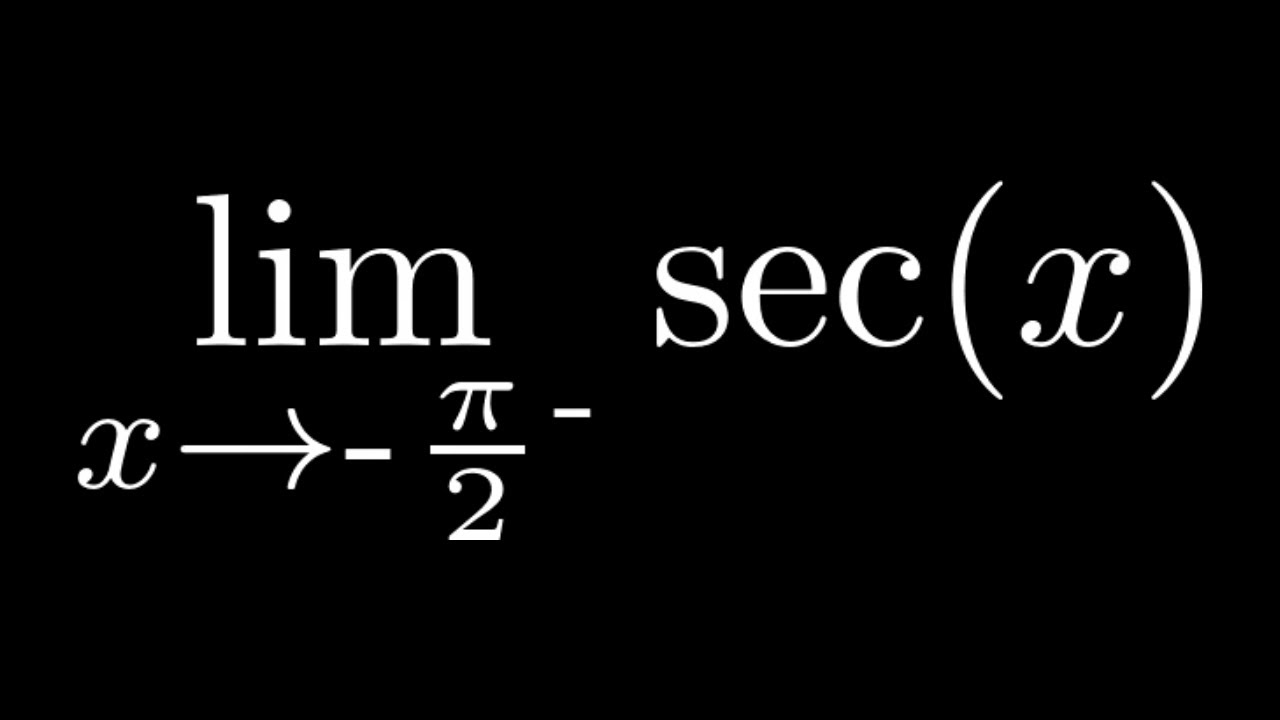
Показать описание

Find the Limit of sec(x) as x approaches negative pi/2 from the left

If you enjoyed this video please consider liking, sharing, and subscribing.

There are several ways that you can help support my channel:)

************Udemy Courses(Please Use These Links If You Sign Up!)*************
Abstract Algebra Course

Advanced Calculus Course

Calculus 1 Course

Calculus 2 Course

Calculus 3 Course

Calculus 1 Lectures with Assignments and a Final Exam

Calculus Integration Insanity

Differential Equations Course

Differential Equations Lectures Course (Includes Assignments + Final Exam)

College Algebra Course

How to Write Proofs with Sets Course

How to Write Proofs with Functions Course

Trigonometry 1 Course

Trigonometry 2 Course

Statistics with StatCrunch Course

Math Graduate Programs, Applying, Advice, Motivation

Daily Devotionals for Motivation with The Math Sorcerer

Thank you:)

Рекомендации по теме

Комментарии

Technically, lim sec(x) (x < –π/2, x —> –π/2) does not exist. However, given x < –π/2, x —> –π/2 implies sec(x) —> –∞, insofar as this is just an abbreviation to say that for all real numbers B < 0, there exists some real number δ > 0, such that for all real numbers –δ < x + π/2 < 0, sec(x) > B.

angelmendez-rivera

Awesome explanation! I looked at the secant graph and I can also see it approached - infinity. What happens if was sec(x)^2?

DVKN

Cool. Interesting considerations with -infinity, +infinity and infinity. Consider a complex plane C has only one infinity, real numbers R has 2 infinities, but real numbers R is a subset of complex plane C. How come?

ivolva

Find the Limit of sec(x) as x approaches negative pi/2 from the left

Find the Limit of sec(x) as x approaches negative pi/2 from the left

Find the Limit of sec(x)/(1 - sin(x)) as x approaches 0

Trigonometric Functions: Finding a One-Sided Limit of (sec x)/x | AP Calculus

Limit of sec(x) as x approaches -pi/2 from the right.

How to Compute a One Sided Limit with a Secant Function

Calculus Infinite Limits xsec(x) as x approaches pi/2 from the right

Derivative of sec(x) using limit definition

Question 3 : Limit of (sec(x) - 1)/x

Finding the arclength of an explicit curve | SETMind Tutoring | MATH 1043

Find the limit.K\lim_{x\rightarrow(\pi/2)}\frac{\sec x}{\tan x} | Plainmath

Limit of x sec x as x approaches 0

Derivative of Sec(x) Proof (Using the Limit Definition)

Calculus 4: Limits of Trigonometric Functions sec x, csc x, cot x

Evaluate the limit of sec x - tan x as x approaches pi/2 using L’Hospital’s rule

derivative of sec(x), quotient rule, calculus 1 tutorial

XI Limits and Derivatives Evaluate lim y → 0 {x+y sec x + y x secx+y} y

Where is The Function f(x) = sec x Continuous

Definite trigonometric integral with power of secant: integrate (sec(x))^2 on 0 to pi/4.

Limits of trigonometric functions | Limits and continuity | AP Calculus AB | Khan Academy

Limit of (3 sec(x)+5)/tan(x) As x Approaches pi/2

Derivative of sec(x) from first principles (definition)

Evaluate the limit as x approaches pi/2 of (1+ sec x)/(tan x). l’Hopital’s Rule

Derivative of sec x

Integral of sec(x) but without that trick!