Stokes's Theorem

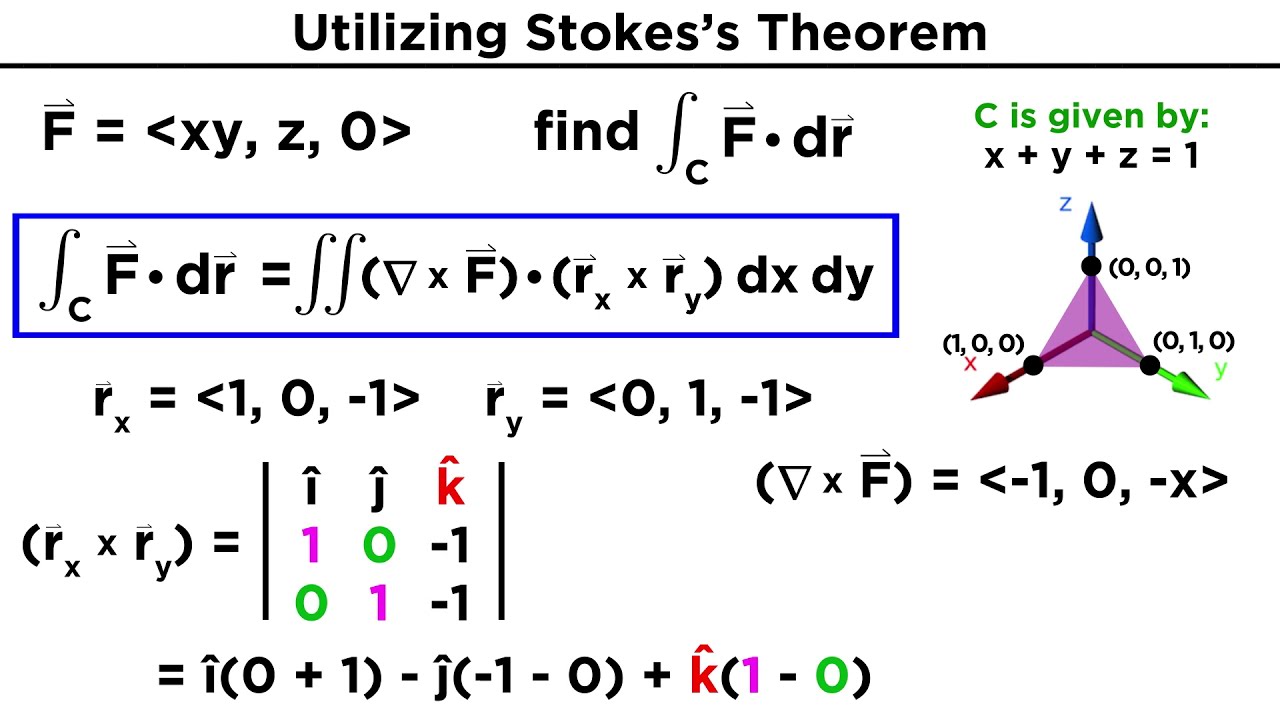
Stokes's Theorem is kind of like Green's Theorem, whereby we can evaluate some multiple integral rather than a tricky line integral. This works for some surface integrals too. Let's see how it works!

Script by Howard Whittle

Check out "Is This Wi-Fi Organic?", my book on disarming pseudoscience!

[slimegolem]

Solution to the problem:                r(x) x r(y) = < -2x, -2y, 1>         del x F = <x, 0, -z>,   (z = x^2 + y^2 --> -z = -(x^2 + y^2) = -x^2 -               Integral: 0<x<2, 0<y<2     <-2x, -2y, 1> (dot) <x, 0, -(x^2 + y^2)> dx dy --> -2x^2 - (x^2 + y^2) dx dy = -2x^2 - x^2 - y^2 dx dy = -3x^2 - y^2 dx dy.  Would be helpful to first solve the integral in terms of dy instead of dx, this makes the solving a bit easier.hope it helps.

[frentz]

Hey Professor Dave. first time viewer, was just passing through. I used to teach at University. good video! impressed by two things #1 your overall presentation has a strong "tightness." no unnecessary audio or visual; the student can focus. #2 you gave the students an exercise! bravo [ claps ] .. so rare that people remember that they are talking to a human being, and that there may actually be a learning outcome LOL

[fuge]

Soooo who else has a calc 3/4 final???? lol

[sooryaprakash]

Tomorrow I have a physics exam .thanks for uploading this dave.
I am learning physics, biology, chemistry, maths from you. All your videos are short and provides knowledge with clarity. Thanks for everything man your videos mean a lot to me.

[AGhostyProduction]

bringing clarity to those in the midst of uncertainty and confusion is so powerful. thank you sir don't stop your passion

[sergeiivanov]

What remains identical or similar in the Green, Gauss, and Stokes theorems is the logic which can be seen in the generalized Stokes theorem.

[gavin]

Shout out to all the homies who are reviewing these the night before a Calc final. Goodluck to us all.

[farhanalam]

Professor Dave, you are probably the best youtuber at explaining concepts logically. Everytime I come to this channel to learn something, I learn it well. Thanks a lot

[shahinabeevis]

Thank you Dave..
I have no idea about my mathematics exam.But this give excellent study material.

[jahjahjah]

when someone finishes your video and thinks "it's just that?", it means you're a good teacher.

[atifsultan_mech]

When curlF . (Rx x Ry) is calacualted we cannot simply put the limits of x and y equal 0 to 2. this is because the projection in x-y plane is is circle and we need to use the polar coordinates to sove it. I guess the answer should -16pi

[yashovardhandubey]

Thanks a lot... I used to hate organic chemistry.... But because of you I'm starting to get better.... Professor dave, you the real MVP!!

[darcash]

Summary:
- We have F, some vector space <a, b, c>, where a, b, and c can each contain x, y, and z.
- We have C, which is the boundary of our surface, some equation involving x, y, and z.
- We have r, <x, y, {x, y}>, where {x, y} is z in terms of x and or y [isolate z from our curve equation]

1) Find the curl: Del x F
2) Find the normal vector of our plane surface: d/dx (r) x d/dy (r)

3) Dot product 1) and 2)
4) Take the double integral of 3. Look at the surface x and y span, may need to sketch it out. If they are not constant (like the box at the end's sample problem), you will need to find x in terms of y or y in terms of x depending on which you want to integrate first.


The part I'm least sure about is 4. In the main example, we have x + y + z = 1, and use (1, 0) and (0, 1). The reason we ignore z seems to be because we already have the integral in terms of x and y. Let's say we have ax + by + cz = d. More simply, we use ax + by = d. It seems like the points we use for the sketch is where the other variable = 0, or ax = d, or by = d.

Idk if that is even right tho, is it? and what about other cases, too--besides something where its just constants for x and y like the sample prob at the end [And assuming the graphs of them are not just given to you to make it easy to see]

OH and how do we calc whether or not it is positively oriented

[HarryJohnson]

Holy shit, I just watched your discussion with Jesse Lee Peterson. That was hilarious. I had no idea you were also the same guy that helped me pass Calc 3. Thanks so much sir. Please keep continuing your work. You’ve been such great help

[sikespiegel]

There's actually an easier method to calculate the normal vector to the phi--> x+y+z -1 plane. Using grad (phi) or (del operator)*(phi), where del = (∂/ ∂x)i + ( ∂/ ∂y)j + ( ∂/ ∂z)k, so doing the (del operator)*(phi) = 1i +1j +1k. This method can be used to find out the normal of all planes provided we have their equations.

[bendavis]

Thanks Dave! I got kind of stuck on that problem at the end, but realized I made a stupid arithmetical mistake that was driving me crazy. Still stoked that I got the process right, even if the I didn't get the exact answer. Hopefully my mental calculator gets better so that I can check both boxes!

[ivowehsely]

To be honest you are single handedly saying my calculus grade! Love the videos and the presentation! Keep going!

[Meenameme]

I am eternally grateful for this. Just saved my whole major. 🙏

[MikeyMyra]

I have my calcII exam tomorrow, thanks for the last minute help!

[arik]

so from the comments I've gathered that the boundaries are taken as is in the question (0<x<2) and (0<y<2). However, this doesn't make sense because the surface equation z = x^2 + y^2 describes a cone-like shape, and if there's a box that contains some of this with the given boundaries, when you project it in the XY plane you get a quadrant line so the y boundary I calculated as; 0 to 4 - x^2. Have I visualized the problem wrong? My final answer was ~28 and the answer you give is ~21, so pretty similar but I guess that doesn't mean anything if I've visualized it wrong? Can someone explain why you don't find the boundaries for integration like I have?