filmov
tv
Variations | Direct, Inverse and Joint Variation

Показать описание
#Variations #Direct #Inverse #Joint
“Variation” defines a concept that deals with variability in mathematics. Variation is defined by any change in some quantity due to change in another. We often come across with different types of variation problems in mathematics. Variation problems involve fairly simple relationships or formulas, involving one variable being equal to one term. Here follows the most common kinds of variation.
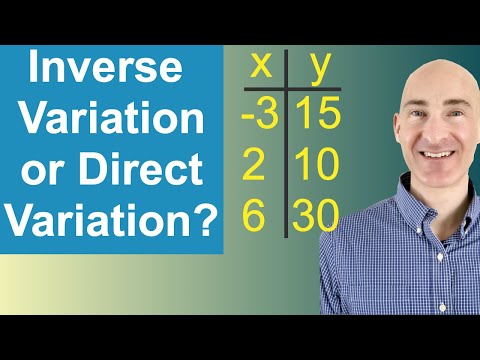
The constant of variation in a direct variation is the constant (unchanged) ratio of two variable quantities. In the following equation y varies directly with x, and k is called the constant of variation:
y = kx
Another form of variation is the inverse variation which works when there is a relationship between two variables in which the product is a constant. When one variable increases the other decreases in proportion so that the product is unchanged.
In problems relating to two or more variables, it is seen that the value of a variable changes with the change in the value (or values) of the related variable (or variables). Suppose a train running at a uniform speed of v km./h. travels a distance of d km. in t hours. Obviously, if t remains unchanged then v increases or decreases according as d increases or decreases. But if d remains unchanged, then v decreases or increases according as t increases or decreases. This shows that the change in the value of a variable may be accompanied differently with the change in the values of related variables. Such relationship with regards to the change in the value of a variable when the values of the related variables change, is termed as variation.
We will discuss about such variations, which are classified into three types:
(1) Direct Variation
(2) Inverse Variation and
(3) Joint Variation.
“Variation” defines a concept that deals with variability in mathematics. Variation is defined by any change in some quantity due to change in another. We often come across with different types of variation problems in mathematics. Variation problems involve fairly simple relationships or formulas, involving one variable being equal to one term. Here follows the most common kinds of variation.
The constant of variation in a direct variation is the constant (unchanged) ratio of two variable quantities. In the following equation y varies directly with x, and k is called the constant of variation:
y = kx
Another form of variation is the inverse variation which works when there is a relationship between two variables in which the product is a constant. When one variable increases the other decreases in proportion so that the product is unchanged.
In problems relating to two or more variables, it is seen that the value of a variable changes with the change in the value (or values) of the related variable (or variables). Suppose a train running at a uniform speed of v km./h. travels a distance of d km. in t hours. Obviously, if t remains unchanged then v increases or decreases according as d increases or decreases. But if d remains unchanged, then v decreases or increases according as t increases or decreases. This shows that the change in the value of a variable may be accompanied differently with the change in the values of related variables. Such relationship with regards to the change in the value of a variable when the values of the related variables change, is termed as variation.
We will discuss about such variations, which are classified into three types:
(1) Direct Variation
(2) Inverse Variation and
(3) Joint Variation.
 0:10:21
0:10:21
 0:09:54
0:09:54
 0:05:30
0:05:30
 0:06:31
0:06:31
 0:07:05
0:07:05
 0:14:23
0:14:23
 0:03:57
0:03:57
 0:18:45
0:18:45
 0:12:23
0:12:23
 0:20:57
0:20:57
 0:10:32
0:10:32
 0:02:34
0:02:34
 0:04:18
0:04:18
 0:09:13
0:09:13
 0:08:27
0:08:27
 0:04:34
0:04:34
 0:27:16
0:27:16
 1:00:52
1:00:52
 0:14:59
0:14:59
 0:00:53
0:00:53
 0:40:15
0:40:15
 0:14:27
0:14:27
 0:06:56
0:06:56
 0:21:45
0:21:45