filmov
tv
4/m + m = 5, m =? Do you have the algebra skills to solve this equation? Let’s see…
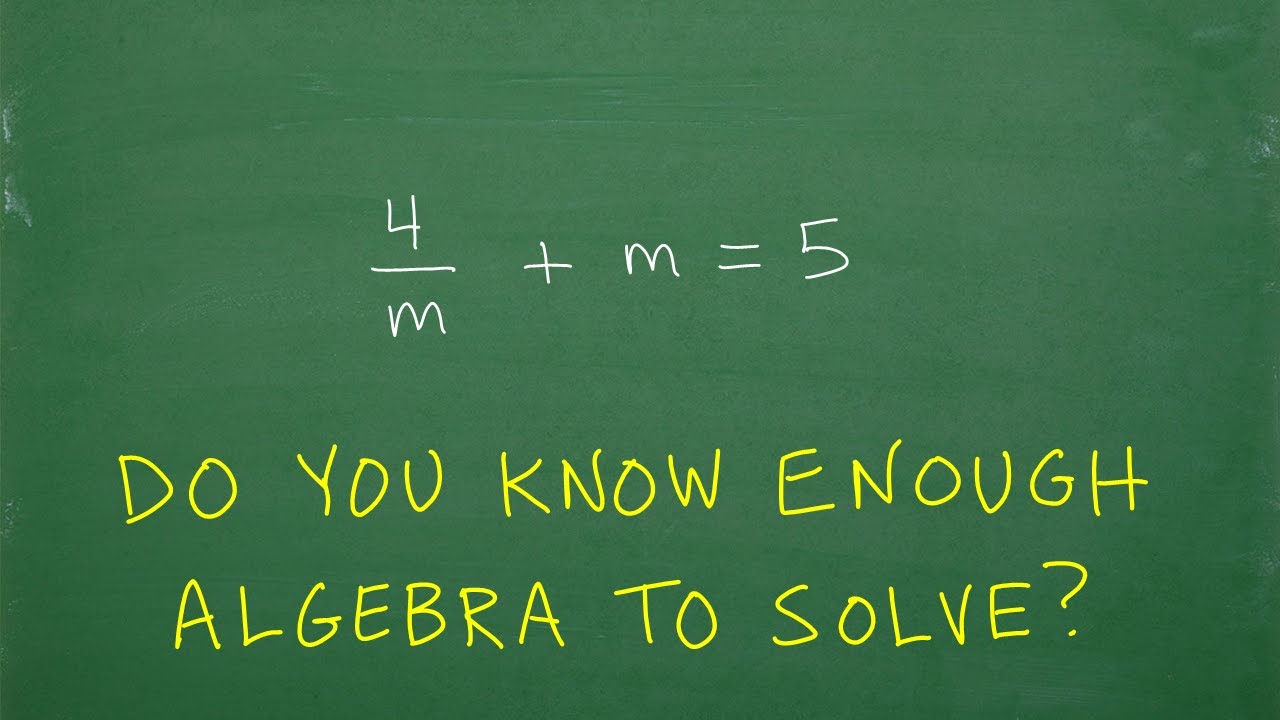
Показать описание
How to solve a rational equation.
For more in-depth math help check out my catalog of courses. Every course includes over 275 videos of easy to follow and understand math instruction, with fully explained practice problems and printable worksheets, review notes and quizzes. All courses developed and taught by me (experienced and certified math teacher 🤩).
With my courses you CAN reach your math goal! 💪
✓Help with Middle and High School Math (Public/Private Schools)
✓High School & College Math Test Prep
✓Teacher Certification Math Test Prep
✓Homeschool Math Program and Courses for Pre-Algebra, Algebra 1, Geometry, Algebra 2 and Pre-Calculus.
TC Math Notes
For more in-depth math help check out my catalog of courses. Every course includes over 275 videos of easy to follow and understand math instruction, with fully explained practice problems and printable worksheets, review notes and quizzes. All courses developed and taught by me (experienced and certified math teacher 🤩).
With my courses you CAN reach your math goal! 💪
✓Help with Middle and High School Math (Public/Private Schools)
✓High School & College Math Test Prep
✓Teacher Certification Math Test Prep
✓Homeschool Math Program and Courses for Pre-Algebra, Algebra 1, Geometry, Algebra 2 and Pre-Calculus.
TC Math Notes
Prym Ösen Ø 4, 5, 8 mm mit Scheiben | Vielseitige Ösen für Schnürverschlüsse, Deko & mehr
Auf 4 und 5 mm Dehnen
4/m + m = 5, m =? Do you have the algebra skills to solve this equation? Let’s see…
LISTY DO M. 5 | Oficjalny zwiastun | Kino Świat
Mega M - Žena 5 ft Tomáš Botló & Prince Marock |Official Video| prod.Drahoslav Bango X Prince Ma...
Nur 5,4m - Bester Grundriss Wohnmobil Kastenwagen 2023 Knaus BoxLife 540 MQ
So etwas gab es noch nie! RUNDSITZGRUPPE im 5,4m Kastenwagen Wohnmobil XL Bett Mega Mobil Lounge 540
Gleitbrett Vergleich 1,5 mm vs. 4 mm | Gleitbrett | Thermomix | KitchenAid ect.
Xcel Women's Axis Hooded 5/4mm Fullsuit Wetsuit | SwimOutlet.com
STEALTH WOHNMOBIL NUR 5,4m|DUSCHE|RUNDSITZGRUPPE|LÄNGSBETTEN| DEUTSCHER (58) baut GEILSTES der Welt!...
Plexiglas / Acrylglas bis 5 mm selber schneiden
'IF YOU YELL AT ME, I'M GONNA CRY!' 😭 | Season 5 Flashback | Dance Moms #Shorts
WOHNMOBIL SUPERLATIVE auf 5,4m KABE AFFINITY Freedom 540 2025 🌴 Highlights CARAVAN SALON 2024
Das Beste auf 5,41 m Länge? Dreamer D42 by Rapido, Bad hinten links, Längsbetten! Kann der was???
O'Neill Epic 5/4mm Wetsuit Review
Suzuki V Strom 250 seat height test. I'm 5 feet 9 inches tall and seat height is 835mm.
5,4m MINIWOHNMOBIL STEALTH CAMPER VAN Kabe Affinity Freedom 540 CMT Stuttgart 2024
Schutzgasschweißen Folge 8: 5 mm Kehlnaht steigend - Schweißen mit Christoph Staffel 2
WAHNSINNs WOHNMOBIL KASTENWAGEN NUR 5,4m Clever Tour 540 2024 mit 4! Schlafplätzen, Dusche Küche
😍 Nur 5,4m mit Dusche! 4 VOLLE Schlafplätze! Wohnmobil 2023 Knaus Boxstar 540 Road! Ab 57.820,- €!...
Pemasangan Kubah Masjid Model Pinang Diameter 5 m Di Kediri #shorts
5,4m! Kleinstes Monocock Wohnmobil der Welt: Wingamm 2022 Oasi 540 Full-GFK mit XXXL Hubbett. Wow!
IRRE DESIGN CAMPER 2024 echte 48.800,- € Euro Rapido Giottiline 5,4m Wohnmobil Kastenwagen
Wohnmobil 2023 NUR 5,4m! Rocket Camper One 540. Der beste Kastenwagen 2023. Gut,stabil,supergünstig!...
Комментарии
 0:02:00
0:02:00
 0:04:54
0:04:54
 0:22:29
0:22:29
 0:02:38
0:02:38
 0:04:17
0:04:17
 0:10:12
0:10:12
 0:08:33
0:08:33
 0:00:30
0:00:30
 0:00:06
0:00:06
 0:49:06
0:49:06
 0:01:58
0:01:58
 0:00:25
0:00:25
 0:15:31
0:15:31
 0:14:00
0:14:00
 0:02:56
0:02:56
 0:00:19
0:00:19
 0:31:17
0:31:17
 0:08:09
0:08:09
 0:25:30
0:25:30
 0:09:29
0:09:29
 0:00:11
0:00:11
 0:20:39
0:20:39
 0:18:06
0:18:06
 0:19:07
0:19:07