filmov
tv
A. Mironchenko. Semilinear boundary control systems: Well-posedness and stability.
Показать описание
Speaker:
Andrii Mironchenko (University of Passau)
Title:
Semilinear boundary control systems: Well-posedness and stability
Slides:
Abstract:
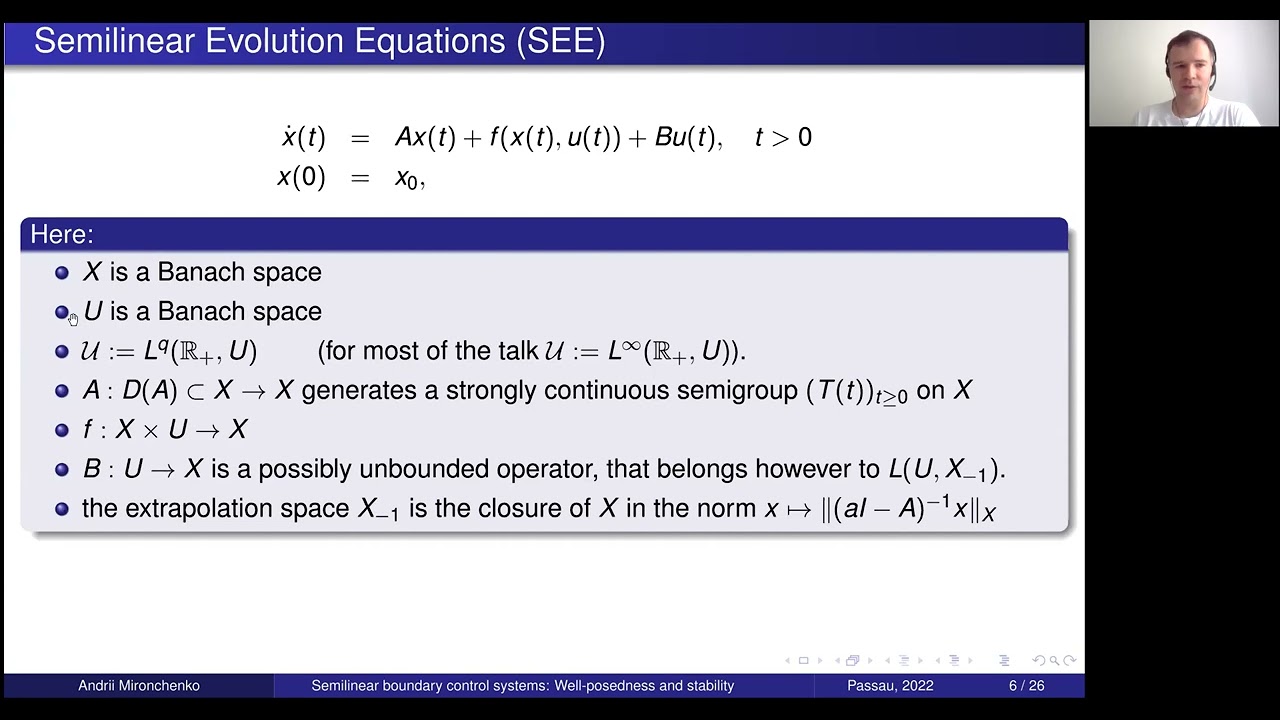
We consider a class of semilinear evolution equations in Banach spaces with unbounded input operators.
We provide sufficient conditions for the existence and uniqueness of mild solutions for this class of systems. Next, we study further properties, such as boundedness of reachability sets, continuous dependence on initial states and inputs, and Lipschitz regularity of the flow map.
Besides the fact that these properties are interesting per se, they are important for the analysis of the stability and robustness of such systems, including input-to-state stability, which we briefly discuss as well.
Having developed this theory, we show that a class of semilinear boundary control systems can be reformulated in terms of semilinear equations in Banach spaces. Thus, the above results can be used to analysis of boundary control systems as well.
Date:
Monday, 04 July 2022
Andrii Mironchenko (University of Passau)
Title:
Semilinear boundary control systems: Well-posedness and stability
Slides:
Abstract:
We consider a class of semilinear evolution equations in Banach spaces with unbounded input operators.
We provide sufficient conditions for the existence and uniqueness of mild solutions for this class of systems. Next, we study further properties, such as boundedness of reachability sets, continuous dependence on initial states and inputs, and Lipschitz regularity of the flow map.
Besides the fact that these properties are interesting per se, they are important for the analysis of the stability and robustness of such systems, including input-to-state stability, which we briefly discuss as well.
Having developed this theory, we show that a class of semilinear boundary control systems can be reformulated in terms of semilinear equations in Banach spaces. Thus, the above results can be used to analysis of boundary control systems as well.
Date:
Monday, 04 July 2022
0:44:26
A. Mironchenko. Semilinear boundary control systems: Well-posedness and stability.
0:58:11
Lauri Oksanen: On the boundary control method
0:16:42
A. Mironchenko. Lyapunov functions for ISS of infinite-dimensional systems with integrable inputs.
0:49:06
Input to state stability of distributed parameter systems. Habilitation Colloquium of Mironchenko A.
1:06:48
A. Mironchenko. Small-gain theory for infinite networks. Lyapunov functions meet Monotone systems.
0:00:33
What does semilinear mean?
0:39:47
A. Mironchenko. Robust forward completeness: a bridge between well-posedness and stability
0:00:45
Control of Haptic Systems Based on Input-to-State Stability
1:28:35
3rd ConFlex Meeting: Input-to-state stability by Dr. David Angeli - Part 1
0:39:10
Lecture 2.3: FOPDE's - General Solutions to Linear and Semilinear equations
0:45:42
M. Wakaiki. Semi-uniform ISS and iISS of infinite-dimensional systems
1:07:12
ICPAM-C9| LILIANE MAIA: Semilinear Parabolic Equations with asymptotically linear growth.
0:51:54
F. Schwenninger. BIBO stability and ISS for infinite-dimensional systems
0:42:03
A. Polyakov. On Input-to-State Stability of Homogeneous Evolution Equations.
0:55:20
CHRISTOPHE PRIEUR -SESSÃO 47 SEMINARIO DE EDP E MATEMATICA APLICADA
0:42:52
Ch. Guiver. The exponential ISS property — characterisations and feedback interconnections.
0:43:44
W. Perruquetti. Non-Asymptotic output feedback of a double integrator: a separation principle.
0:43:42
N. Espitia. Event-triggered boundary control of an unstable reaction diffusion PDE with input delay
0:58:58
Stability Analysis
0:55:31
J. L. Mancilla-Aguilar. From ISS to iISS without the use of Lyapunov functions
0:15:54
Opening
Комментарии