filmov
tv
Proof of the Sum-Difference Formulas - Part 1
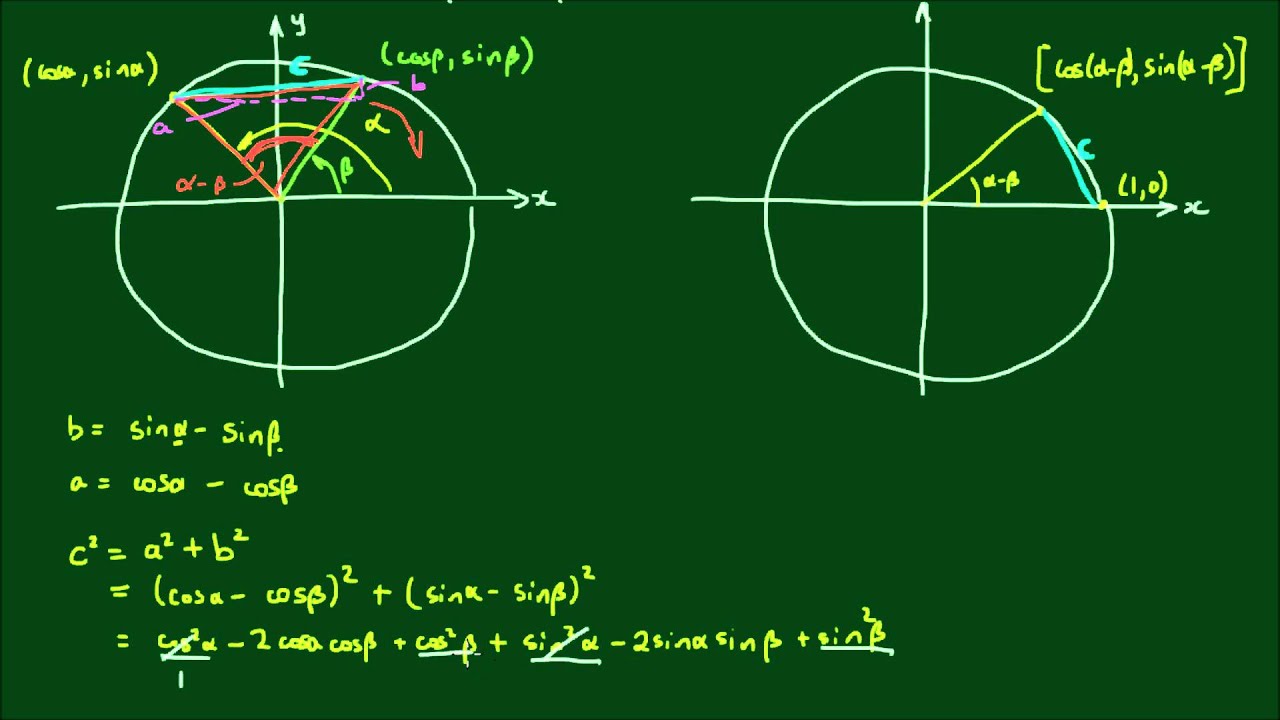
Показать описание
In this video, I demonstrate how to prove the following sum-difference formulas, or trigonometric identities:
cos(a - b) = cos(a)*cos(b) + sin(a)*sin(b)
cos(a + b) = cos(a)*cos(b) - sin(a)*sin(b)
sin(a + b) = sin(a)*cos(b) + sin(b)*cos(a)
sin(a - b) = sin(a)*cos(b) - sin(b)*cos(a)
From the results above, I also derive the following identities:
cos(2a) = cos^2(a) - sin^2(a)
sin(2a) = 2*sin(a)*cos(a)
Thanks for watching. Please give me a "thumbs up" if you have found this video helpful.
Please ask me a maths question by commenting below and I will try to help you in future videos.
cos(x - y) = cos(x)*cos(y) + sin(x)*sin(y)
cos(x + y) = cos(x)*cos(y) - sin(x)*sin(y)
sin(x + y) = sin(x)*cos(y) + sin(x)*cos(y)
sin(x - y) = sin(x)*cos(y) - sin(x)*cos(y)
cos(2x) = cos^2(x) - sin^2(x)
sin(2x) = 2*sin(x)*cos(x)
cos(a - b) = cos(a)*cos(b) + sin(a)*sin(b)
cos(a + b) = cos(a)*cos(b) - sin(a)*sin(b)
sin(a + b) = sin(a)*cos(b) + sin(b)*cos(a)
sin(a - b) = sin(a)*cos(b) - sin(b)*cos(a)
From the results above, I also derive the following identities:
cos(2a) = cos^2(a) - sin^2(a)
sin(2a) = 2*sin(a)*cos(a)
Thanks for watching. Please give me a "thumbs up" if you have found this video helpful.
Please ask me a maths question by commenting below and I will try to help you in future videos.
cos(x - y) = cos(x)*cos(y) + sin(x)*sin(y)
cos(x + y) = cos(x)*cos(y) - sin(x)*sin(y)
sin(x + y) = sin(x)*cos(y) + sin(x)*cos(y)
sin(x - y) = sin(x)*cos(y) - sin(x)*cos(y)
cos(2x) = cos^2(x) - sin^2(x)
sin(2x) = 2*sin(x)*cos(x)
Proof of the Sum-Difference Formulas - Part 1
Sine of a Sum I (visual proof; trigonometry)
Sum and Difference Formula Proofs
Proof of Sum and Difference Formulas for Sine, Cosine, and Tangent
Proof of angle addition formula for sine | Trigonometry | Khan Academy
Proof of the Sum-Difference Formulas - Part 2
Derivation of Sum and Difference Identities
Formulas for Trigonometric Functions: Sum/Difference, Double/Half-Angle, Prod-to-Sum/Sum-to-Prod
How to prove the sum-to-product identity for sine: sinA+sinB=2sin((A+B)/2)cos((A-B)/2)
Proof of the tangent angle sum and difference identities
Proof: Angle Sum/Difference Trig Identities (TAN)
The Sum and Difference Formulas - derivations (3 of 4: Trigonometric Identities) (A level)
Derive cosine angle sum & difference identities / addition & subtraction formulas
Factor a Sum of Two Cubes (visual proof without words)
Using Sum and Difference Formulas Involving Inverse Trigonometric Functions [7.5-4]
How to prove the cofunction identities using sum and difference formulas
Introduction to Sum and Difference Formulas in Trigonometry (Precalculus - Trigonometry 25)
TR-38: Angle Sum and Difference Identities (Trigonometry series by Dennis F. Davis)
Proof of the Sine, Cosine, and Tangent Sum and Difference Identities
Sine of a Sum II (visual proof, trigonometry)
P5.b (1/2) Proving Sum and Difference Formulas for Tangent
Geometric sum of powers of 7
Angle Sum Formula Proof with Linear Algebra!
Proof of Sum/Difference Rule [STEP BY STEP] | Calculus I
Комментарии
 0:17:25
0:17:25
 0:02:07
0:02:07
 0:20:57
0:20:57
 0:21:59
0:21:59
 0:08:26
0:08:26
 0:07:29
0:07:29
 0:14:58
0:14:58
 0:09:29
0:09:29
 0:04:59
0:04:59
 0:06:55
0:06:55
 0:04:02
0:04:02
 0:26:14
0:26:14
 0:01:00
0:01:00
 0:01:54
0:01:54
 0:15:47
0:15:47
 0:02:56
0:02:56
 0:51:27
0:51:27
 0:07:54
0:07:54
 0:12:30
0:12:30
 0:02:04
0:02:04
 0:04:16
0:04:16
 0:01:00
0:01:00
 0:01:59
0:01:59
 0:03:59
0:03:59